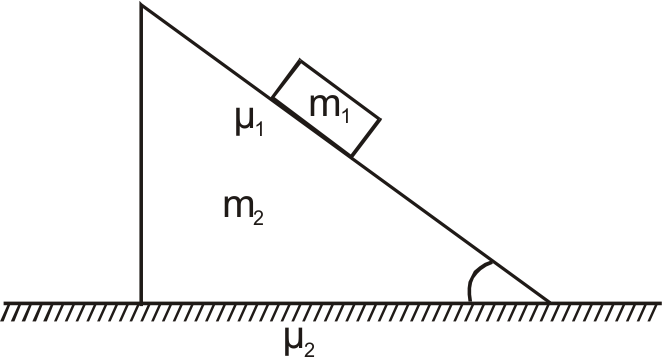
The block of mass m1 is placed on a wedge of an angle θ, as shown. The block is moving over the inclined surface of the wedge. Friction coefficient between the block and the wedge is μ1, whereas it is μ2 between the wedge and the horizontal surface. if μ1=12,θ=45∘,m1=4kg,m2=5kg and g=10m/s2, find minimum value of μ2 so that the wedge remains stationary on the surface. express your answer in multiple of 10−3
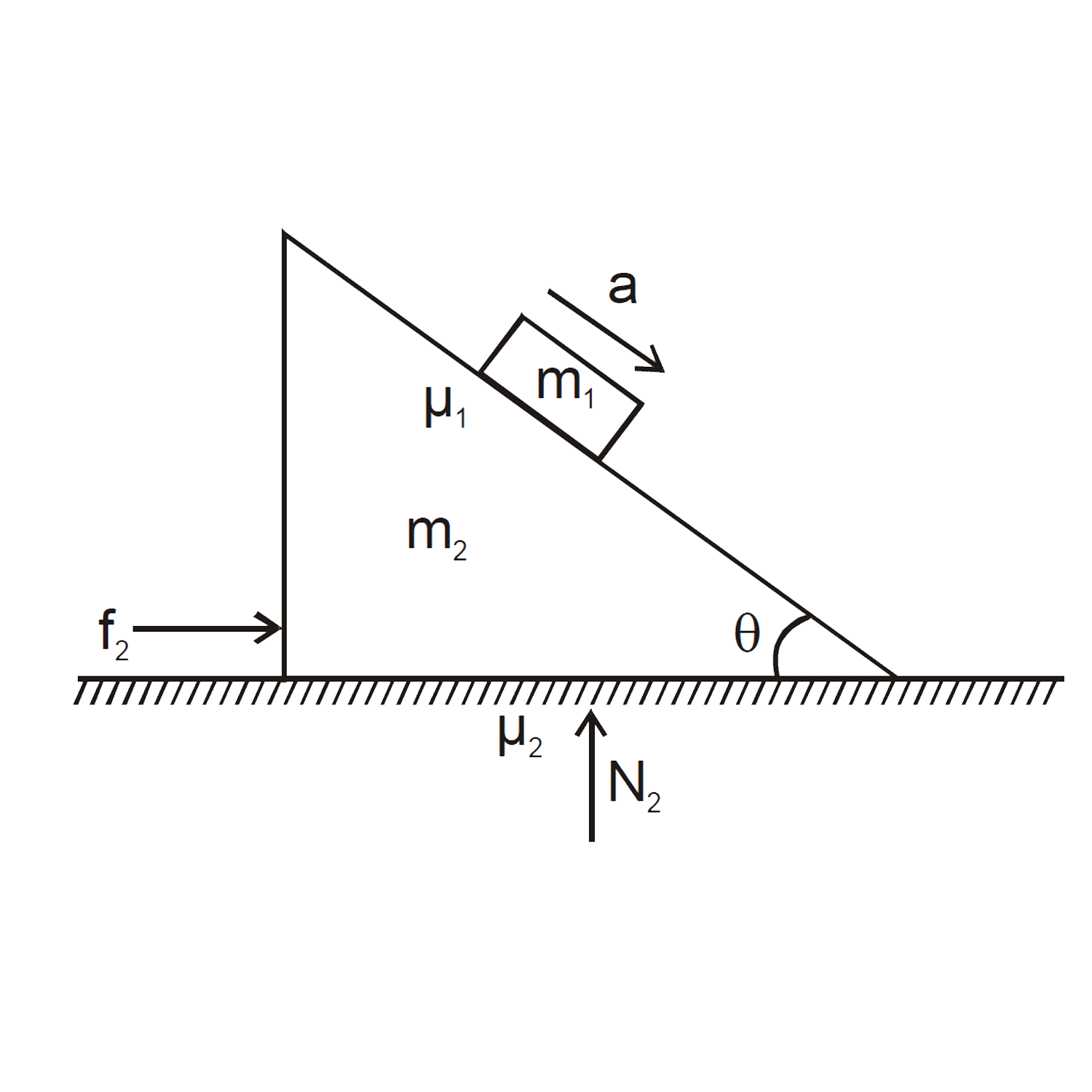
Taking block + wedge as system and applying NLM in horizontal direction
f2=m1acosθ ltbr> =m1[g(sinθ−μ,cosθ)]cosθ .... (1)
Again applying NLM in vertical direction
(m1+m2)g−N2=m1asinθ
N2=(m1+m2)g−m1sinθ(gsinθ−μ1gcosθ)
For limiting condition f2=μ2N2.....(2)
μ2=m1cosθ(gsinθ=μ1gcosθ)(m2+m2)g−m1sinθ(gsnθ−μ1gcosθ)
Using values
μ2=18=125×10−3
=125
Topper's Solved these Questions
Similar Questions
a Block of mass m is placed on a wedge of mass M there is no friction between the block and the wedge .The minmum coefficient of friction between the wedge and the gound so that wedge does not move is,
View SolutionA block of mass m is placed on a smooth wedge of mass M, as shown in the figure . Just after placiing block m over wedge M.
View SolutionA smooth block of mass m is held stationary on a smooth wedge of mass M and inclination θ as shown in figure .If the system is released from rest , then the normal reaction between the block and the wedge is

View SolutionA cube of mass m is placed on top of a wedge of mass 2m, as shown in figure. There is no friction between the cube and the wedge. The minimum co - efficient of friction between the wedge and the horizontal surface, so that the wedge does not move is
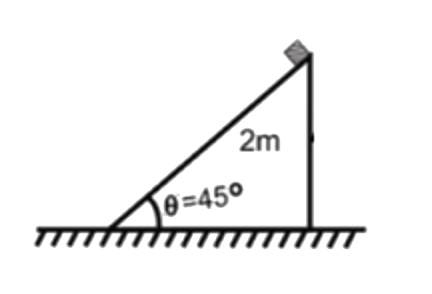
View SolutionIn the figure if block A and wedge b will move with same acceleration, then the magnitude of normal reaction between the block and the wedge will be (There is no friction between block and the wedge and the wedge moves on horizontal surface as shown.)
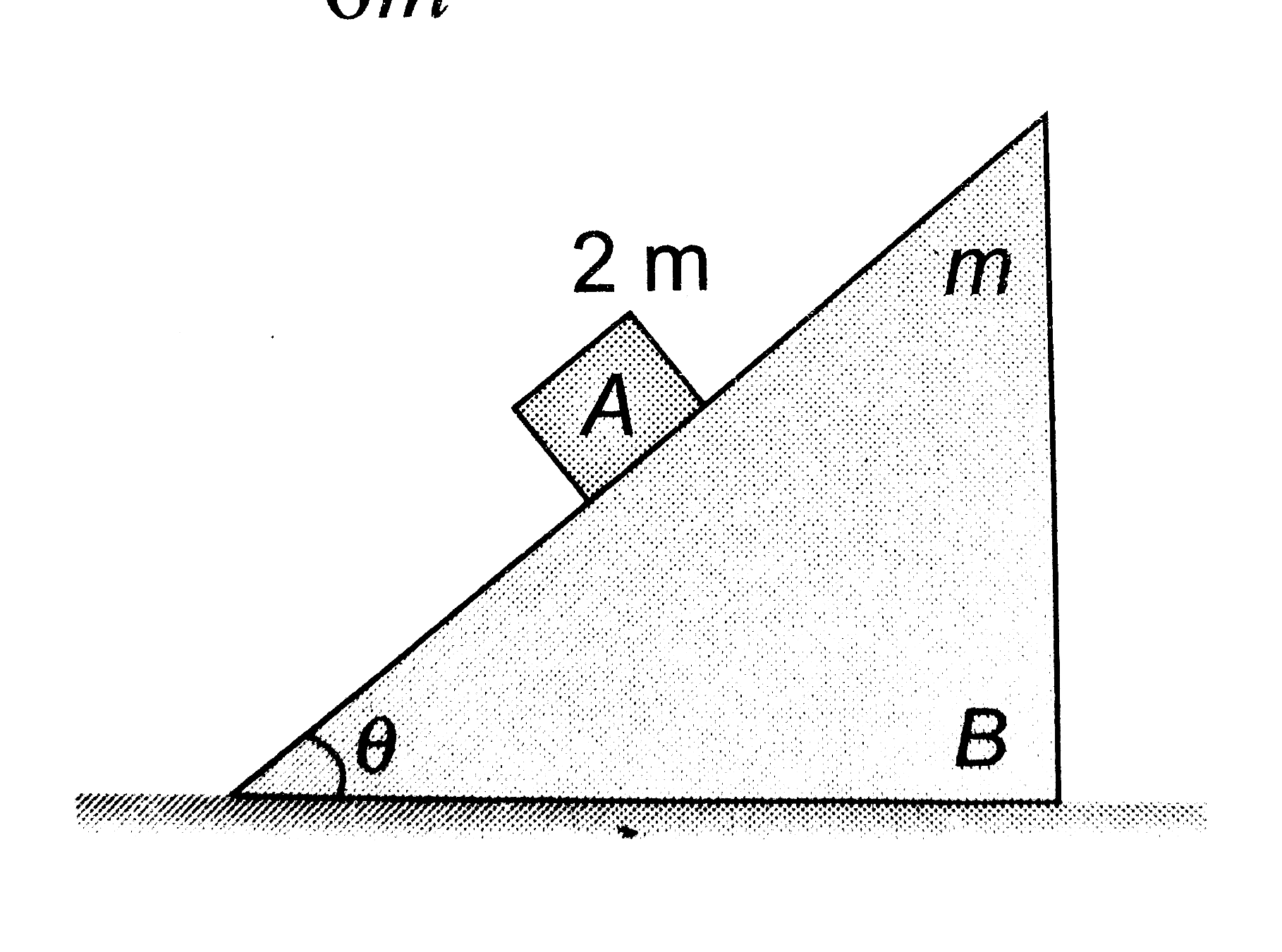
View SolutionIn the figure shown, if friction coefficient of block 1 kg and 2kg with inclined plane is μ1=0.5 and μ2=0.4 respectively, then
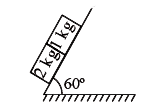
View SolutionTwo blocks of mass m and 2m are arranged on a wedge that is fixedd on a horizontal surface. Friction coefficient between the block and wedge are shown . Find the magnitude of acceleration of two blocks.

View SolutionAll surfaces are smooth in following figure. Find F, such that block remain stationary with respect to wedge.
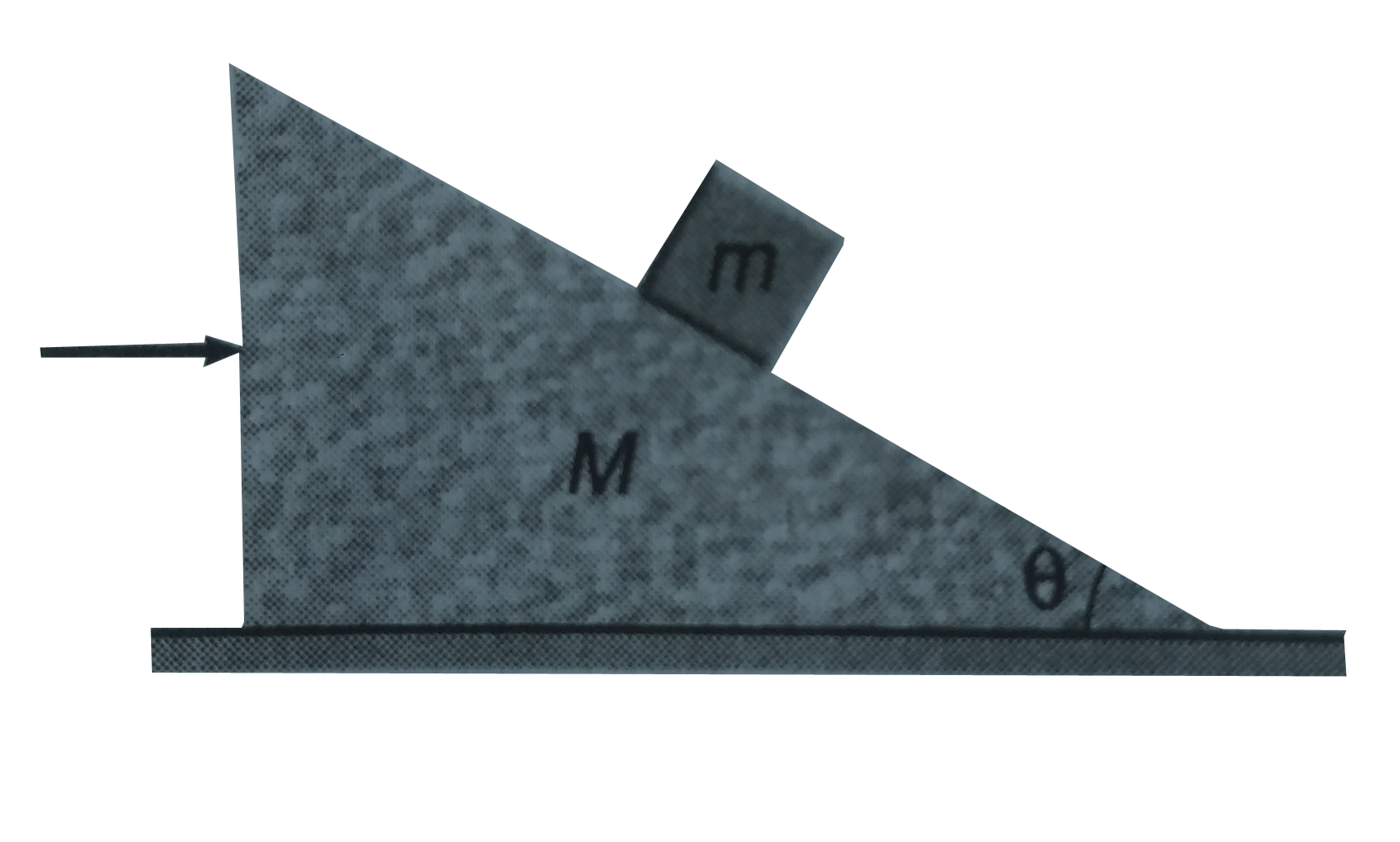
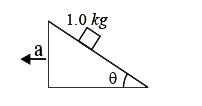
View SolutionA block of mass 1.0 kg rests on a wedge of angle θ. The acceleration which should be given to the wedge so that the block falls freely is :
View SolutionA block of mass m is placed on the inclined sufrace of a wedge as shown in fig. Calculate the acceleration of the wedge and the block when the block is released. Assume all surfaces are frictionless.

View Solution
