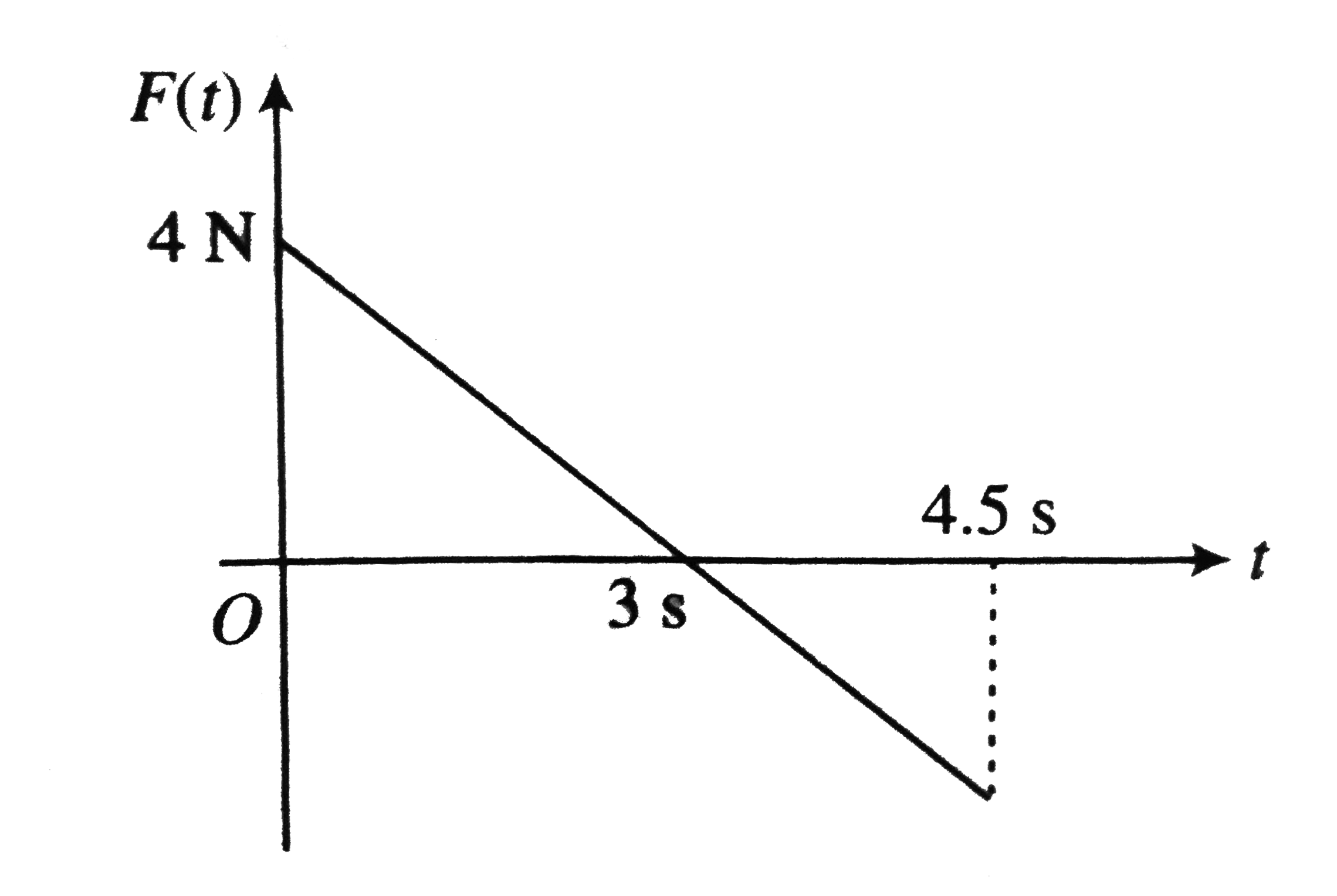
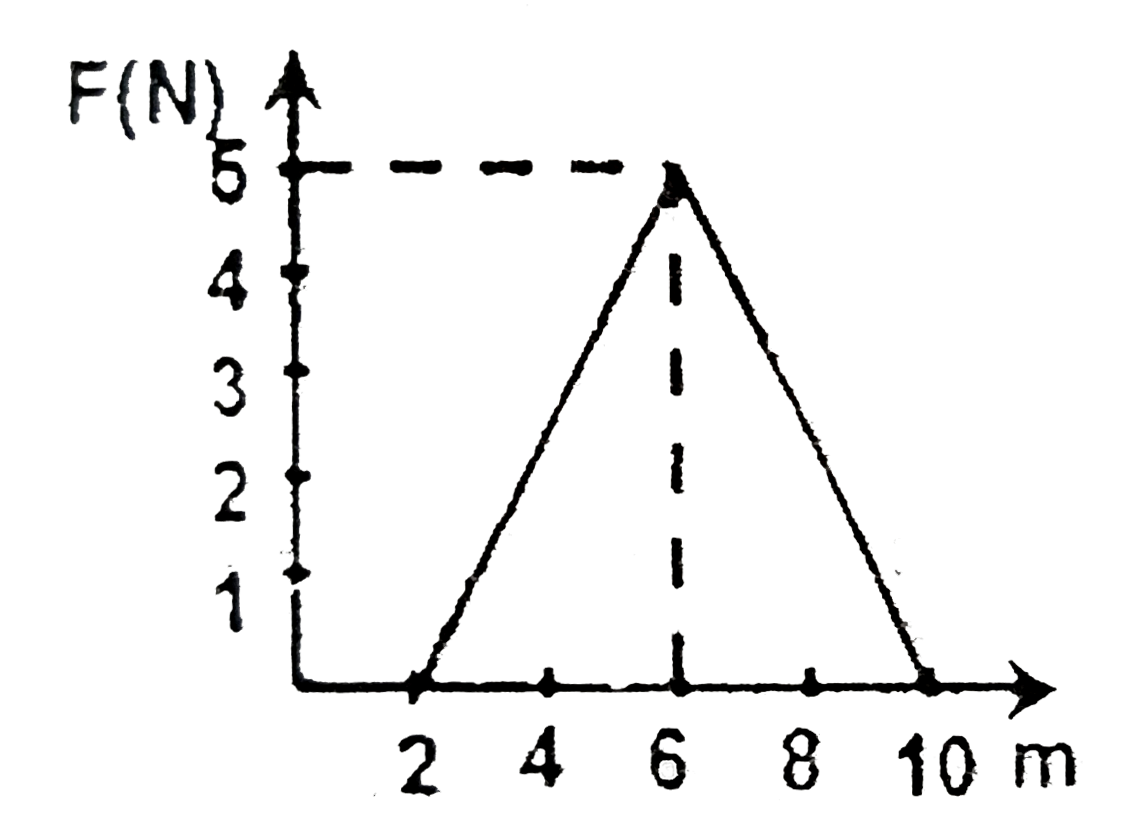
A block of mass 2kg is free to move along the x-axis. It is at rest and from t=0 onwards, it is subjected to a time-dependent force F(t) in the x-direction. The force F(t) vaies with t as shown in figure. The kinetic energy of the block after 4.5s is
3.50 J
7.50 J
5.06J
14.06J
The correct Answer is:C
The area under force time graph gives the change in momentum.
At t=0, block is at rest, so it means momentum at t=0 is zero. Let p is the momentum at t=4.5 s.
p−0=12×4×3−12×2×1.5=4.5N−s
KE at t=4.5s = p22m=(4.5)22×2 =5.0625J = 5.06J
Topper's Solved these Questions
Similar Questions
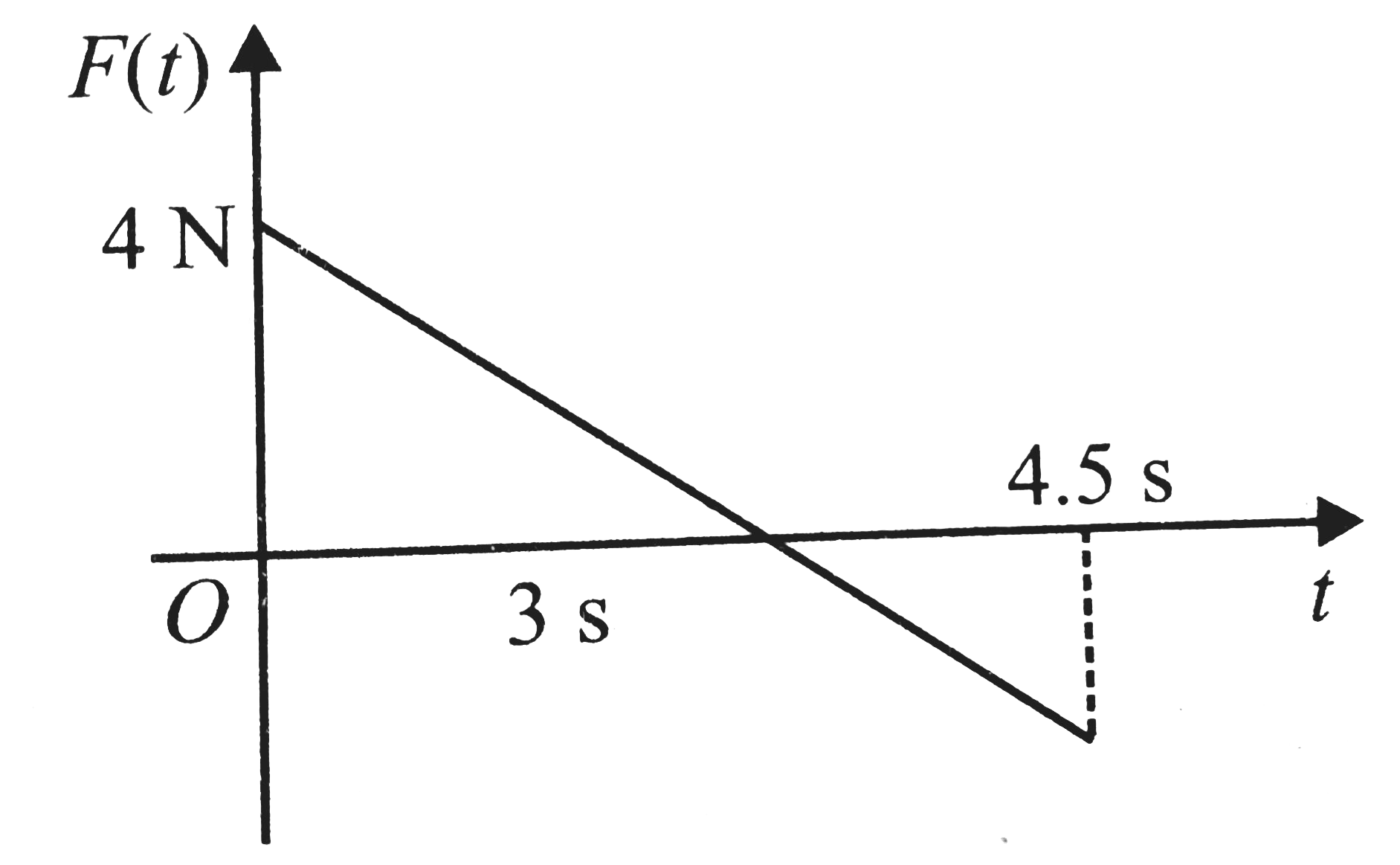
A block of mass 2kg is free to move along the x-axis. It at rest and from t=0onwards it is subjected to a time-dependent force F(t) in the x direction. The force F(t) varies with t as shown in the figure. The kinetic energy of the block after 4.5 seconds is
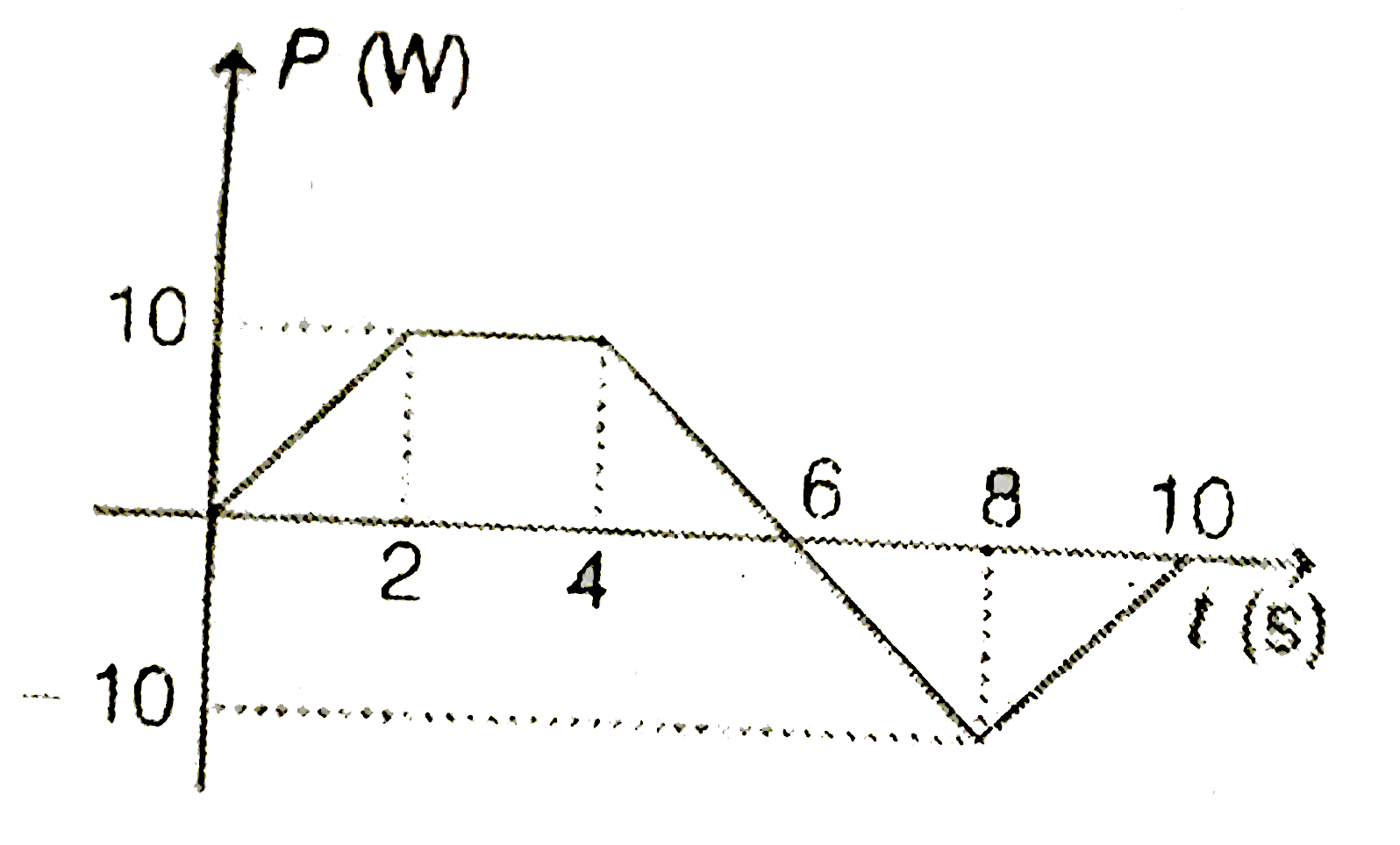
View SolutionPower of a force acting on a block varies with time t as shown in figure. Then, angle between force acting on the block and its velocity is
View SolutionA particle of mass m is at rest at the origin at time t=0 It is subjected to a force F(t)=F0e−bt in the X-direction. Its speed V(t) is depicted by which of the following curves
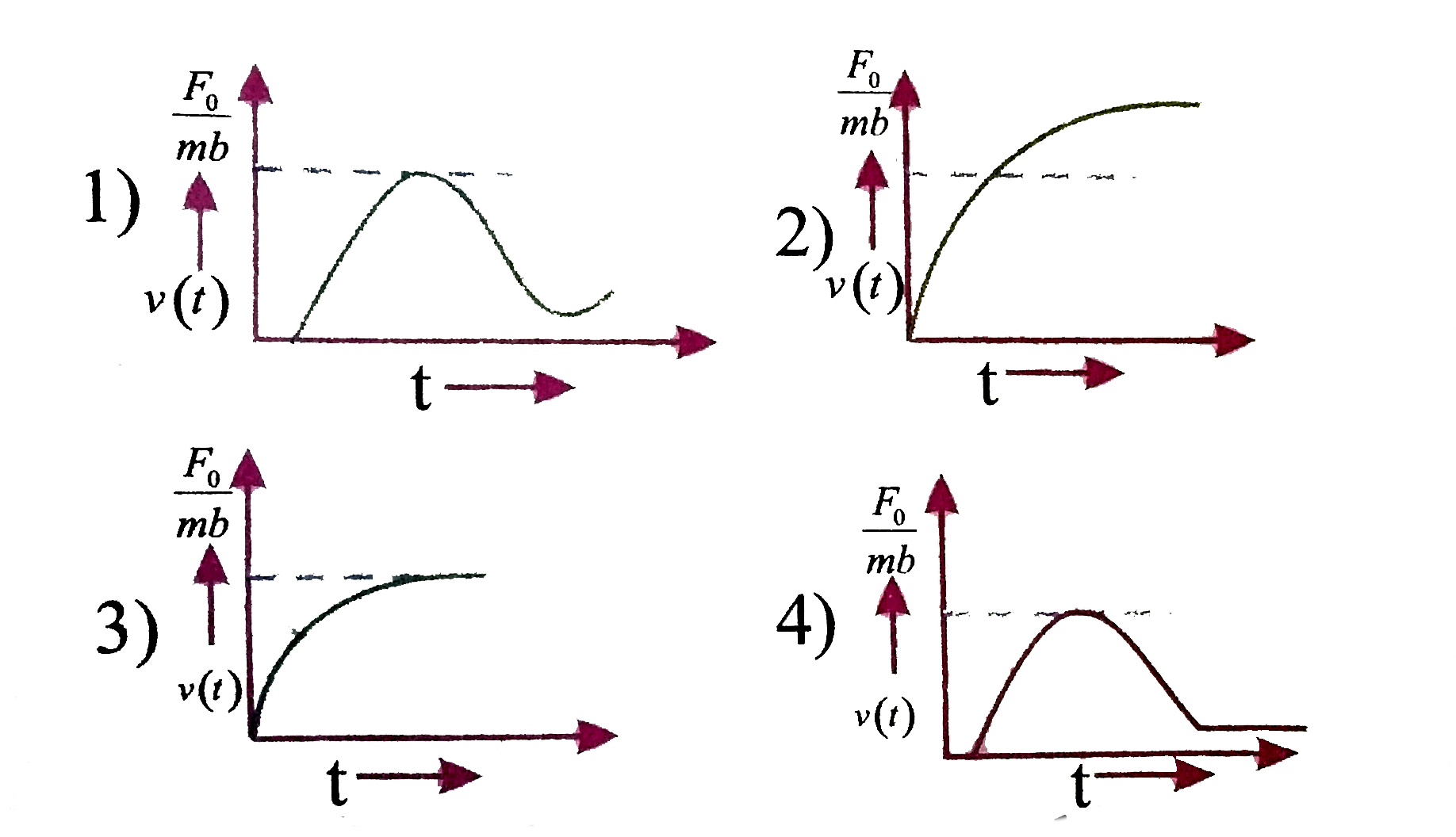
View SolutionA particle of mass m is at rest the origin at time t=0 . It is subjected to a force F(t)=F0e−bt in the x - direction. Its speed v(t) is depicted by which of the following curves ?
View SolutionA force shown in the F−x graph is applied to a 2kg block horizontal as shown in figure. The change in kinetic energy is
View SolutionThe momentum p of an object varies with time (t) as shown in figure. The corresponding force (F)- time (t) graph is -
View SolutionA block of mass m at rest is acted upon by a force F for a time t. The kinetic energy of block after time t is
View SolutionA sufficiently long plane of mass 4kg is placed on a smooth horizontal surfaces A small block of mass 2kg is placed over the plank and is being acted upon by a time varying horizontal force F=(0.5t) where F is in newton and t is in second as shown in figure. The coefficient of friction slipping the plank and the block is given is μs=μk=μ, at time t=12s the relative slipping between the plank and the block is just likely to occur

The coefficient of friction μ is equal to
View SolutionA sufficiently long plane of mass 4kg is placed on a smooth horizontal surfaces A small block of mass 2kg is placed over the plank and is beging acted upon by a time verying horizontal force F=(0.5t) where f is in newton and t is in second as shown in figure .The coefficient of friction slipping the plank and the block is given is μs=μk=mu time t=12s the relative slipping between the plank and the block is just likely to accor

The acceleration a versus time l graph for the and the block shown in figure below is correctely represented in
View SolutionA sufficiently long plane of mass 4kg is placed on a smooth horizontal surfaces A small block of mass 2kg is placed over the plank and is beging acted upon by a time verying horizontal force F=(0.5t) where f is in newton and t is in second as shown in figure .The coefficient of friction slipping the plank and the block is given is μs=μk=μ time t=12s the relative slipping between the plank and the block is just likely to accor

The average acceleration of the plank in the time interval 0→15s in the figure will be
View Solution