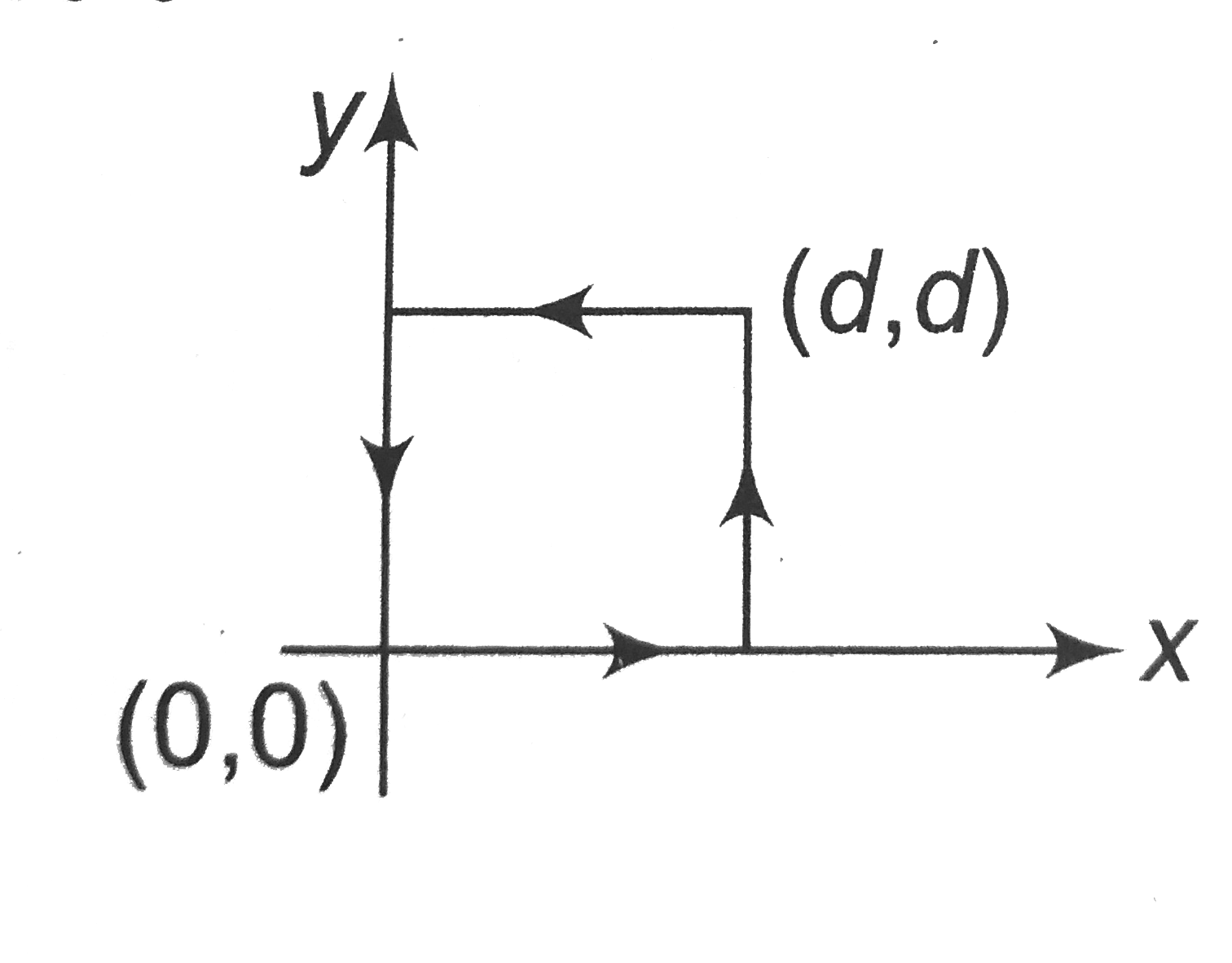
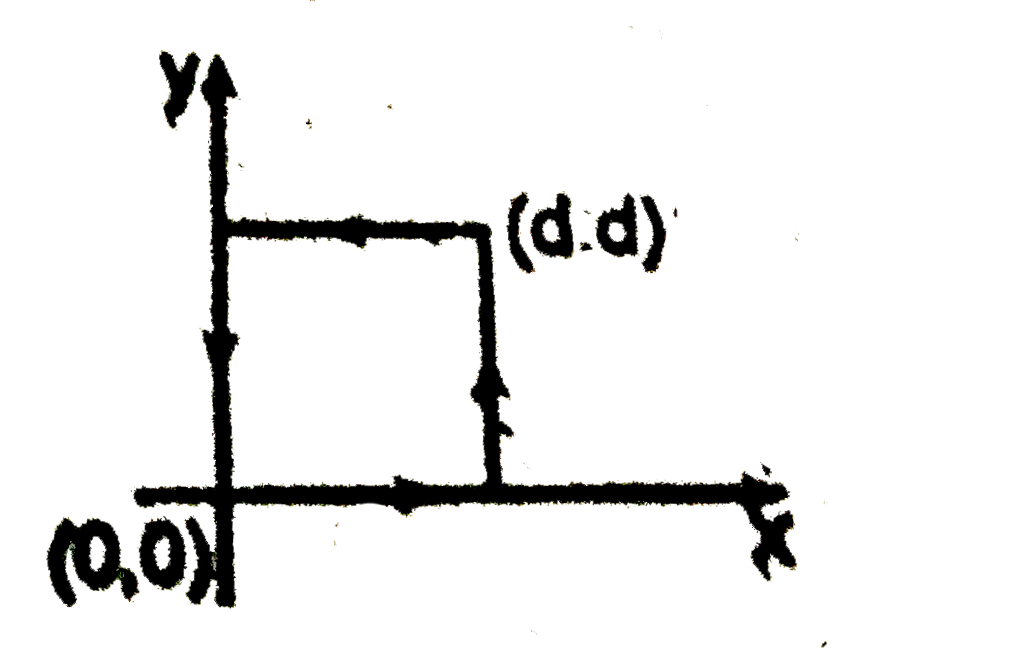
The work done by the force =→F=A(y2ˆi+2x2ˆj), where A is a constant and x and y are in meters around the path shown is.
zero
Ad
Ad2
Ad3
The correct Answer is:D
W=∫→F.−→dx=∫A(y2ˆi+2x2ˆj).(dxˆi+dy.ˆj)
=A∫(y2dx+2x2dy)
WOA=0+0,WAB−A[0+2d2d]
WBC=A[d2(−d)+0],WCD=A[0+0]
W=0+2ad2−Ad3+0=Ad3
Topper's Solved these Questions
Similar Questions
The work done by the force →F=A(y2ˆi+2x2ˆj), where A is a constant and x & y are in meters around the path shown is :
View SolutionThe work done by the forces →F=2ˆi−ˆj−ˆk in moving an object along the vectors 3ˆi+2ˆj−5ˆk is:
View SolutionA particle is moved along a path AB−BC−CD−DE−EF−FA, as shown in figure, in presence of a force →F=(αyˆi+2αxˆj)N, where x and y are in meter and α=−1Nm−1. The work done on the particle by this by this force will be Joule.

View SolutionThe intensity of an electric field depends only on the coordinates x and y as follows,
E=a(xˆi+yˆj)x2+y2 where, a is constant and ˆi and ˆj are the unit vectors of the x and y axes. Find the charges within a sphere of radius R with the centre at the origin.
View SolutionA force F=−k(^ˆi+xˆj) (where k is a positive constant) acts on a particle moving in the x−y plane. Starting from the origin, the particle is taken along the positive x-axis to the point (a,0) and then parallel to the y-axis to the point (a,a). The total work done by the force F on the particle is
(a) −2ka2 , (b) 2ka2,(c)-ka^(2),(d)ka^(2)
View SolutionThe work done by an applied variable force F=x+x3 from x = 0 m to x = 2m, where x is displacement, is
View SolutionA particle of mass m initially at rest. A variabl force acts on the particle f=kx2 where k is a constant and x is the displacment. Find the work done by the force f when the speed of particles is v.
View SolutionA force →F=(−yˆi+xˆj)N acts on a particle as it moves in an anticlockwise circular motion in x-y plane. The centre of the circle is at the origin. If the work done by the force is 32πJ in one complete revolution then asSigmaing x,y to be in meters, find the radius of the path.
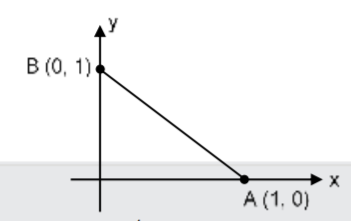
View SolutionParticle moves from point A to point B along the line shown in figure under the action of force. evcF=−xˆi+yˆj. Determine the work done on the particle by →F in moving the particle from point A to point B.
View SolutionThe work done by a force →F=(−6x3ˆi) N in displacing a particle from x = 4m to x = - 2m is
View Solution
