Three particles are projected upwards with initial speeds 10m/s,20m/s,30m/s. The displacements covered by them in their last second of motion are x1,x2,x3 then:
x1:x2:x3=1:2:3
x1:x2:x3=1:4:9
x1:x2:x3=1:5:7
none of these
The correct Answer is:D
To solve the problem, we need to find the displacements x1,x2,x3 covered by three particles projected upwards with initial speeds u1=10m/s, u2=20m/s, and u3=30m/s during their last second of motion.
Step 1: Understand the motion
When a particle is projected upwards, it will eventually stop momentarily at its highest point before falling back down. The displacement covered in the last second of motion can be calculated using the equations of motion.
Step 2: Use the equation of motion
The displacement s during the last second of motion can be calculated using the formula:
s=u⋅t−12gt2
where:
- u is the initial velocity,
- g is the acceleration due to gravity (approximately 10m/s2),
- t is the time interval (which is 1s for the last second).
Step 3: Calculate x1 for the first particle
For the first particle with u1=10m/s:
x1=u1⋅1−12g⋅(1)2
x1=10⋅1−12⋅10⋅12
x1=10−5=5m
Step 4: Calculate x2 for the second particle
For the second particle with u2=20m/s:
x2=u2⋅1−12g⋅(1)2
x2=20⋅1−12⋅10⋅12
x2=20−5=15m
Step 5: Calculate x3 for the third particle
For the third particle with u3=30m/s:
x3=u3⋅1−12g⋅(1)2
x3=30⋅1−12⋅10⋅12
x3=30−5=25m
Step 6: Find the ratio x1:x2:x3
Now we have:
- x1=5m
- x2=15m
- x3=25m
The ratio x1:x2:x3 is:
x1:x2:x3=5:15:25
Dividing each term by 5 gives:
1:3:5
Final Answer
The ratio of displacements covered by the three particles in their last second of motion is:
1:3:5
When a particle is projected upwards, it will eventually stop momentarily at its highest point before falling back down. The displacement covered in the last second of motion can be calculated using the equations of motion.
The displacement s during the last second of motion can be calculated using the formula:
s=u⋅t−12gt2
where:
- u is the initial velocity,
- g is the acceleration due to gravity (approximately 10m/s2),
- t is the time interval (which is 1s for the last second).
For the first particle with u1=10m/s:
x1=u1⋅1−12g⋅(1)2
x1=10⋅1−12⋅10⋅12
x1=10−5=5m
For the second particle with u2=20m/s:
x2=u2⋅1−12g⋅(1)2
x2=20⋅1−12⋅10⋅12
x2=20−5=15m
For the third particle with u3=30m/s:
x3=u3⋅1−12g⋅(1)2
x3=30⋅1−12⋅10⋅12
x3=30−5=25m
Now we have:
- x1=5m
- x2=15m
- x3=25m
x1:x2:x3=5:15:25
Dividing each term by 5 gives:
1:3:5
The ratio of displacements covered by the three particles in their last second of motion is:
1:3:5
Topper's Solved these Questions
Similar Questions
A particle is projected vertically upwards with an initial velocity of 40m/s. Find the displacement and distance covered by the particle in 6s. Take g=10m/s2.
View SolutionTwo particles are projected with speed 4m/s and 3m/s simultaneously from same point as shown in the figure. Then:

View SolutionA body is projected with an initial elocity 20m/s at 60° to the horizontal. The displacement after 2 s is
View SolutionA ball is projected vertically up with speed 20 m/s. Take g=10m/s2
View SolutionFrom the top of a tower, a particle is thrown vertically downwards with a velocity of 10m/s. The ratio of the distances, covered by it in the 3rd and 2nd seconds of the motion is (Takeg=10m/s2).
View SolutionA particle is projected vertically upwards with velocity 40m/s. Find the displacement and distance travelled by the particle in
(a) 2s (b) 4s (c) 6s Take g=10m/s2
View SolutionA body is projected vertically upward with speed 40m/s. The distance travelled by body in the last second of upward journey is [take g=9.8ms2 and neglect of air resistance]:-
View SolutionA particle has an initial velocity of 9m/s due east and a constant acceleration of 2m/s2 due west. The distance coverd by the particle in the fifth second of its motion is :
View SolutionA particle A is projected with an initial velocity of 60m/s at an angle 30∘ to the horizontal. At the same time a second particle B is projected in opposite direction with initial speed of 50m/s from a point at a distance of 100 m from A. If the particles collide in air, find (a)the angle of projection α of particle B, (b) time when the collision takes place and (c) the distance of P from A, where collision occurs. (g=10m/s2)
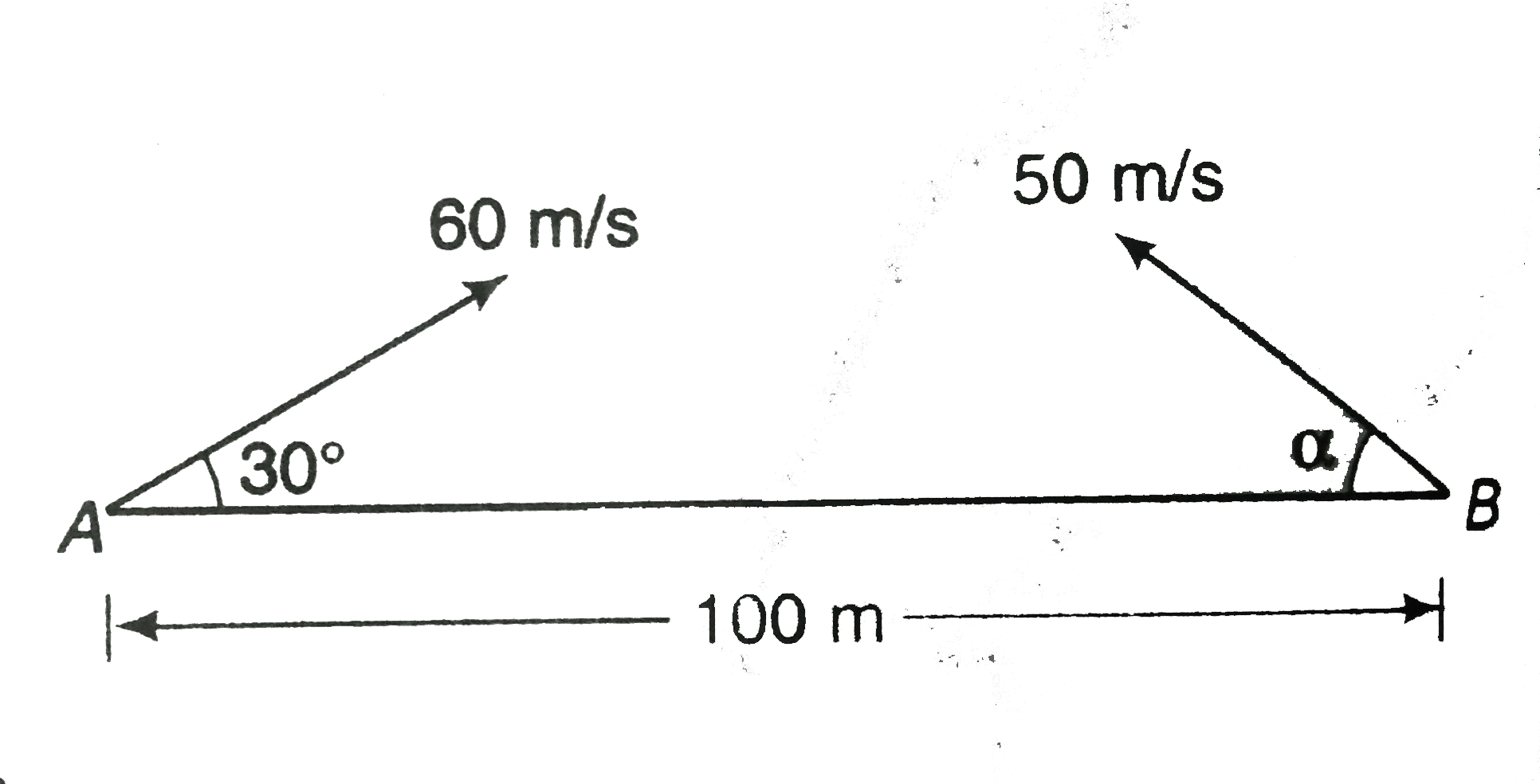
View SolutionTwo particles of equal mass are projected simultaneously with speeds 20m/s end 10√3m/s as shown in figure. Find the maximum height reached by the centre of mass of the particles
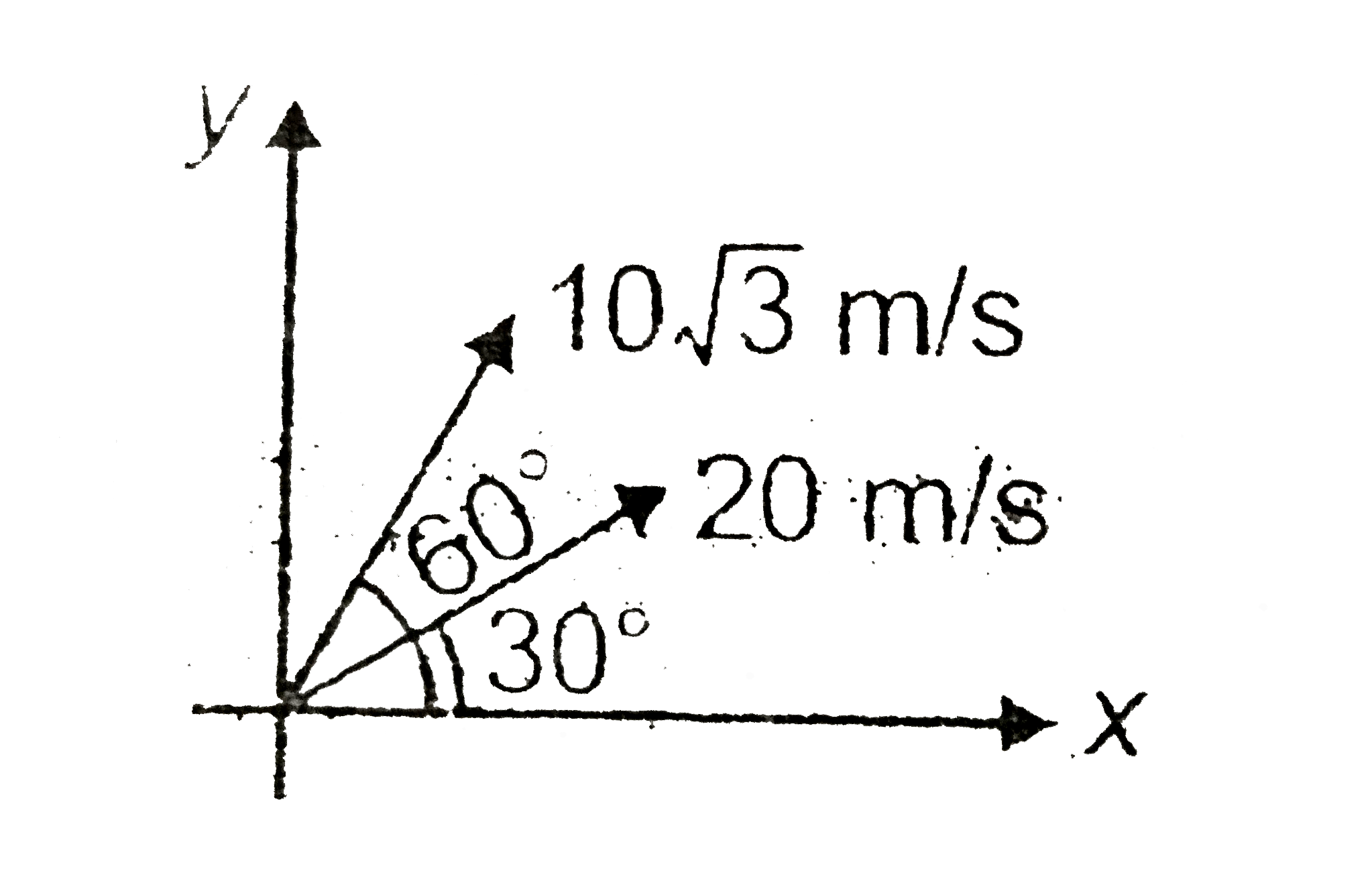
View Solution
