A particle is projected with speed from a point making an angle with the horizontal. At the same instant, a second particle is thrown vertically upwards from a point directly below the maximum height point of parabolic path of A, with velocity . If the two particles collide then the ratio of should be:
The correct Answer is:B
To solve the problem, we need to analyze the motion of both particles A and B and find the ratio of their velocities when they collide.
1. Understanding the Motion of Particle A:
- Particle A is projected with speed VA at an angle of 60∘ with the horizontal.
- The vertical component of the velocity of A can be calculated as:
VAy=VAsin(60∘)=VA⋅√32
2. Time Taken by Particle A to Reach Maximum Height:
- The time taken by particle A to reach its maximum height can be calculated using the formula:
tA=VAyg=VA⋅√32g
- Simplifying this gives:
tA=VA√32g
3. Understanding the Motion of Particle B:
- Particle B is thrown vertically upwards from a point directly below the maximum height of A with velocity VB.
- The time taken by particle B to reach its maximum height is given by:
tB=VBg
4. Setting the Times Equal:
- Since both particles collide at the same height at the same time, we set tA equal to tB:
VA√32g=VBg
- We can cancel g from both sides:
VA√32=VB
5. Finding the Ratio VAVB:
- Rearranging the equation gives:
VA=2VB√3
- Therefore, the ratio VAVB is:
VAVB=2√3
Final Answer:
The ratio of VA to VB is:
VAVB=2√3
- Particle A is projected with speed VA at an angle of 60∘ with the horizontal.
- The vertical component of the velocity of A can be calculated as:
VAy=VAsin(60∘)=VA⋅√32
- The time taken by particle A to reach its maximum height can be calculated using the formula:
tA=VAyg=VA⋅√32g
- Simplifying this gives:
tA=VA√32g
- Particle B is thrown vertically upwards from a point directly below the maximum height of A with velocity VB.
- The time taken by particle B to reach its maximum height is given by:
tB=VBg
- Since both particles collide at the same height at the same time, we set tA equal to tB:
VA√32g=VBg
- We can cancel g from both sides:
VA√32=VB
- Rearranging the equation gives:
VA=2VB√3
- Therefore, the ratio VAVB is:
VAVB=2√3
The ratio of VA to VB is:
VAVB=2√3
Topper's Solved these Questions
Similar Questions
A particle A is projected with speed VA from a point making an angle 60∘ with the horizontal. At the same instant, second particle B( lie in the same horizontal plane ) is thrown vertically upwards from a point directly below the maximum height point of parabolic path of A, with velocity VB. If the two particles collide then the ratio of VA/VB should be :
View SolutionA particle A is projected with speed VA from a point making an angle 60∘ with the horizontal. At the same instant, second particle B( lie in the same horizontal plane ) is thrown vertically upwards from a point directly below the maximum height point of parabolic path of A, with velocity VB. If the two particles collide then the ratio of VA/VB should be :
View SolutionA particle P is projected with velocity u1 at an angle of 30∘ with the horizontal. Another particle Q is thrown vertically upwards with velocity u2 from a point vertically below the highest point of path of P. Determine the necessary condition for the two particles to collide at the highest point.
 .
.
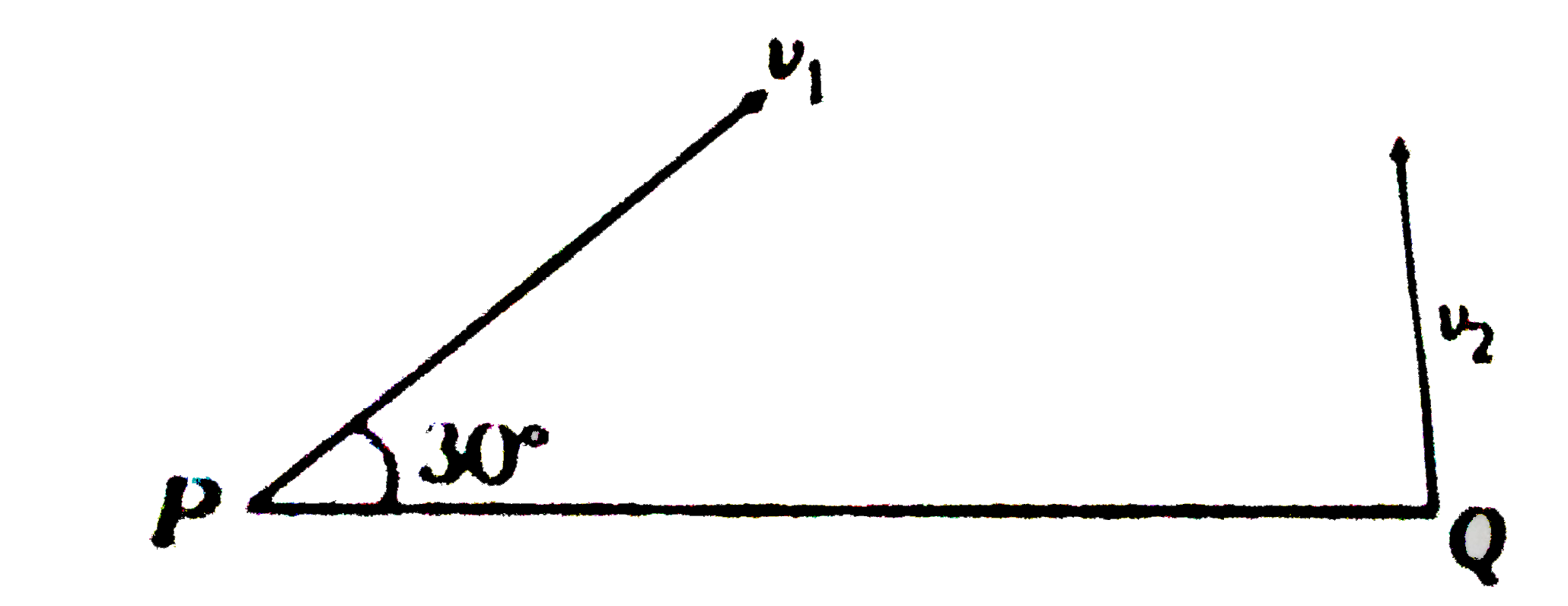
View SolutionA projectile A is thrown at an angle 30∘ to the horizontal from point P. At the same time, another projectile B is thrown with velocity v2 upwards from the point Q vertically below the highest point A would reach. For B to collide with A the ratio v2v1 should be
View SolutionA particle is projected vertically upward with velocity u from a point A, when it returns to the point of projection .
View SolutionA particle of mass m is projected with a velocity v making an angle of 45∘ with the horizontal. The magnitude of the angular momentum of the projectile abut the point of projection when the particle is at its maximum height h is.
View SolutionA particle is projected with a velocity u making an angle θ with the horizontal. At any instant its velocity becomes v which is perpendicular to the initial velocity u. Then v is
View SolutionTwo projectiles thrown from the same point at angles 60∘and30∘ with the horizontal attain the same height. The ratio of their initial velocities is
View SolutionA particle A is projected with an initial velocity of 60m/s at an angle 30∘ to the horizontal. At the same time a second particle B is projected in opposite direction with initial speed of 50m/s from a point at a distance of 100 m from A. If the particles collide in air, find (a)the angle of projection α of particle B, (b) time when the collision takes place and (c) the distance of P from A, where collision occurs. (g=10m/s2)
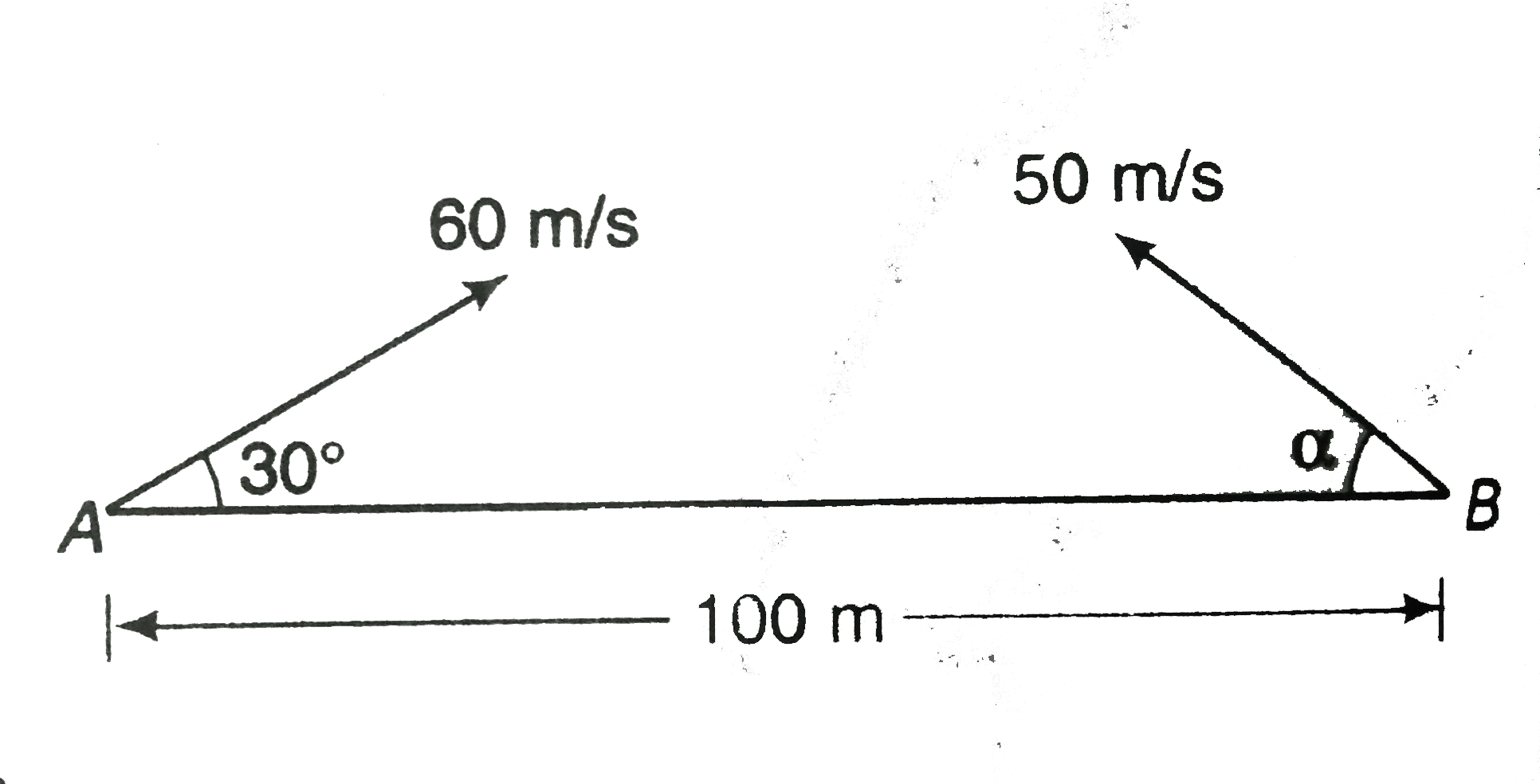
View SolutionA particle is projected with velocity v at an angle θ aith horizontal. The average angle velocity of the particle from the point of projection to impact equals
View Solution