Two small electric dipoles each of dipole moment pˆi are situated at (0,0,0) and (r,0,0). The electric potential at a point (r2,√3r2,0) is:
p4π∈0r2
0
p2π∈0r2
p8π∈0r2
The correct Answer is:B
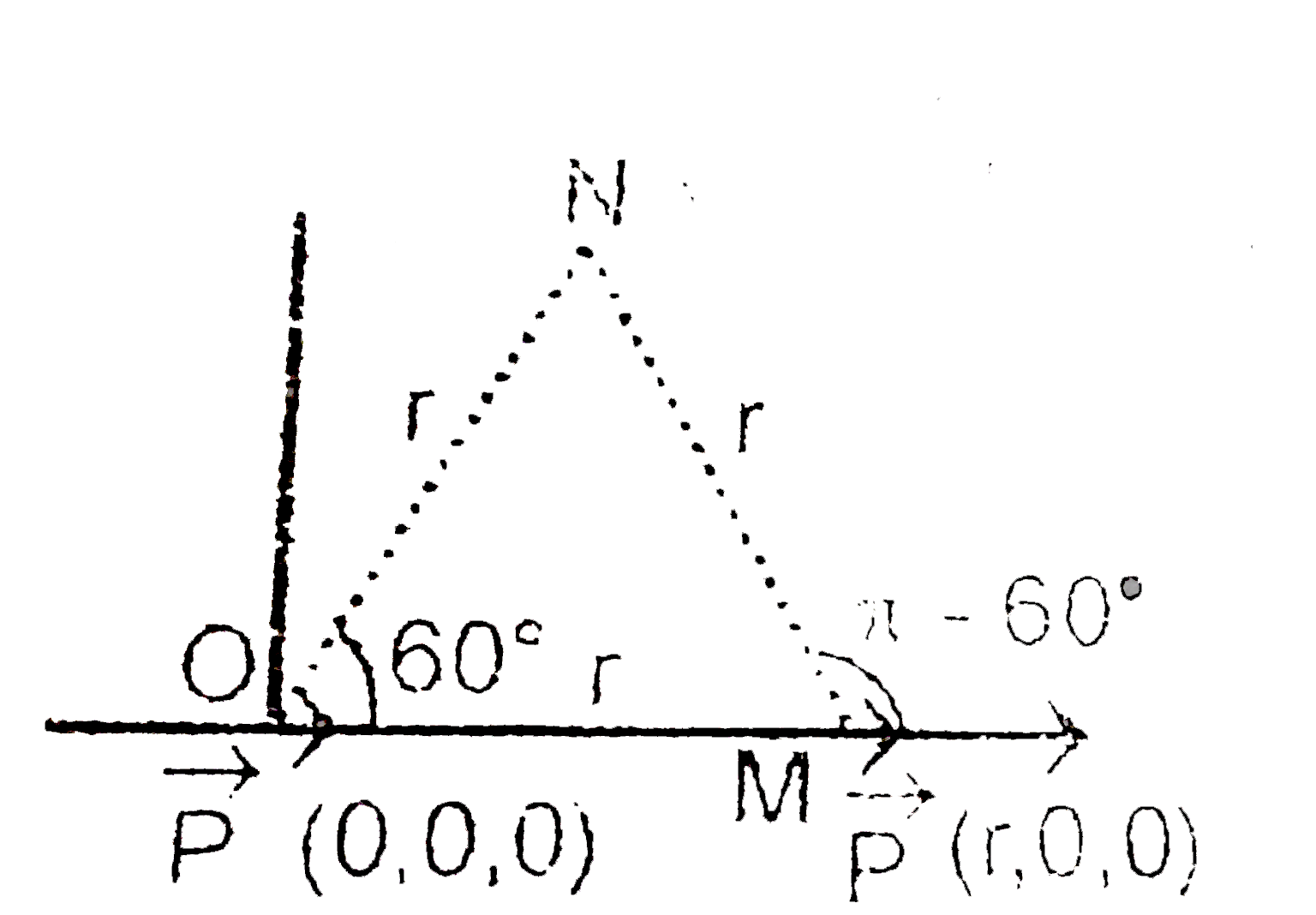
As ON=MN=OM=r
So its equilateral triangle
∴ Potential at N due to two dipoles ,
V=V1+V2=Kpcos60∘r2+Kpcos(π−60∘)r2=0
Related Playlists
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.329videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.3320videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.309videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)19videos
CURRENT ELECTRICITY
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 327videos
ELECTRO MAGNETIC WAVES
Similar Questions
A small electric dipole is placed at origin with its dipole moment directed along positive x-axis .The direction of electric field at point (2,2√2,0)
View SolutionTwo point charges −q and +q are located at points (0,0−a) and (0,0,a) respectively. The electric potential at point (0,0,z)is (Z>a)
View SolutionA short electric dipole having dipole moment ¯ρ is placed along x-axis as shown in figure. The electric potential at point A(-2, 3) is (k=14πε0 and all quantities are in S. I. units)
View SolutionA dipole of dipole moment →P=2ˆi−3ˆj+4ˆk is placed at point A (2, -3, 1). The electric potential due to this dipole at the point B (4, -1, 0) is equal to (All the parameters specified here are in S.L. units) ____×109 volts
View SolutionA tiny electric dipole of dipole moment →P=P0ˆj is placed at point (l,0). There exists an electric field →E=2ax2ˆi+(2by2+2cy)ˆj.
View SolutionTwo short dipoles pˆk and P2ˆk are located at (0,0,0) & (1m,0,2m) respectivley. The resultant electric field due to the two dipoles at the point (1m,0,0) is
View SolutionTwo short dipoles pˆk and P2ˆk are located at (0,0,0) & (1m,0,2m) respectivley. The resultant electric field due to the two dipoles at the point (1m,0,0) is
View SolutionTwo small particles P and Q each of mass m are fixed along x-axis at points (a,0) and (-a,0). A third particle R is kept at origin. Then
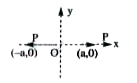
View SolutionA system of two electric dipoles, each of dipole moment having magnitude P are arranged in the configuration shown in figure The electrostatic interaction energy of this systm of two dipoles is :
 View Solution
View SolutionTwo short dipoles, each of diple moment p , are placed at origin. The dipole moment of one dipole is along x-axis, while that of other is along y-axis . The electric field at a point (a,0) is given by
View Solution


