Sand is droped vetically downward at the constant rate on a conveyor belt which is moving horizontally with velocity . Assume that sant particles comes to rest with respect to belt immediately after landing.
The rate which work is done by friction force on sand is
To solve the problem, we need to determine the rate at which work is done by the friction force on the sand as it lands on the conveyor belt. Here’s the step-by-step solution:
Step 1: Understand the system
- Sand is dropped vertically at a constant rate of μkgs onto a conveyor belt moving horizontally with a velocity v.
- When the sand particles land on the belt, they come to rest relative to the belt immediately.
Step 2: Determine the mass on the conveyor belt
- At any time t, the mass of sand that has landed on the conveyor belt is given by:
\(
m = \mu t
\)
where μ is the rate of sand falling (in kg/s).
Step 3: Calculate the kinetic energy of the sand
- The kinetic energy (KE) of the mass of sand on the conveyor belt moving with velocity v is:
\(
KE = \frac{1}{2} m v^2 = \frac{1}{2} (\mu t) v^2
\)
- This simplifies to:
\(
KE = \frac{1}{2} \mu v^2 t
\)
Step 4: Find the rate of change of kinetic energy
- To find the rate at which work is done by the friction force, we need to differentiate the kinetic energy with respect to time t:
\(
\frac{d(KE)}{dt} = \frac{d}{dt} \left( \frac{1}{2} \mu v^2 t \right)
\)
- Since μ and v are constants, we can differentiate:
\(
\frac{d(KE)}{dt} = \frac{1}{2} \mu v^2 \cdot \frac{d(t)}{dt} = \frac{1}{2} \mu v^2
\)
Step 5: Conclusion
- The rate at which work is done by the friction force on the sand is:
\(
\text{Rate of work done by friction} = \frac{1}{2} \mu v^2
\)
Final Answer
The rate at which work is done by the friction force on the sand is \(\frac{1}{2} \mu v^2\).
---
- Sand is dropped vertically at a constant rate of μkgs onto a conveyor belt moving horizontally with a velocity v.
- When the sand particles land on the belt, they come to rest relative to the belt immediately.
- At any time t, the mass of sand that has landed on the conveyor belt is given by:
\(
m = \mu t
\)
where μ is the rate of sand falling (in kg/s).
- The kinetic energy (KE) of the mass of sand on the conveyor belt moving with velocity v is:
\(
KE = \frac{1}{2} m v^2 = \frac{1}{2} (\mu t) v^2
\)
- This simplifies to:
\(
KE = \frac{1}{2} \mu v^2 t
\)
- To find the rate at which work is done by the friction force, we need to differentiate the kinetic energy with respect to time t:
\(
\frac{d(KE)}{dt} = \frac{d}{dt} \left( \frac{1}{2} \mu v^2 t \right)
\)
- Since μ and v are constants, we can differentiate:
\(
\frac{d(KE)}{dt} = \frac{1}{2} \mu v^2 \cdot \frac{d(t)}{dt} = \frac{1}{2} \mu v^2
\)
- The rate at which work is done by the friction force on the sand is:
\(
\text{Rate of work done by friction} = \frac{1}{2} \mu v^2
\)
The rate at which work is done by the friction force on the sand is \(\frac{1}{2} \mu v^2\).
Topper's Solved these Questions
Similar Questions
Sand particles drop vertically at the rate of 2kgs−1 on a conveyor belt moving horizontally with a velocity of 0.2ms−1.
The extra force required to keep the belt moving is
View SolutionSand particles drop vertically at the rate of 2kgs−1 on a conveyor belt moving horizontally with a velocity of 0.2ms−1.
The extra force required to keep the belt moving is
View SolutionSand particles drop vertically at the rate of 2kgs−1 on a conveyor belt moving horizontally with a velocity of 0.2ms−1.
The time rate of change of kinetic energy of sand particles is
View SolutionSand is being dropped on a conveyor belt at the rate of Mkg/s . The force necessary to kept the belt moving with a constant with a constant velocity of vm/s will be.
View SolutionSand drops from a stationary hopper at the rate of 5kg/s on to a conveyor belt moving with a constant speed of 2m/s. What is the force required to keep the belt moving and what is the power delivered by the motor, moving the belt?
View SolutionAn object of mass 1 kg moving on a horizontal surface with initial velocity 8 m/s comes to rest after 10 s. If one wants to keep the object moving on the same surface with velocity 8 m/s the force required is
View SolutionA horizontal 50 N force acts on a 2 kg crate which is at rest on a smooth horizontal surface. At the instant the particle has gone 2 m, the rate at which the force is doing work is :
View SolutionA 16kg block moving on a frictionless horizontal surface with a velocity of 4m/s compresses an ideal spring and comes to rest. If the force constant of the spring be 100N/m, then how much is the spring commpressed ?
View SolutionAn inclined plane is moving up with constant velocity v. A block kept on incline is at rest. Calculate the work done by gravity, friction force, and normal reaciton on block in time interval of t.
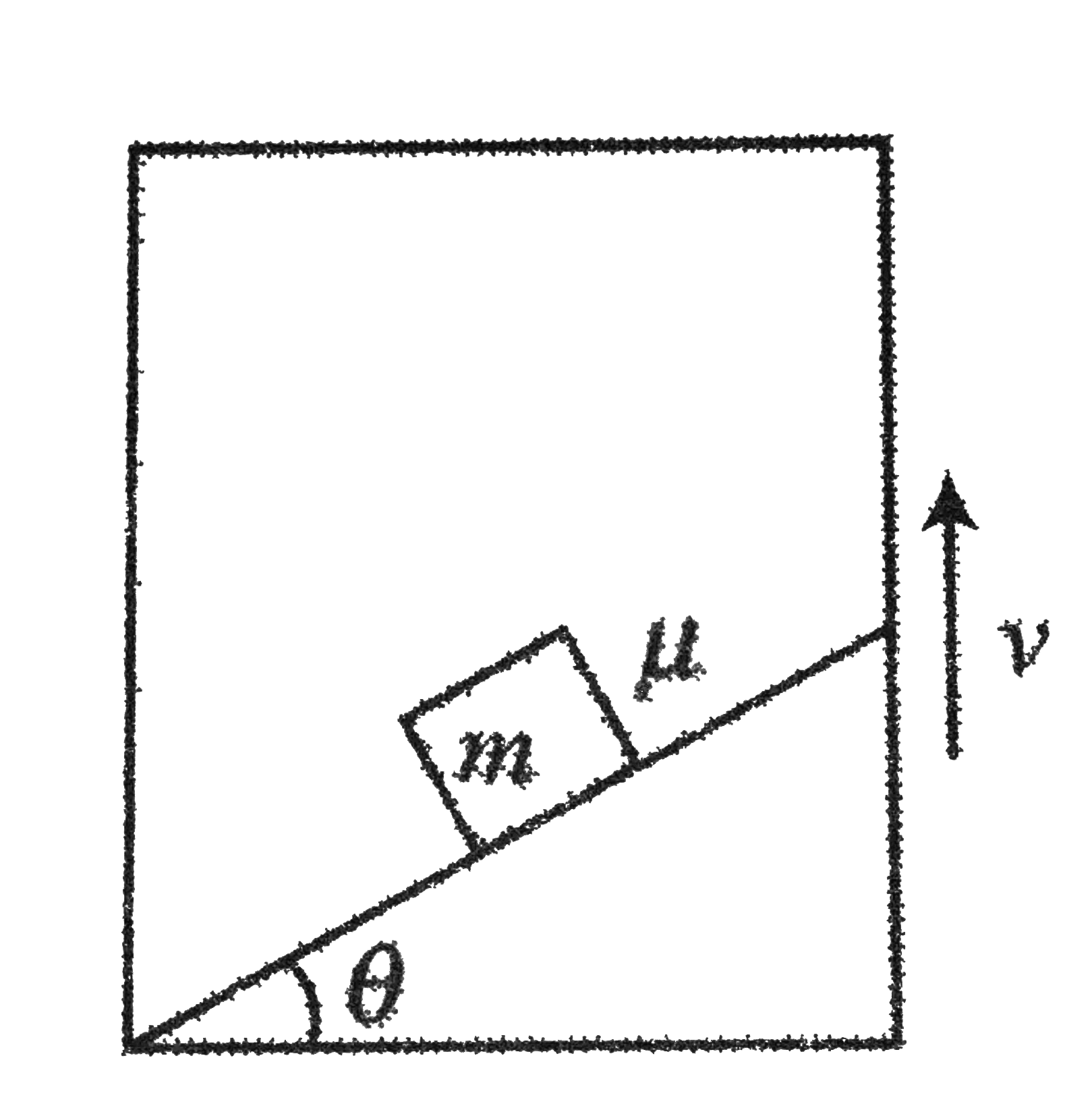
View SolutionA block is gently placed on a conveyor belt moving horizontally with constant speed. After t = 4s, the velocity of the block becomes equal to the velocity of the belt. If the coefficient of friction between the block and the belt is μ=0.2, then the velocity of the conveyor belt is (g=10ms−2)
View Solution
