A gas consisting of rigid diatomic molecules (degrees of freedom r = 5) under standard conditions (P0=105 Pa and T0=273K) was compressed adiabatically η=5 times. Find the mean kinetic energy of a rotating molecule in the final state.
To solve the problem step by step, we will follow the outlined approach in the video transcript.
Step 1: Understanding the Degrees of Freedom
For a rigid diatomic molecule, the degrees of freedom (r) are given as 5. This includes:
- 3 translational degrees of freedom (movement in x, y, z directions)
- 2 rotational degrees of freedom (rotation about two axes perpendicular to the bond axis)
Step 2: Mean Kinetic Energy of a Rotating Molecule
The mean kinetic energy (KE) associated with each degree of freedom is given by the formula:
KE=12kT
where k is the Boltzmann constant and T is the temperature in Kelvin. For rotational motion, since there are 2 degrees of freedom, the total mean kinetic energy due to rotation is:
KErotation=kT
Step 3: Determine the Final Temperature After Adiabatic Compression
For adiabatic processes, the relationship between the initial and final states can be described by the equation:
TfTi=(ViVf)γ−1
where:
- Tf is the final temperature
- Ti is the initial temperature
- Vi is the initial volume
- Vf is the final volume
- γ=CpCv is the heat capacity ratio
Given that the gas is compressed 5 times, we have:
Vf=Vi5
Thus,
ViVf=5
Step 4: Calculate Gamma
For a diatomic gas with 5 degrees of freedom:
γ=r+2r=5+25=75
Thus,
γ−1=25
Step 5: Substitute Values into the Adiabatic Equation
Substituting into the adiabatic equation:
TfTi=525
Given Ti=273K:
Tf=273×525
Step 6: Calculate 525
Calculating 525:
525≈2.236
Thus,
Tf≈273×2.236≈610.6K
Step 7: Calculate Mean Kinetic Energy
Now, substituting Tf into the mean kinetic energy formula:
KErotation=kTf
Using k=1.38×10−23J/K:
KErotation=1.38×10−23×610.6
Calculating this gives:
KErotation≈8.43×10−21J
Final Answer
The mean kinetic energy of a rotating molecule in the final state is approximately:
KErotation≈8.43×10−21J
For a rigid diatomic molecule, the degrees of freedom (r) are given as 5. This includes:
- 3 translational degrees of freedom (movement in x, y, z directions)
- 2 rotational degrees of freedom (rotation about two axes perpendicular to the bond axis)
The mean kinetic energy (KE) associated with each degree of freedom is given by the formula:
KE=12kT
where k is the Boltzmann constant and T is the temperature in Kelvin. For rotational motion, since there are 2 degrees of freedom, the total mean kinetic energy due to rotation is:
KErotation=kT
For adiabatic processes, the relationship between the initial and final states can be described by the equation:
TfTi=(ViVf)γ−1
where:
- Tf is the final temperature
- Ti is the initial temperature
- Vi is the initial volume
- Vf is the final volume
- γ=CpCv is the heat capacity ratio
Vf=Vi5
Thus,
ViVf=5
For a diatomic gas with 5 degrees of freedom:
γ=r+2r=5+25=75
Thus,
γ−1=25
Substituting into the adiabatic equation:
TfTi=525
Given Ti=273K:
Tf=273×525
Calculating 525:
525≈2.236
Thus,
Tf≈273×2.236≈610.6K
Now, substituting Tf into the mean kinetic energy formula:
KErotation=kTf
Using k=1.38×10−23J/K:
KErotation=1.38×10−23×610.6
Calculating this gives:
KErotation≈8.43×10−21J
The mean kinetic energy of a rotating molecule in the final state is approximately:
KErotation≈8.43×10−21J
Topper's Solved these Questions
Similar Questions
A gas consisting of rigid di-atomic molecules (degree of freedom =5) at pressure p0=105N/m2 and temperature 273K was compressed adiabatically 5 times. The mean kinetic energy of rotating molecules in final state is nx10−21J. Find value of 'n'.(K=1.38×10−23,(5)2/5=1.90).
View SolutionGaseous hydrogen contained initially under standard conditions in sealed vessel of volume V=5L was cooled by ΔT=55K. Find how much the internal energy of the gas will change and what amount of heat will be lost by the gas.
View SolutionA sample of helium gas is at a temperature of 300K and a pressure of 0.5atm. What is the average kinetic energy of a molecule of a gas ?
View SolutionAn ideal monoatomic gas Cv=1.5R initialy at 298 K and 1.013×106 Pa. pressure expands adiabatically unit it is a in equilibrium with a constant external pressure of 1.013×105 Pa. Calculate the final temperature of gas.
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
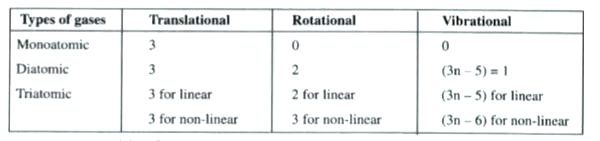
Where 'n' is atomicity of gas.
The rotational kinetic energy of H20 molecule is equal to
View SolutionIn a crude model of a rotating diatomic molecule of chlorine (Cl2), the two (Cl) atoms are 2.0×10−10m apart and rotate about their centre of mass withb angular speed ω=2.0×1012rad/s. What is the rotational kinetic energy of one molecule of Cl2 , Which has a molar mass of 70.0g/mol ?
 .
.
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
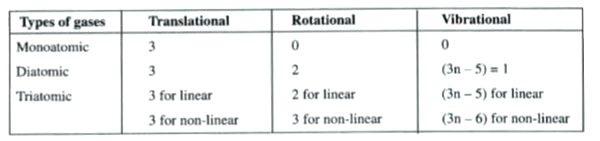
Where 'n' is atomicity of gas.
The vibrational kinetic energy of CO2 molecule is
View SolutionComprehension-3
An ideal gas initially at pressure p0 undergoes a free expansion (expansion against vacuum under adiabatic conditions) until its volume is 3 times its initial volume. The gas is next adiabatically compressed back to its original volume. The pressure after compression is 32/3p0.
The gas, is monoatomic, is diatomic, is polyatomic, type is not possible to decide from the given information. What is the ratio of the average kinetic energy per molecule in the final state to that in the initial state?
View SolutionAs adiabatic vessel contains n1=3 mole of diatomic gas. Moment of inertia of each molecule is I=2.76×10−46kgm2 and root-mean-square angular velocity is ω0=5×1012rad/s. Another adiabatic vessel contains n2=5 mole of a monatomic gas at a temperature 470K. Assume gases to be ideal, calculate root-mean-square angular velocity of diatomic molecules when the two vessels are connected by a thin tube of negligible volume. Boltzmann constant k=1.38×10−23J/:molecule.
View SolutionA ideal gas (γ=1.5) is expanded adiabatically. How many times has the gas to be expanded to reduce the root mean square velocity of molecules 2.0 times
View Solution
