A particle constrained to move along x-axis given a velocity u along the positive x-axis. The acceleration ' a ' of the particle varies as a = - bx, where b is a positive constant and x is the x co-ordinate of the position of the particle . Then select the correct alternative(s): .
The maximum displacement of the particle from the starting point is u√b
The particle will oscillate about the origin
Velocity is maximum at the origin
Given data is insufficient to determine the exact motion of the particle.
To solve the problem, we need to analyze the motion of a particle constrained to move along the x-axis with a given velocity and a specific form of acceleration. The acceleration is given as a=−bx, where b is a positive constant and x is the position of the particle.
1. Understand the relationship between acceleration, velocity, and position:
The acceleration a can be expressed as:
a=dvdt=dvdx⋅dxdt=vdvdx
where v is the velocity of the particle.
2. Set up the equation:
From the given acceleration a=−bx, we can equate:
vdvdx=−bx
3. Rearranging the equation:
Rearranging gives:
vdv=−bxdx
4. Integrate both sides:
We will integrate both sides. The left side integrates from the initial velocity u to v, and the right side integrates from 0 to x:
∫vuvdv=−b∫x0xdx
5. Perform the integrations:
The left side becomes:
v22∣∣∣vu=v22−u22
The right side becomes:
−b⋅x22∣∣∣x0=−bx22
6. Setting the equations equal:
Equating both sides gives:
v22−u22=−bx22
7. Simplifying the equation:
Rearranging the equation leads to:
v2=u2−bx2
8. Finding the maximum velocity:
The velocity v will be maximum when x=0. Substituting x=0 into the equation gives:
v2=u2
Thus, v=u when x=0.
9. Conclusion:
The particle has maximum velocity at the origin x=0.
Final Answer:
The correct alternative is that the velocity is maximum at the origin.
The acceleration a can be expressed as:
a=dvdt=dvdx⋅dxdt=vdvdx
where v is the velocity of the particle.
From the given acceleration a=−bx, we can equate:
vdvdx=−bx
Rearranging gives:
vdv=−bxdx
We will integrate both sides. The left side integrates from the initial velocity u to v, and the right side integrates from 0 to x:
∫vuvdv=−b∫x0xdx
The left side becomes:
v22∣∣∣vu=v22−u22
The right side becomes:
−b⋅x22∣∣∣x0=−bx22
Equating both sides gives:
v22−u22=−bx22
Rearranging the equation leads to:
v2=u2−bx2
The velocity v will be maximum when x=0. Substituting x=0 into the equation gives:
v2=u2
Thus, v=u when x=0.
The particle has maximum velocity at the origin x=0.
The correct alternative is that the velocity is maximum at the origin.
Topper's Solved these Questions
Similar Questions
The velocity of a particle moving in the positive direction of the X-axis varies as V=K√S where K is a positive constant. Draw V-t graph.
View SolutionA particle moves along x-axis such that its position veries with time as x=50t−5t2. Select the correct alternative (s).
View SolutionA particle moves in the plane xy with constant acceleration 'a' directed along the negative y-axis. The equation of motion of the particle has the form y=px−qx2 where p and q are positive constants. Find the velocity of the particle at the origin of co-ordinates.
View SolutionA particle moves in the plane xy with constant acceleration 'a' directed along the negative y-axis. The equation of motion of the particle has the form y=px−qx2 where p and q are positive constants. Find the velocity of the particle at the origin of co-ordinates.
View SolutionThe position x of particle moving along x-axis varies with time t as x=Asin(ωt) where A and ω are positive constants. The acceleration a of particle varies with its position (x) as
View SolutionA particle of unit mass is moving along x-axis. The velocity of particle varies with position x as v(x). =αx−β (where α and β are positive constants and x>0 ). The acceleration of the particle as a function of x is given as
View SolutionA particle located at position x=0, at time t=0, starts moving along the positive x-direction with a velocity v2=αx (where alpha is a positive constant). The displacement of particle is proportional to
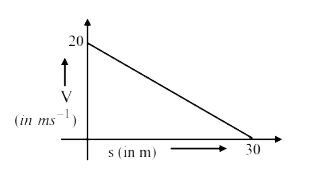
View SolutionThe velocity –position graph of a particle moving along a straight line is shown. Then acceleration of the particle at s = 15 m is :
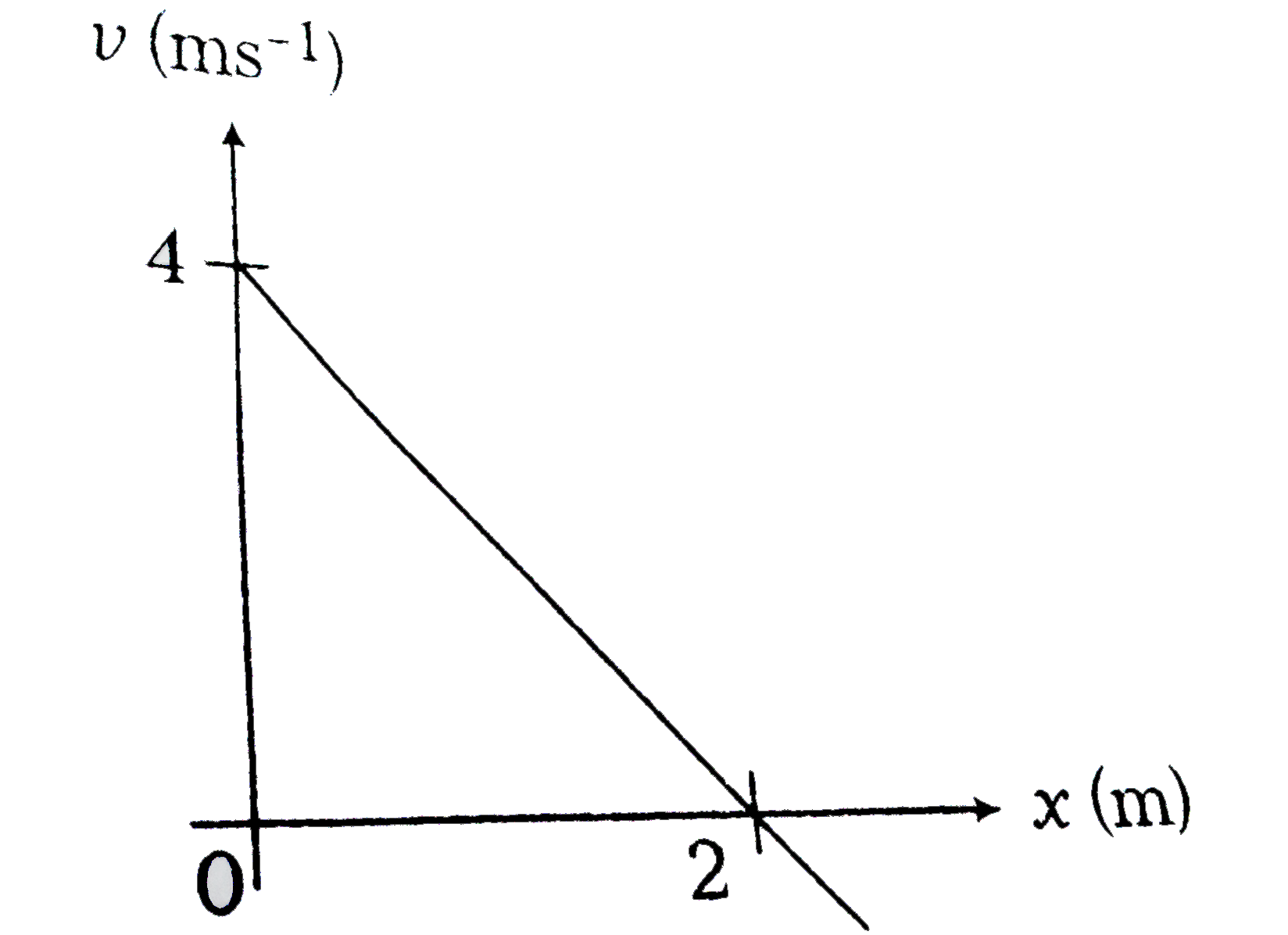
View SolutionThe velocity (υ) of a particle moving along X-axis varies with its position x as shown in figure. The acceleration (a) of particle varies with position (x) as
View SolutionA particle is moving along x-axis. Its velocity v with x co-ordinate is varying as v=√x. Then
View Solution
