The capacitor of capacitance 4μF and 6μF are connected in series. A potential difference of 500 volts applied to the outer plates of the two capacitor system. Then the charge on each capacitor is numerically
6000μ C
1200μC
1200 C
6000 C
To solve the problem of finding the charge on each capacitor when two capacitors of capacitance 4μF and 6μF are connected in series across a potential difference of 500 volts, we can follow these steps:
Step 1: Calculate the Equivalent Capacitance
For capacitors in series, the equivalent capacitance Ceq can be calculated using the formula:
1Ceq=1C1+1C2
Where:
- C1=4μF
- C2=6μF
Substituting the values:
1Ceq=14+16
Finding a common denominator (which is 12):
1Ceq=312+212=512
Thus,
Ceq=125μF=2.4μF
Step 2: Calculate the Charge on the Capacitors
The charge Q on the equivalent capacitor when a potential difference V is applied can be calculated using the formula:
Q=Ceq×V
Substituting the values:
Q=2.4μF×500V
Calculating this gives:
Q=1200μC
Step 3: Conclusion
Since the capacitors are in series, the charge on each capacitor is the same. Therefore, the charge on each capacitor is:
Q=1200μC
Summary of the Solution
The charge on each capacitor is 1200μC.
---
For capacitors in series, the equivalent capacitance Ceq can be calculated using the formula:
- C1=4μF
- C2=6μF
The charge Q on the equivalent capacitor when a potential difference V is applied can be calculated using the formula:
Since the capacitors are in series, the charge on each capacitor is the same. Therefore, the charge on each capacitor is:
The charge on each capacitor is 1200μC.
Similar Questions
Two capacitor of capacitance 4.0μFand 6.0muF' are connected in series with a battery of 20 V . Find the energy supplied by the battery .
View SolutionThree capacitors 3μF,6μF and 6μF are connected in series to source of 120 V. The potential difference in volt, across the 3μF capacitor will be
View Solution
Knowledge Check
- Question 1 - Select One
The potential difference between points A and B is
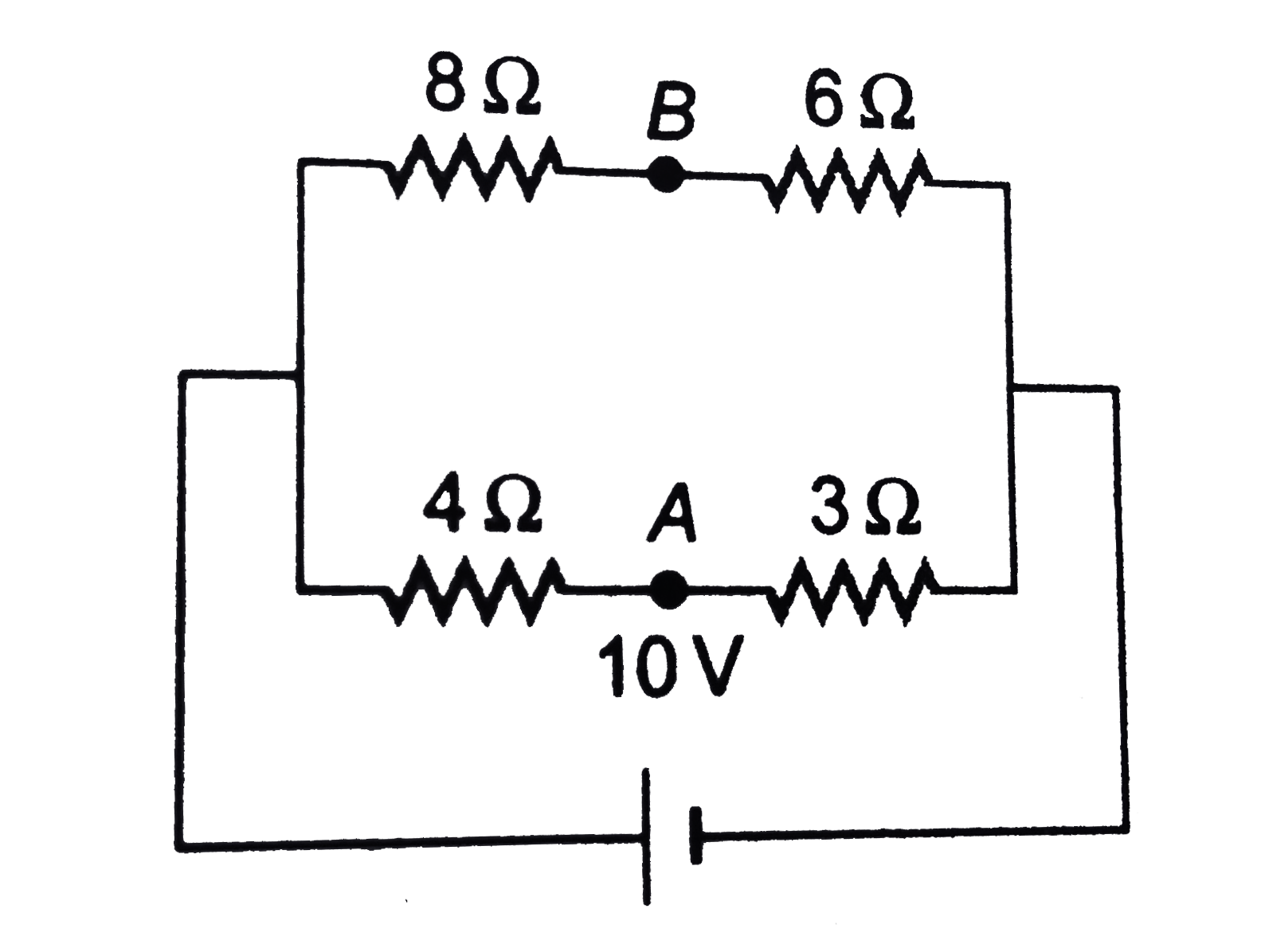 A2B5C11D18
A2B5C11D18
Two capacitors of capacitance of 6μFand12μF are connected in series with a battery. The voltage across the 6μF capacitor is 2V. Compute the total battery voltage.
View SolutionTwo capacitors of capacitance 2μF and 3μF are joined in series. Outer plate first capacitor is at 1000 volt and outer plate of second capacitor is earthed (grounded). Now the potential on inner plate of each capacitor will be
View SolutionTwo capacitors of capacitances 20.0pF and 50.0pF are connected in series with a 6.00V bettery . Find (a )the potential difference across each capacitor and (b) the energy stored in each capacitor .
View SolutionThree capacitors having capacitances of 8.4μF,8.4μF and 4.2μF are connected in series across a 36 potential difference.
(a) What is the charge on 4.2μF capacitor?
(b) What is the total energy stored in all three capacitors?
(c) The capacitors are disconnected from the potential difference without allowing them to discharge. They are then reconnected in parallel with each other, with the positively charged plates connected together. What is the voltage across each capacitor in the parallel combination?
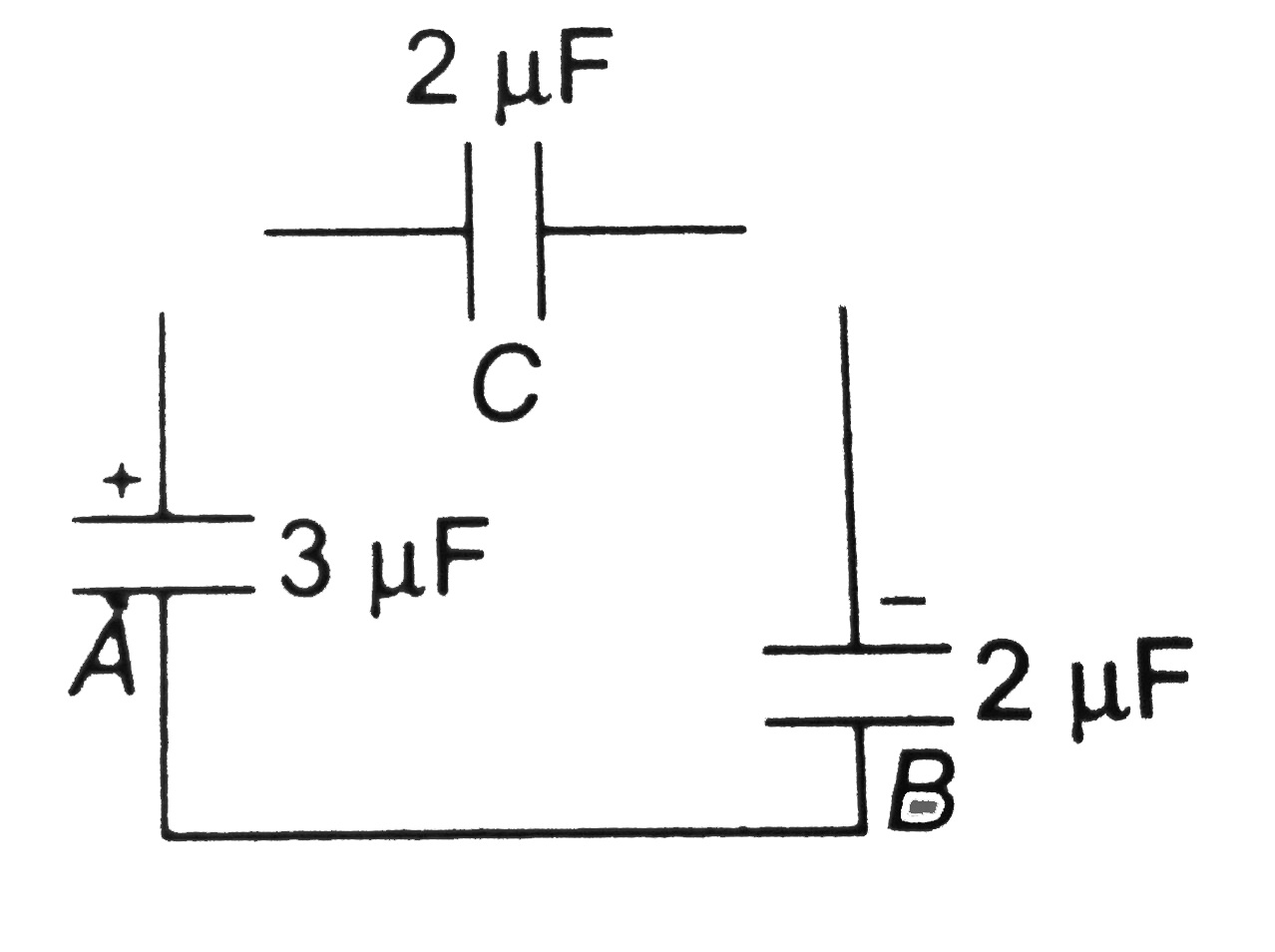
(d) What is the total energy now stored in the capacitors?View SolutionTwo capacitors A and B with capacities 3μF and 2μF are charged to a potential difference of 100V and 180V, respectively. The plates of the capacitors are connected as show in figure with one wire of each capacitor free. The upper plate of A is positive and that of B is negastive. An uncharged 2μF capcitor C with lead wires falls on the free ends to complete the circuit. Calculate
a. the final charge on the three capacitors.
b. the amount of electrostatic energy stored in the system before and after completion of the circuit.
 View Solution
View SolutionTwo capicitors of capacitances 4and6μF respectively are connected in series across a pd of 250 V. These are disconnected and now connected in parallel. Find the new pd and charge on each capacitor.
View SolutionTwo capacitor of capacitance 2μFand3μF respectively are charged to potential difference 20 V each. Now the capacitors are connected such that the positively chargd plate of one capacitory is connected to the negatively charged plate of the other. after the current in the circuit has become negligible, the potential difference across the 2μF capacitor is ____V.
View Solution
