For the damped oscillator shown in Figure, the mass m of the block is 400 g, k=45Nm−1 and the damping constant b is 80gs−1. Calculate .
(a) The period of osciallation ,
(b) Time taken for its amplitude of vibrations to drop to half of its initial value and
(c ) The time taken for its mechanical energy to drop to half its initial value.
(a) km=45×0.4=18kgNm−1=18kg2s−2
∴√km=4.243kgs−1
b=0.08kgs−1 is much less than √km . Hence, the time period T from equation ( 49) is given by
T=2π√mk
=2π√0.445=0.6s
(b) From equation ( 48), the time T1/2 for amplitude to drop to half of its initial value of given by T1/2=log(2)b2m
=0.6920.082×0.4
=6.93s
(c ) For calculateing the time t12 for its mechanical energy to drop to half its initial value we make use of equation (50)
E(t12)/E(0)=exp. ⎛⎜⎝−bt12m⎞⎟⎠
12=exp⎛⎜⎝−bt12m⎞⎟⎠
ln(12)=−bt12m
or t12=0.69280gs−1×400g
=3.46s
This is just half of the decay period for amplitude, which is not surprising, because, according to equations ( 48 ) and ( 50) , energy depends on the square of the amplitude. Notice that there is a factor of 2 in the exponents of the two exponentials.
Topper's Solved these Questions
- OSCILLATIONSBook:AAKASH INSTITUTE ENGLISHChapter:OSCILLATIONSExercise:Exercise
Explore 18 Videos
- OSCILLATIONSBook:AAKASH INSTITUTE ENGLISHChapter:OSCILLATIONSExercise:Assignment (Section - A) (OBJECTIVE TYPE QUESTIONS)
Explore 60 Videos
- OSCILLATIONSBook:AAKASH INSTITUTE ENGLISHChapter:OSCILLATIONSExercise:ASSIGNMENT ( SECTION-J )
Explore 8 Videos
- NUCLEIBook:AAKASH INSTITUTE ENGLISHChapter:NUCLEIExercise:ASSIGNMENT (SECTION-D)
Explore 10 Videos
- PHYSICAL WORLDBook:AAKASH INSTITUTE ENGLISHChapter:PHYSICAL WORLDExercise:ASSIGNMENT (Section-B)
Explore 4 Videos
Similar Questions
For the damped oscillator shown in Fig, the mass of the block is 200g,k=80Nm−1 and the damping constant b is 40gs−1 Calculate
The period of oscillation

View SolutionFor the small damping oscillator, the mass of the block is 500 g and value of spring constant is k = 50 N/m and damping constant is 10gs^(-1) The time period of oscillation is (approx.)
View Solution
Knowledge Check
In the question number 93, the time elapsed for its mechanical energy to drop half of its initial value is
A2.5 sB3.5 sC4.5 sD7.5 sThe half life of a radioactive substance is 20s, the time taken for the sample to decay by 7/8th of its initial value is
A20 sB40 sC60 sD80 sA block of mass 200 g executing SHM under the influence of a spring of spring constant k=90Nm−1 and a damping constant b=40gs−1. The time elapsed for its amplitude to drop to half of its initial value is (Given, ln (1/2)=−0.693)
A7sB9sC4sD11s
The displacement of a damped harmonic oscillator is given by
x(t)−e−0.1tcos(10πt+φ).Here t is in seconds.
The time taken for its amplitude of vibration to drop to half of its initial value is close to :
View Solution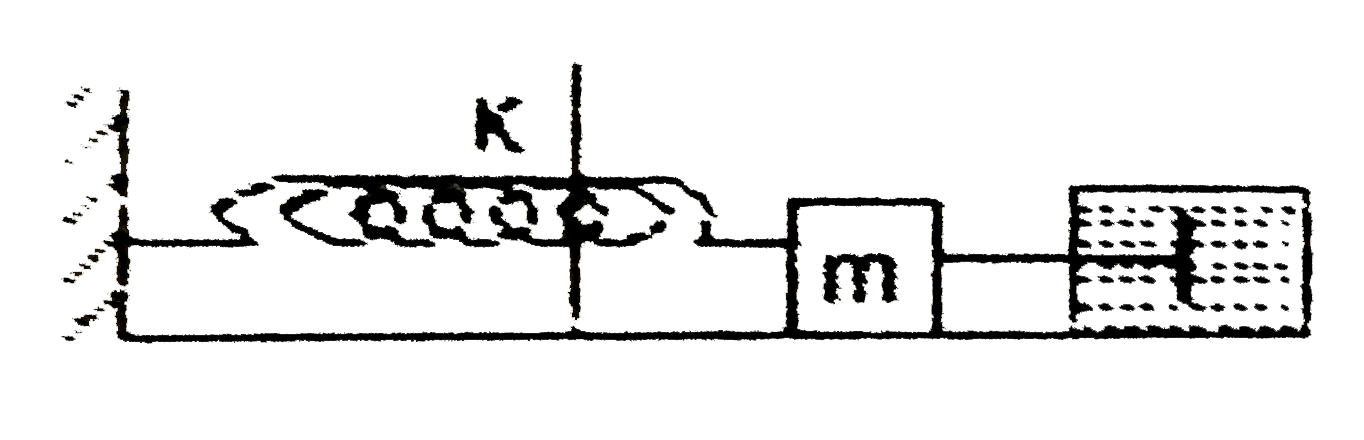
For a damped oscillator which follow the equation →F=−k→x−b→V
the mass of the block is 100 gm K=100N/M and the damping constant is 20 gm/sec. thhen find the time taken for its mechanical energy to drop to one-fourth of its initial value.
View SolutionFor the damped oscillator shown in previous figure, k=180Nm−1 and the damping constant b is 40gs−1 .Period of oscillation is given as 0.3 s, find the mass of the block . (Assume b is much less than √km).
Hint :T=2π√mk
View SolutionThe time period of oscillation of a simple pendulum is √2s. If its length is decreased to half of initial length, then its new period is
View SolutionThe time period of oscillation of the block as shown in figure is
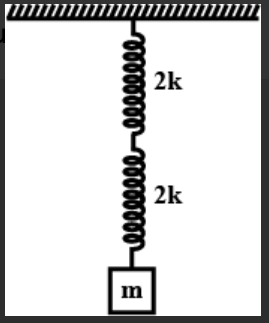
View Solution