A 2.5 kg collar is attached to a spring of spring constant 250Nm−1 . It slides without friction over a horizontal surface . It is displaced from its equilibrium position by 20 cm and releasd. Calculate the period of osciallation and the maximum speed.
To solve the problem step by step, we will calculate the period of oscillation and the maximum speed of the collar attached to the spring.
Step 1: Identify the given values
- Mass of the collar (m) = 2.5 kg
- Spring constant (k) = 250 N/m
- Displacement from equilibrium (x) = 20 cm = 0.2 m (convert to meters)
Step 2: Calculate the period of oscillation
The formula for the period (T) of a mass-spring system undergoing simple harmonic motion (SHM) is given by:
T=2π√mk
Substituting the known values into the formula:
T=2π√2.5kg250N/m
Calculating the fraction:
2.5250=0.01
Now, taking the square root:
√0.01=0.1
Now substituting back into the equation for T:
T=2π×0.1=2π10=π5seconds
Step 3: Calculate the maximum speed
The maximum speed (v_max) in SHM can be calculated using the formula that relates kinetic energy and potential energy. At maximum displacement (amplitude), all energy is potential:
12kx2=12mv2max
Rearranging for vmax:
vmax=√kmx2
Substituting the values:
vmax=√250N/m2.5kg(0.2m)2
Calculating the fraction:
2502.5=100
Now substituting back into the equation:
vmax=√100×(0.2)2=√100×0.04=√4=2m/s
Final Results
- The period of oscillation T=π5seconds
- The maximum speed vmax=2m/s
- Mass of the collar (m) = 2.5 kg
- Spring constant (k) = 250 N/m
- Displacement from equilibrium (x) = 20 cm = 0.2 m (convert to meters)
The formula for the period (T) of a mass-spring system undergoing simple harmonic motion (SHM) is given by:
The maximum speed (v_max) in SHM can be calculated using the formula that relates kinetic energy and potential energy. At maximum displacement (amplitude), all energy is potential:
- The period of oscillation T=π5seconds
- The maximum speed vmax=2m/s
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - Exercise18videos
OSCILLATIONS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - Assignment (Section - A) (OBJECTIVE TYPE QUESTIONS)60videos
OSCILLATIONS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT ( SECTION-J )8videos
OSCILLATIONS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)10videos
NUCLEI
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)4videos
PHYSICAL WORLD
Similar Questions
A 10 kg collar is attached to a spring of spring constant 1000Nm−1 . It slides without friction over a horizontal surface. If is displaced from its equilibrium position by 20 cm and released . Calculate
(a) The period of oscillation (b) The maximum speed
Hint :T=2π√mk,vm=Aω=A√kmView SolutionA 5 kg collar is attached to a spring of spring constant 500Nm−1 . It slides without friction over a horizontal rod. The collar is displaced from its equilibrium position by 10 cm and released. Calculate (a) The period of oscillations (b) The maximum speed and (c) maximum acceleration of the collar.
View Solution
Knowledge Check
- Question 1 - Select One
A 5 kg collar is attached to a spring of spring constant 500 N m−1. It slides without friction over a horizontal rod. The collar is displaced from its equilibrium position by 10 cm and released. The time period of oscillation is
Aπ5sBπ2sCπsD2πs
A 2.5 kg collar attached to a spring of foce constant 1000 Nm−1 slides without friction on a horizontal rod. The collar is displaced from its equilibrium position by 5.0 cm and released. Calculate (i) the period of oscillation (ii) the maximum speed of the collar and (iii) the maximum acceleration of the collar.
View SolutionA 5kg collar is attached to a spring . It slides without friction over a horizontal surface. It is displaced from its equilibrium position by 10 cm and released , its maximum speed is 1ms−1 Calculate
(a) Spring constant
(b) The period of oscillation
(c ) Maximum acceleration of the collar
Hint :m=5kg,A=0.1m,k=?,T=?or a=?,vm=1ms−1
vm=Aω=A√km
Find k,
T=2π√mk
amax=ω2A=kmAView SolutionA 4 kg roller is attached to a massless spring of spring constant k = 100 N/m. It rolls without slipping along a frcitionless horizontal road. The roller is displaced from its equilibrium position by 10 cm and then released. It rolls without slipping along a frictionless horizontal road. The roller is displaced from its equilibrium position by 10 cm and then released. Its maximum speed will be
View SolutionA trolley of mass 3.0kg is connected to two identical spring each of force constant 600Nm−1 as shown in figure. If the trolley is displaced from its equilibrium position by 5.0 cm and released, what is
(a) the period of ensuing oscillation?
(b) the maximum speed of trolley?
(c) How much is the total energy dissipated as heat by the time the trolley comes to rest due to damping forces?
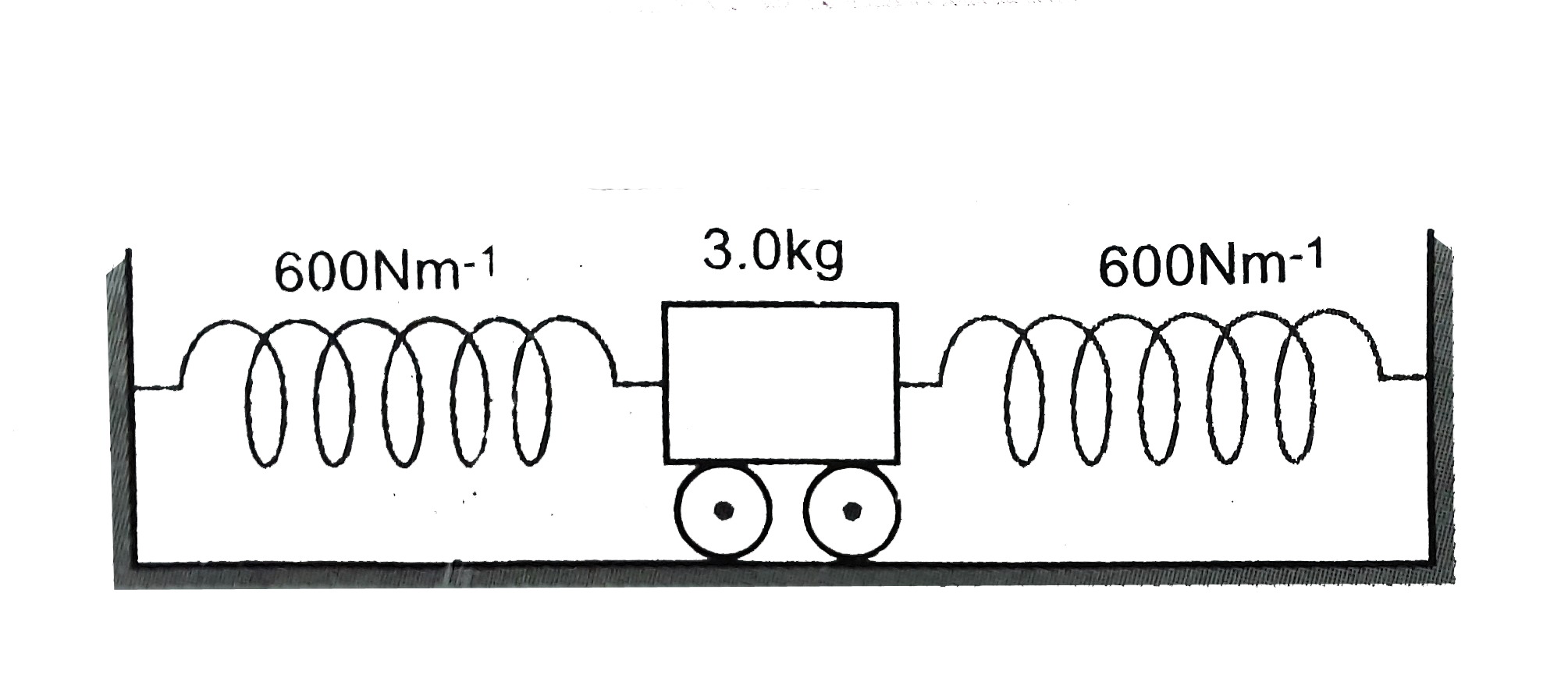 View Solution
View SolutionA mass of 1.5 kg is connected to two identical springs each of force constant 300Nm−1 as shown in the figure. If the mass is displaced from its equilibrium position by 10cm, then the period of oscillation is
 View Solution
View SolutionA block of mass m hangs from a vertical spring of spring constant k. If it is displaced from its equilibrium position, find the time period of oscillations.
View SolutionA mass of 1.5 kg is connected to two identical springs each of force constant 300Nm−1 as shown in the figure. If the mass is displaced from its equilibrium position by 10 cm, then maximum speed of the trolley is
 View Solution
View Solution
