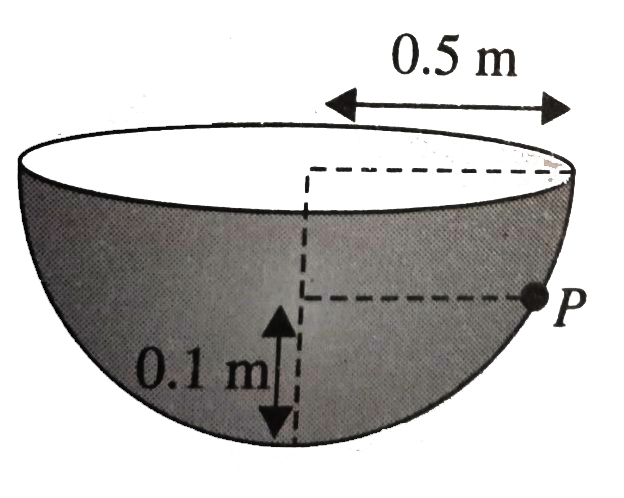
A small mass of 10 g lies in a hemispherical bowl of radius 0.5 m at a height of 0.1 m from the bottom of the bowl. The mass will be in equilibrius if the bowl rotates at an
10√3rads
10√3rads
5rads
none of these
The correct Answer is:C


Nsinθ=mrω2
Nsinθ=mrω2
tanθ=rω2g
ω=√gtanθr
ω=5rads
Similar Questions
How many litres of milk can a hemispherical bowl of diameter 10.5 cm hold?
View SolutionA hemispherical bowl is made of steel 0.5 cm thick. The inside radius of the bowl is 4cm.
View SolutionA hemispherical bowl has a radius of 3.5 cm. What would be the volume of water it would contain?
View SolutionA small ball of radius r rolls without sliding in a big hemispherical bowl. of radius R. what would be the ratio of the translational and rotaional kinetic energies at the bottom of the bowl.
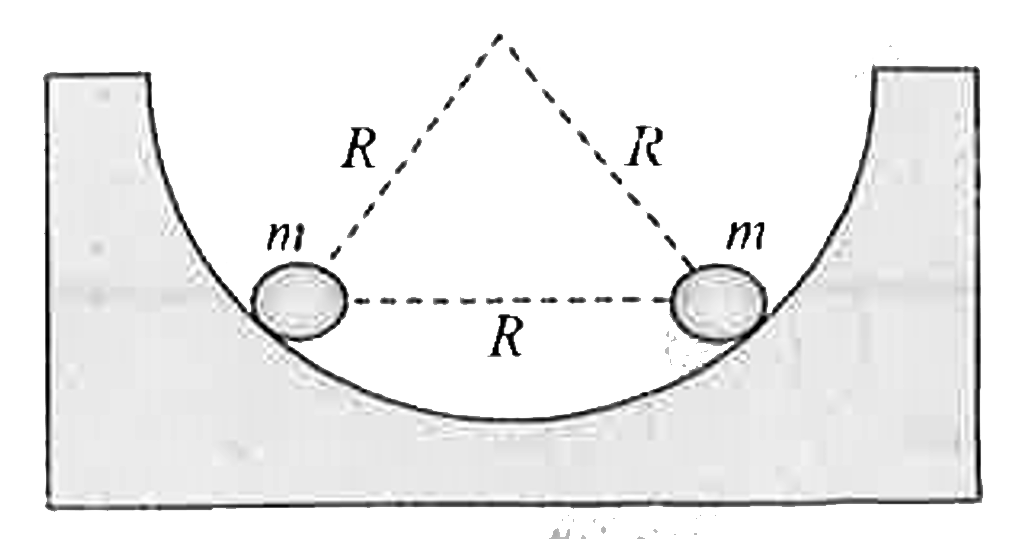
View SolutionTwo identical small balls each have a mass m and charge q. When placed in a hemispherical bowl of radius R with frictionless, nonconductive walls, the bead move, and at equilibrium the line joining the balls is horizontal and the distance between them is R (see figure). Neglect any induced charge on the hemispherical bowl. Then the charge on each bead is : (here K=14πε0)
View SolutionA hemispherical bowl is made of steel 0.5 cm thick. The inside radius of the bowl is 4cm. find volume of steel
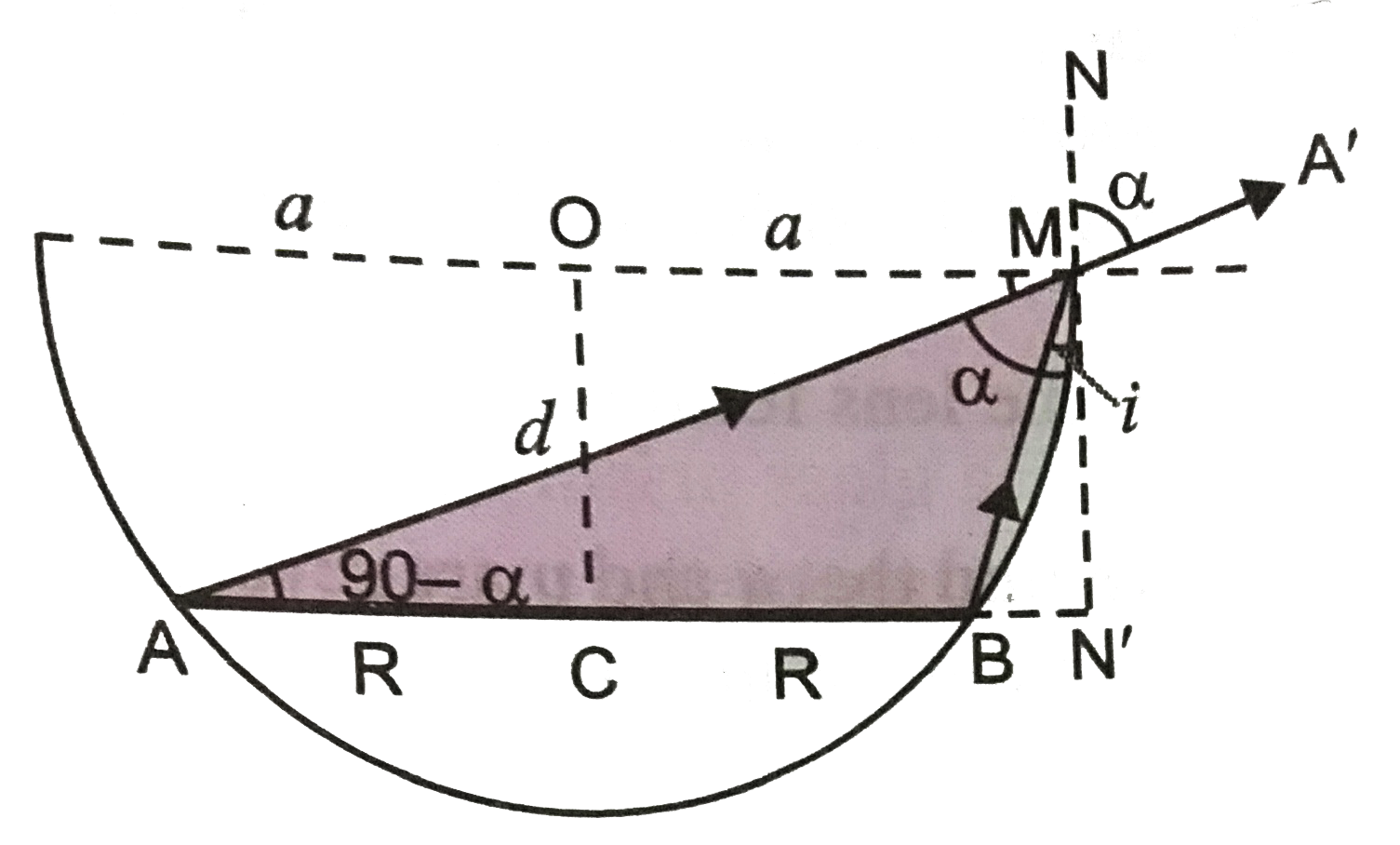
View SolutionA circuilar disc of radius 'R' is placed co-axially and horizontally inside and opaque hemispherical bowl of radius 'a', Fig. The far edge of the disc is just visible when viewed from the edge of the bowl. The bowl is filled with transparent liquid of refractive index μ and the near edge of the dise becomes just visible. How far below the top of the bowl is the disc placed ?
 .
.
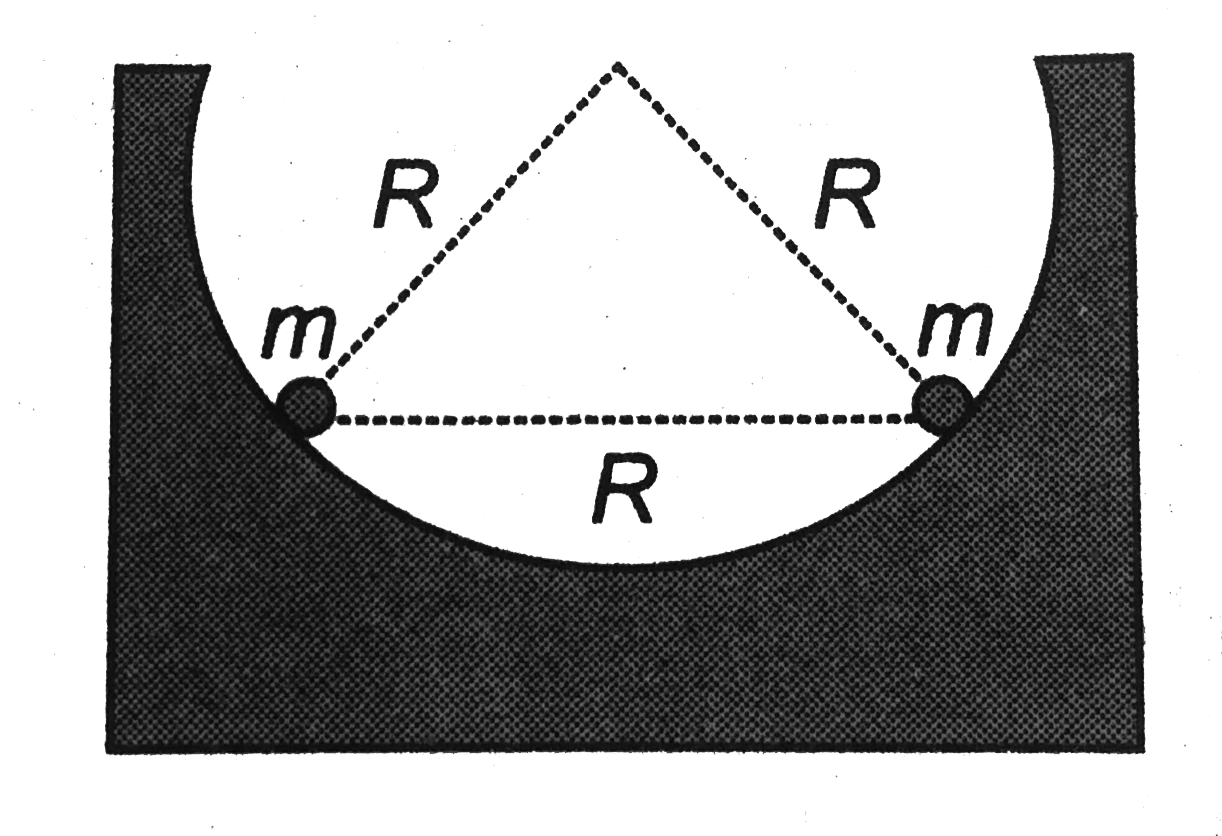
View SolutionTwo identical beads each have a mass m and charge q. When placed in a hemispherical bowl of radius R with frictionless, non-conducting walls, the beads move, and at equilibrium they area distance R apart (figure). Determine the charge on each bead.
View SolutionA hemispherical bowl is made of steel, 0.25 cm thick. The inner radius of the bowl is 5 cm. Find the outer curved surface area of the bowl.
View SolutionA hemispherical bowl is made of steel, 0.25 cm thick. The inner radius of the bowl is 5 cm. Find the outer curved surface area of the bowl.
View Solution
