A particle P will be in equilibrium inside a hemispherical bowl of radius 0.5 m at a height 0.2 m from the bottom when the bowl is rotated at an angular speed (g=10m/sec2)

10/√3 rad/sec
10√3 rad/sec
10 rad/sec
√20 rad/sec
The correct Answer is:A
Step by step video, text & image solution for A particle P will be in equilibrium inside a hemispherical bowl of radius 0.5 m at a height 0.2 m from the bottom when the bowl is rotated at an angular speed (g=10m//sec^(2)) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- WORK, POWER & ENERGY BOOK - MOTIONCHAPTER - WORK, POWER & ENERGY EXERCISE - Exercise - 3 Section-A
25 Videos
- WORK, POWER & ENERGY BOOK - MOTIONCHAPTER - WORK, POWER & ENERGY EXERCISE - Exercise - 3 Section-B
22 Videos
- WORK, POWER & ENERGY BOOK - MOTIONCHAPTER - WORK, POWER & ENERGY EXERCISE - Exercise - 1
42 Videos
- WAVESBOOK - MOTIONCHAPTER - WAVESEXERCISE - EXERCISE -4 (Level -II) (previous year JEE Advanced)
10 Videos
Similar Questions
Knowledge Check
A particle P will be in equilibrium inside a hemispherical bowl of radius 0.5 m at a height 0.2 m from the bottom when the bowl is rotated at an angular speed ( g = 10 m/sec2)
 A10/√3rad/secB10√rad/secC10rad/secD√20rad/sec
A10/√3rad/secB10√rad/secC10rad/secD√20rad/sec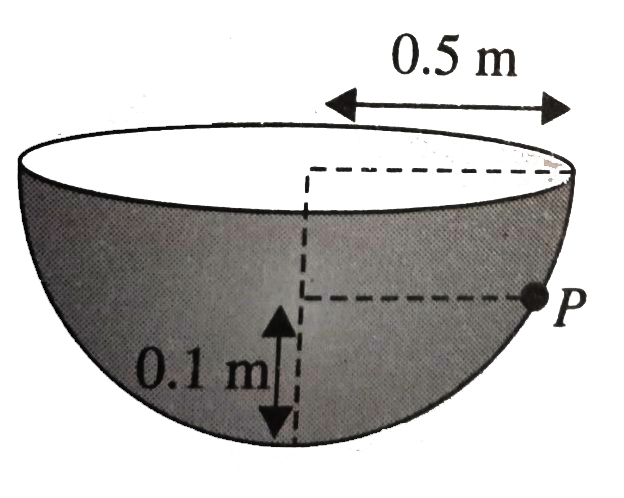
A small mass of 10 g lies in a hemispherical bowl of radius 0.5 m at a height of 0.1 m from the bottom of the bowl. The mass will be in equilibrius if the bowl rotates at anA10√3radsB10√3radsC5radsDnone of theseWhen a circular disc of radius 0.5 m is rotating about its own axis, then the direction of its angular momentum is
Aalong the tangent drawn at every pointBradialCperpendicular to the direction of angular velocityDalong the axis of rotation of the body
A hemispherical bowl of radius R is rotating about its own axis (which is vertical) with an angular velocity ω . A particle on the frictionless inner surface of the bowl is also rotating with the same ω . The particle is a height h from the bottom of the bowl.
(i) Obtain the relation between h and ω _________
(ii) Find minimum value of ω needed, in order to have a non-zero value of h _____________ .
View SolutionA particle of mass m is released in a smooth hemispherical bowl from shown position A. Find work done by gravity as it reaches the lowest point B.
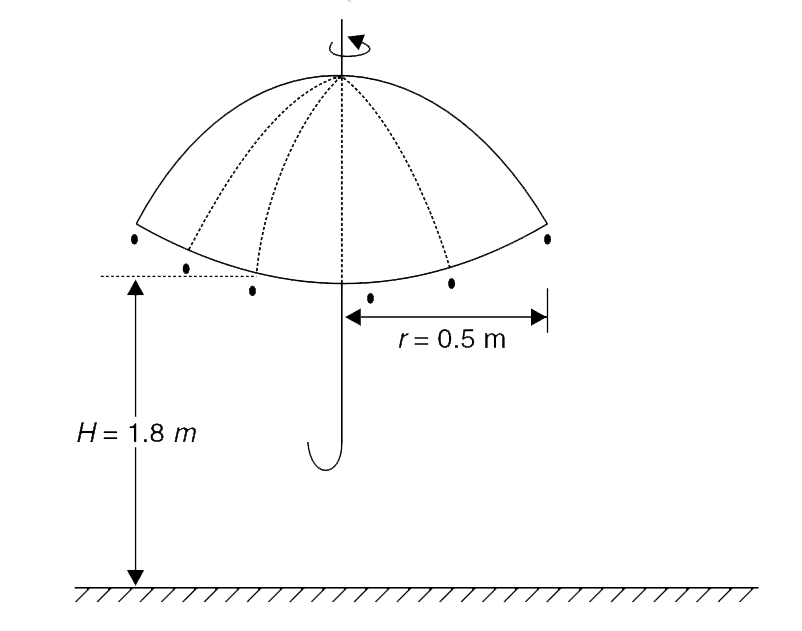
View SolutionA wet umbrella is held upright (see figure). The man holding it is rotating it about its vertical shaft at an angular speed of ω=5 rad s–1. The rim of the umbrella has a radius of r=0.5 m and it is at a height of H=1.8 m from the floor. The man holding the umbrella gradually increases the angular speed to make it 2ω. Calculate the area of the floor that will get wet due to water drops spun off the rim and hitting the floor. [g=10m/s2].
View SolutionA 2 g ball of glass is released from the edge of a hemispherical cup whose radius is 20 cm. What is the speed of the ball when it reaches the bottom of the cup? (Take g=10ms−2)

View SolutionA particle of mass 2 kg is on a smooth horizontal table and moves in a circular path of radius 0.6 m. The height of the table from the ground is 0.8 m. If the angular speed of the particle is 12 rad s−1 , the magnitude of its angular momentum about a point on the ground right under the centre of the circle is
View Solution
