A stone is projected from level ground at sec such that its horizontal and vertical components of initial velocity are and respectively. Then the instant of time at which tangential and normal components of acceleration of stone are same is: (neglect air resistance).
3 sec
4 sec
The correct Answer is:C
To solve the problem, we need to find the time at which the tangential and normal components of acceleration of a stone projected at an angle are equal. Let's break down the solution step by step.
Step 1: Understand the motion components
The stone is projected with:
- Horizontal component of initial velocity, ux=10m/s
- Vertical component of initial velocity, uy=20m/s
The acceleration due to gravity, g=10m/s2, acts downwards.
Step 2: Identify the components of acceleration
In projectile motion:
- The tangential component of acceleration at is due to the change in the vertical velocity, which is affected by gravity.
- The normal component of acceleration an is due to the change in direction of the velocity vector.
Since there is no horizontal acceleration, the horizontal component of velocity Vx remains constant at 10m/s.
Step 3: Determine the angle of projection
The angle θ of the velocity vector can be determined using the initial velocity components:
tanθ=uyux=2010=2⇒θ=tan−1(2)
Step 4: Set up the equations for tangential and normal acceleration
At any time t:
- The vertical component of velocity Vy can be expressed as:
Vy=uy−gt=20−10t
The tangential acceleration at is given by:
at=gsinθ
And the normal acceleration an is given by:
an=gcosθ
Step 5: Equate tangential and normal components of acceleration
We need to find the time t when at=an:
gsinθ=gcosθ
This simplifies to:
tanθ=1⇒θ=45∘
Step 6: Find the time when the angle is 45 degrees
Using the relationship between the vertical and horizontal components of velocity:
tanθ=VyVx
At θ=45∘:
Vy=Vx⇒20−10t=10
Solving for t:
20−10t=10⇒10=10t⇒t=1s
Step 7: Calculate total time of flight
The total time of flight T for the projectile can be calculated using:
T=2uyg=2×2010=4s
Step 8: Determine the time when tangential and normal accelerations are equal
Since the projectile takes 4 seconds to reach the ground, and we found that t=1s corresponds to the time when at=an, we need to find the total time from the start to this point:
- The time taken to reach the point where at=an is 1s.
- The time to reach the maximum height (where Vy=0) is 2s (half of the total time of flight).
Thus, the time at which the tangential and normal components of acceleration are equal is:
t=3s
Final Answer
The instant of time at which tangential and normal components of acceleration of the stone are the same is 3 seconds.
---
The stone is projected with:
- Horizontal component of initial velocity, ux=10m/s
- Vertical component of initial velocity, uy=20m/s
In projectile motion:
- The tangential component of acceleration at is due to the change in the vertical velocity, which is affected by gravity.
- The normal component of acceleration an is due to the change in direction of the velocity vector.
The angle θ of the velocity vector can be determined using the initial velocity components:
tanθ=uyux=2010=2⇒θ=tan−1(2)
At any time t:
- The vertical component of velocity Vy can be expressed as:
Vy=uy−gt=20−10t
at=gsinθ
And the normal acceleration an is given by:
an=gcosθ
We need to find the time t when at=an:
gsinθ=gcosθ
This simplifies to:
tanθ=1⇒θ=45∘
Using the relationship between the vertical and horizontal components of velocity:
tanθ=VyVx
At θ=45∘:
Vy=Vx⇒20−10t=10
Solving for t:
20−10t=10⇒10=10t⇒t=1s
The total time of flight T for the projectile can be calculated using:
T=2uyg=2×2010=4s
Since the projectile takes 4 seconds to reach the ground, and we found that t=1s corresponds to the time when at=an, we need to find the total time from the start to this point:
- The time taken to reach the point where at=an is 1s.
- The time to reach the maximum height (where Vy=0) is 2s (half of the total time of flight).
t=3s
The instant of time at which tangential and normal components of acceleration of the stone are the same is 3 seconds.
Topper's Solved these Questions
- CENGAGE PHYSICS DPPBook:CENGAGE PHYSICS ENGLISHChapter:CENGAGE PHYSICS DPPExercise:subjective type
Explore 51 Videos
- CENGAGE PHYSICS DPPBook:CENGAGE PHYSICS ENGLISHChapter:CENGAGE PHYSICS DPPExercise:Multiple correct Answer Type
Explore 54 Videos
- CAPACITOR AND CAPACITANCEBook:CENGAGE PHYSICS ENGLISHChapter:CAPACITOR AND CAPACITANCEExercise:Integer
Explore 5 Videos
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICS ENGLISHChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Single Answer Correct Type
Explore 22 Videos
Similar Questions
A stone is projectef from level ground such that its horizontal and vertical components of initial velocity are ux=10ms and uy=20ms respectively. Then the angle between velocity vector of stone one second before and one second after it attains maximum height is:
View SolutionA body is projected with an initial velocity of 58.8 m/s at angle 60° with the vertical. The vertical component of velocity after 2 sec is
View SolutionA projectile is fired at an angle of 30∘ to the horizontal such that the vertical component of its initial velocity is 80m/s. Its time of fight is T. Its velocity at t=T/4 has a magnitude of nearly.
View SolutionA particle is projected with velocity 20√2m/s at 45∘ with horizontal. After 1s , find tangential and normal acceleration of the particle. Also, find radius of curvature of the trajectory at that point. (Take g=10m/s2)
View SolutionA body is projected with an initial Velocity 20 m/s at 60° to the horizontal. Its initial velocity vector is- (g=10 m/s2)
View SolutionTwo stones are projected from level ground. Trajectory of two stones are shown in figure. Both stones have same maximum heights above level ground as shown. Let T1 and T2 be their time of flights and u1 and u2 be their speeds of projection respectively (neglect air resistance). then
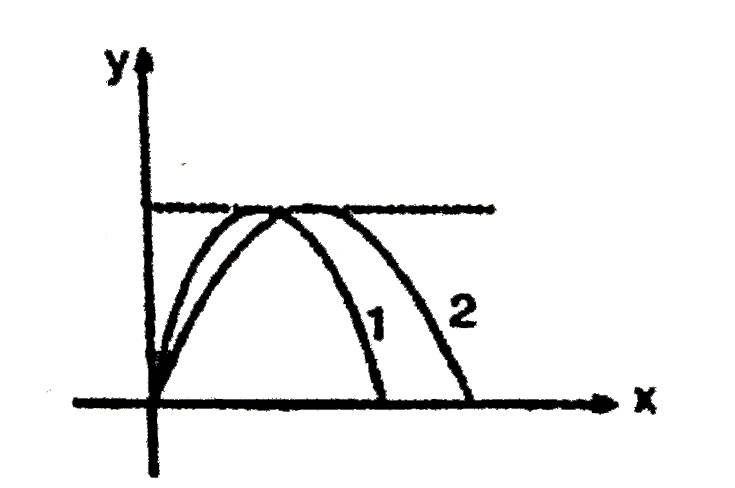
View SolutionA body is thrown into air with a velocity 5 m/s making an angle 30∘ with the horizontal .If the vertical component of the velocity is 5 m/s what is the velocity of the body ? Also find the horizontal component .
View SolutionA stone is dropped from a height 300 m and at the same time another stone is projected vertically upwards with a velocity of 100m/sec. Find when and where the two stones meet.
View SolutionA stone is projected with speed 20m/s at angle 37∘ with the horizontal and it hits the ground with speed 12m/s due to air resistance. Assume the effect of air resistance to reduce only horizontal component of velocity. Then the time of flight will be-
View SolutionA stone is projected from the ground with a velocity of 20 m/s at angle 30∘ with the horizontal. After one second it clears a wall then find height of the wall. (g=10ms−2)
View Solution