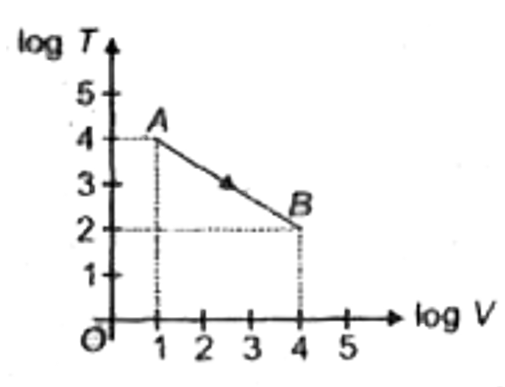
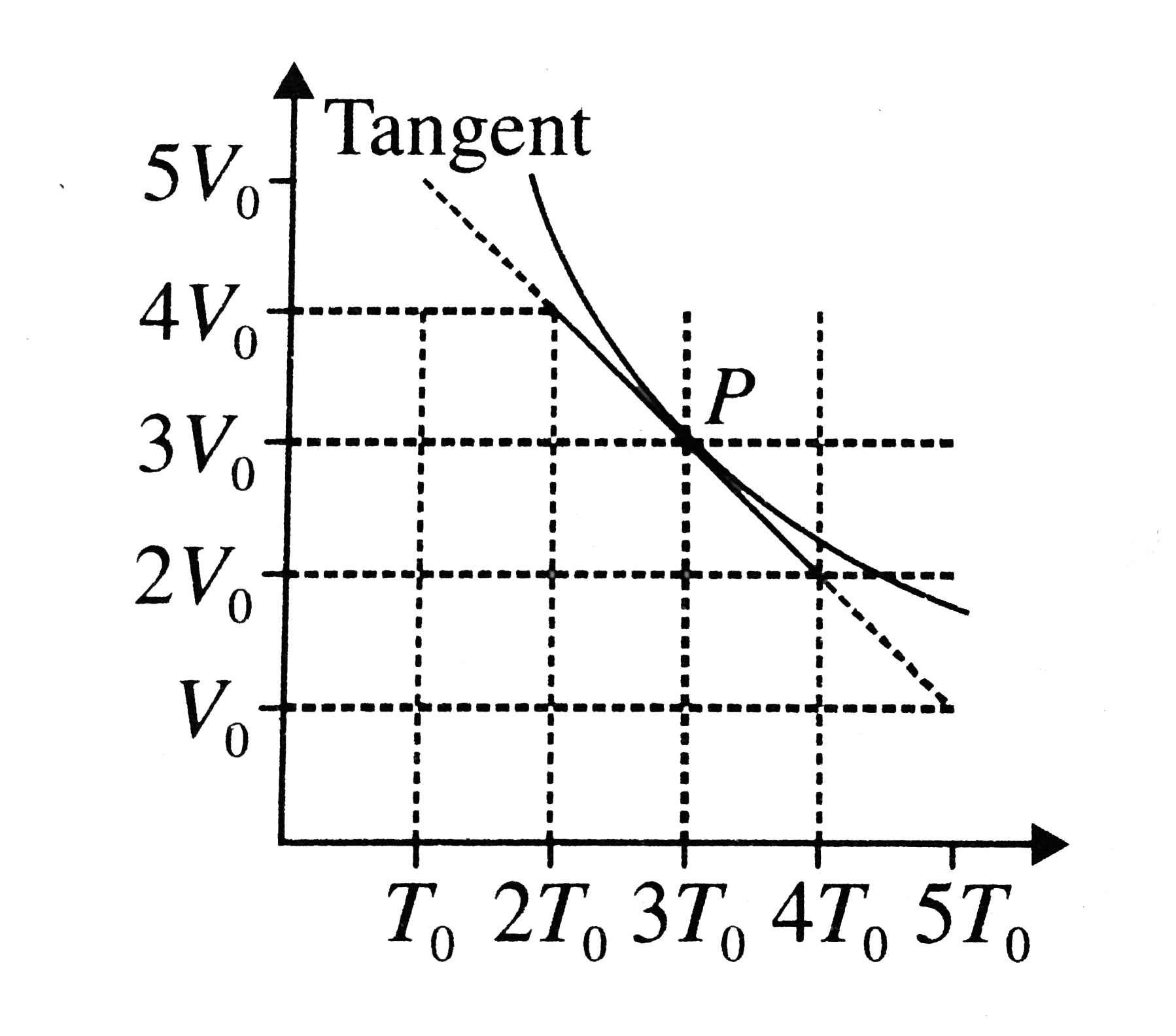
Figure shows the adiabatic curve for n moles of an ideal gas, the bulk modulus for the gas corresponding to the point P will be
A
5nRT03V0
B
nR(2+T0V0)
C
nR(1+T0V0)
D
2nRT0V0
Video Solution
Text Solution
The correct Answer is:D
d. For adiabatic process :
Bulk modulus : B=γP
for point p:P=nRTV=nR3T03V0=nRT0V0
⇒B=γnRT0V0
Now TVγ−1= constant
⇒(γ−1)TdV+VdT=0
⇒dVdT=−V(γ−1)T
for point P→
−3V03T0=−(3V0)(γ−1)(eT0)0
⇒γ=2
so from Eq. (i) B=2nRT0V0
|
Topper's Solved these Questions
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Multiple Corrects
Explore 29 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Assertion-Reasoning
Explore 6 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Subjective
Explore 22 Videos
- KINETIC THEORY OF GASESBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASESExercise:Compression
Explore 2 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBook:CENGAGE PHYSICS ENGLISHChapter:LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONExercise:Single correct anwer type
Explore 14 Videos
Similar Questions
Knowledge Check
In an adiabatic expansion of an ideal gas -
A(a) W=−ΔUB(b) W=ΔUC(c) ΔU=0D(d) W=0
For n moles of ideal gas, the equation of state may be written as
View SolutionFor adiabatic process of an ideal gas the value of dPP is equals to
View SolutionDuring an adiabatic reversibly expansion of an ideal gas
View SolutionThe bulk modulus of an ideal gas at constant temperature
View SolutionAdiabatic expansion of an ideal gas is accompanied by
View SolutionCurve in the figure shows an adiabatic compression of an ideal gas from 15m3 to 12m3, followed by an isothermal compression to a final volume of 3.0m3. There are 2.0 moles of the gas. Total heat supplied to the gas is equal to: (ln2= 0.693)
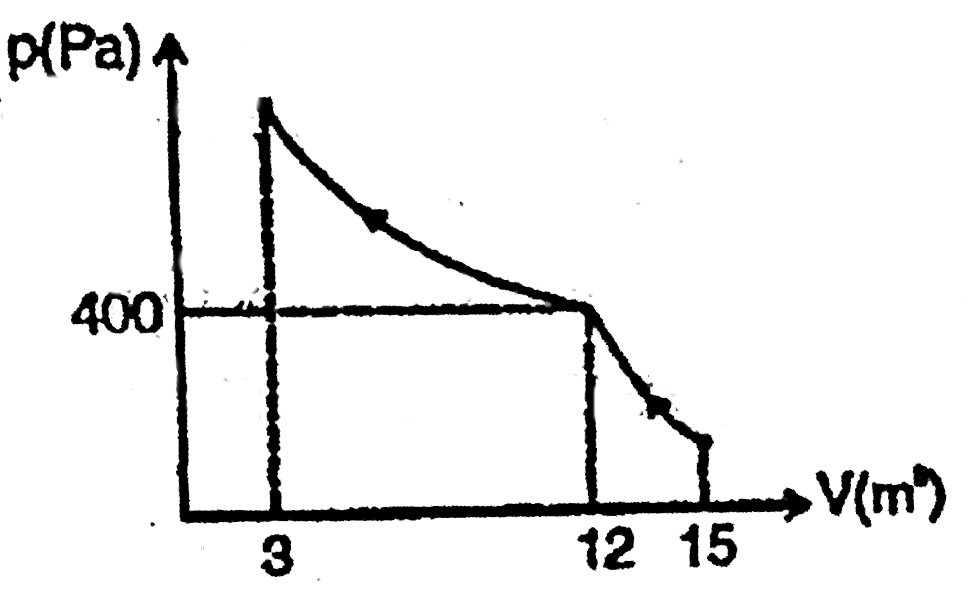
View SolutionFor a reversible adiabatic expansion of an ideal gas dp/p is equal to
View Solution
_S01_085_Q01.png)