A sound wave passing volume 1m3 is divided into two equal compartments by a partition. One of these compartments contains an ideal gas at 300K. The other compartment is vacuum. The whole system is thermally isolated from its surroundings. The partition is removed and the gas expands to occupy the whole volume of the container. Its temperature now would be
8.97×10−4K
8.97×10−6K
8.97×10−8K
none of these
The correct Answer is:C
To solve the problem step by step, we can follow these logical steps:
Step 1: Understand the System
We have a volume of 1 m³ divided into two compartments. One compartment contains an ideal gas at a temperature of 300 K, and the other compartment is a vacuum. The system is thermally isolated from its surroundings.
Hint: Visualize the setup to understand the initial conditions of the gas and vacuum.
Step 2: Identify the Process
When the partition is removed, the gas expands to fill the entire volume. This process is known as free expansion, where the gas expands into a vacuum without doing any work on the surroundings.
Hint: Recall the definition of free expansion and how it differs from other types of gas expansion.
Step 3: Apply the First Law of Thermodynamics
According to the first law of thermodynamics, the change in internal energy (ΔU) is related to heat added to the system (ΔQ) and work done by the system (ΔW) by the equation:
ΔQ=ΔU+ΔW
Since the system is thermally isolated, there is no heat exchange with the surroundings:
ΔQ=0
Hint: Remember that in an isolated system, energy cannot enter or leave the system.
Step 4: Analyze Work Done
In free expansion, the gas does not perform any work because it expands into a vacuum (no external pressure to work against):
ΔW=0
Hint: Consider the implications of expanding into a vacuum on the work done.
Step 5: Relate Internal Energy Change to Temperature Change
From the first law, we have:
0=ΔU+0
This implies:
ΔU=0
For an ideal gas, the change in internal energy is given by:
ΔU=nCVΔT
where n is the number of moles, CV is the molar heat capacity at constant volume, and ΔT is the change in temperature.
Since ΔU=0, it follows that:
nCVΔT=0
Given that n and CV cannot be zero, we conclude:
ΔT=0
Hint: Recall that for an ideal gas, internal energy is a function of temperature.
Step 6: Conclude the Final Temperature
Since the change in temperature is zero, the final temperature of the gas after expansion remains the same as the initial temperature:
Tfinal=Tinitial=300K
Hint: Understand that the temperature of an ideal gas does not change in free expansion.
Final Answer
The temperature of the gas after it expands to occupy the whole volume is 300 K.
We have a volume of 1 m³ divided into two compartments. One compartment contains an ideal gas at a temperature of 300 K, and the other compartment is a vacuum. The system is thermally isolated from its surroundings.
When the partition is removed, the gas expands to fill the entire volume. This process is known as free expansion, where the gas expands into a vacuum without doing any work on the surroundings.
According to the first law of thermodynamics, the change in internal energy (ΔU) is related to heat added to the system (ΔQ) and work done by the system (ΔW) by the equation:
ΔQ=ΔU+ΔW
ΔQ=0
In free expansion, the gas does not perform any work because it expands into a vacuum (no external pressure to work against):
ΔW=0
From the first law, we have:
0=ΔU+0
This implies:
ΔU=0
ΔU=nCVΔT
where n is the number of moles, CV is the molar heat capacity at constant volume, and ΔT is the change in temperature.
nCVΔT=0
ΔT=0
Since the change in temperature is zero, the final temperature of the gas after expansion remains the same as the initial temperature:
Tfinal=Tinitial=300K
The temperature of the gas after it expands to occupy the whole volume is 300 K.
Topper's Solved these Questions
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Multiple Corrects
29 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Assertion-Reasoning
6 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Subjective
22 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Single correct anwer type
14 Videos
Similar Questions
A container of volume 1m3 is divided into two equal parts by a partition. One part has an ideal gas at 300K and the other part is vacuum. The whole system is thermally isolated from the surroundings. When the partition is removed, the gas expands to occupy the whole volume. Its temperature will now be .......
View SolutionA container of volume 1m3 is divided into two equal parts by a partition. One part has an ideal gas at 300K and the other part is vacuum. The whole system is thermally isolated from the surroundings. When the partition is removed, the gas expands to occupy the whole volume. Its temperature will now be .......
View SolutionA container of volume V is divided into two equal parts by a screen. One part has an ideal gas at 300K and the other part is vacuum. The whole system is thermally isolated from the surroundings. When the screen is removed, the gas expands to occupy the whole volume. Its temperature will now be .......
View SolutionA rigid and insulated tank of 3m3 volume is divided into two compartments. One compartment of volume of 2m3 contains an ideal gas at 0.8314 Mpa and 400 K while the second compartment of volume of 1m3 contains the same gas at 8.314 Mpa and 500 K. If the partition between the two compartments is rptured, the final temperature of the gas is :
View SolutionA vessel of volume V is divided into two parts by a partition. The pressure of the gas in one part is p1 and that in the other part is p2. If the partition is removed find the pressure of the gas in the vessel.
View SolutionA container of volume 2L is seperated into equal compartments. In one compartment, one mole of an ideal monoatomic gas is filled at 1 bar pressure and the other compartment is completely evacuted. A pinhole is made in the seperator so gas expands to occupy full 2 L and heat is supplied to gas so that finally pressure of gas equals 1 bar. Then :
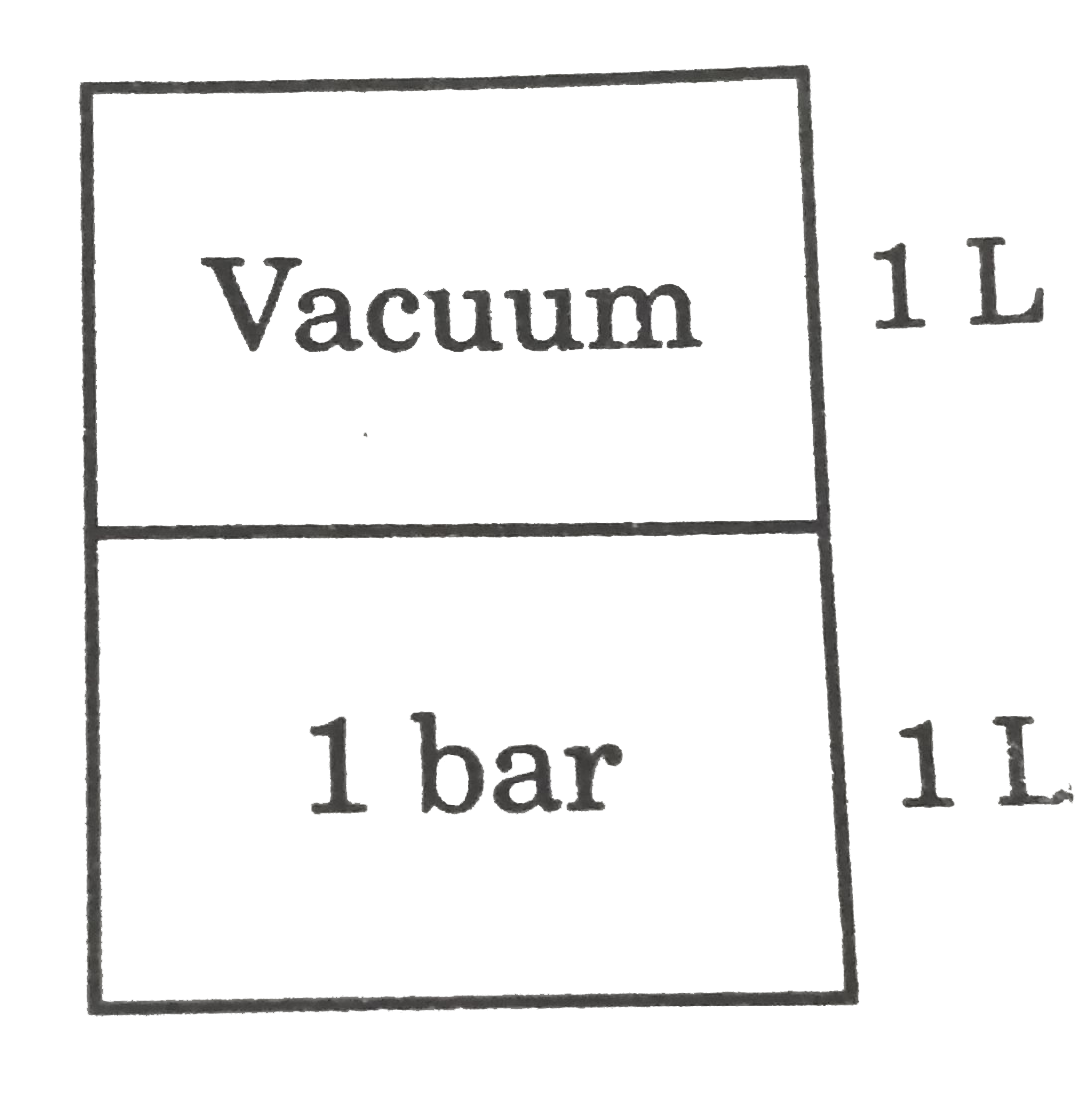
View SolutionA masslesss piston divides a closed thermallyy insulated cylinder into two equal parts. One part contains M = 28 g of nitrogen. At this temperature, one-third of molecules are dissociated into atoms and the other part is evacuated. The piston is released and the gas fills the whole volume of the cylinder at temperature T0. Then, the piston is slowly displaced back to its initial position. calculate the increases in internal energy of the gas. Neglect further dissociation of molecules during, the motion of the piston.
View SolutionA thermally insulated container is divided into two parts by a screen. In one part the pressure and temperature are P and T for an ideal gas filled. In the second part it is vacuum. If now a small hole is created in the screen, then the temperature of the gas will
View SolutionA container with insulating walls is divided into two equal parts by a partition fitted with a valve. One part is filled with an ideal gas at a pressure P and temperature T, whereas the other part is completely evacuated . If the valve is suddenly opened, the pressure and temperature of the gas will be
View SolutionA container with insulating walls is divided into two equal parts by a partition fitted with a valve. One part is filled with an ideal gas at a pressure P and temperature T, whereas the other part is completely evacuated . If the valve is suddenly opened, the pressure and temperature of the gas will be
View Solution
