A cylinder of ideal gas is closed by an 8kg movable piston of area 60cm2. The atmospheric pressure is 100kPa. When the gas is heated form 30∘C to 100∘C, the piston rises 20 cm. The piston is then fastened in the place and the gas is cooled back to 30∘C. If ΔQ1 is the heat added to the gas during heating and ΔQ2 is the heat lost during cooling, find the difference.
(a)zero
(b)136J
(c)−136J
(d)−68J
The correct Answer is:B
To solve the problem, we need to analyze the heating and cooling process of the ideal gas in the cylinder with a movable piston. We will calculate the heat added to the gas during heating (ΔQ1) and the heat lost during cooling (ΔQ2), and then find the difference between these two quantities.
1. Calculate the Total Pressure on the Gas:
The total pressure (P) on the gas is the sum of the atmospheric pressure and the pressure due to the weight of the piston.
P=Patm+mgA
Where:
- Patm=100kPa=100×103Pa
- m=8kg (mass of the piston)
- g=9.8m/s2 (acceleration due to gravity)
- A=60cm2=60×10−4m2 (area of the piston)
Calculate the pressure due to the weight of the piston:
Ppiston=mgA=8×9.860×10−4=78.40.006=13067Pa=13.067kPa
Now, calculate the total pressure:
P=100×103+13.067×103=113067Pa=113.067kPa
2. Calculate the Change in Volume (ΔV):
The piston rises by 20 cm, which is:
ΔV=A×h=60×10−4m2×0.2m=12×10−4m3=0.0012m3
3. Calculate the Work Done (W) during Heating:
The work done by the gas when the piston moves is given by:
W=PΔV=113067Pa×0.0012m3=135.68J
4. Calculate the Change in Internal Energy (ΔU) during Heating:
The change in internal energy for an ideal gas can be calculated using:
ΔU=nCvΔT
Where:
- Cv is the specific heat at constant volume.
- ΔT=(100−30)°C=70°C=70K
We need the number of moles (n) of the gas, which can be calculated using the ideal gas law:
PV=nRT
Rearranging gives:
n=PVRT
Assuming R=8.314J/(mol K) and using an average temperature of 65°C=338.15K:
n=113067×0.00128.314×338.15=135.682814.67≈0.048mol
Now, we can calculate ΔU:
ΔU=nCvΔT(assuming Cv for a diatomic gas is about 5R/2)
Cv≈5×8.314/2=20.785J/(mol K)
ΔU=0.048×20.785×70≈70.0J
5. Calculate the Heat Added (ΔQ1):
Using the first law of thermodynamics:
ΔQ1=ΔU+W=70.0J+135.68J≈205.68J
6. Calculate the Heat Lost (ΔQ2) during Cooling:
When the gas is cooled back to 30°C, the process is at constant volume, so:
ΔQ2=ΔU=nCvΔT=0.048×20.785×(−70)≈−70.0J
7. Find the Difference (ΔQ1 - ΔQ2):
ΔQ=ΔQ1−ΔQ2=205.68−(−70.0)=205.68+70.0=275.68J
Final Answer:
The difference in heat added and heat lost is approximately 275.68 J.
The total pressure (P) on the gas is the sum of the atmospheric pressure and the pressure due to the weight of the piston.
P=Patm+mgA
Where:
- Patm=100kPa=100×103Pa
- m=8kg (mass of the piston)
- g=9.8m/s2 (acceleration due to gravity)
- A=60cm2=60×10−4m2 (area of the piston)
Ppiston=mgA=8×9.860×10−4=78.40.006=13067Pa=13.067kPa
P=100×103+13.067×103=113067Pa=113.067kPa
The piston rises by 20 cm, which is:
ΔV=A×h=60×10−4m2×0.2m=12×10−4m3=0.0012m3
The work done by the gas when the piston moves is given by:
W=PΔV=113067Pa×0.0012m3=135.68J
The change in internal energy for an ideal gas can be calculated using:
ΔU=nCvΔT
Where:
- Cv is the specific heat at constant volume.
- ΔT=(100−30)°C=70°C=70K
PV=nRT
Rearranging gives:
n=PVRT
Assuming R=8.314J/(mol K) and using an average temperature of 65°C=338.15K:
n=113067×0.00128.314×338.15=135.682814.67≈0.048mol
ΔU=nCvΔT(assuming Cv for a diatomic gas is about 5R/2)
Cv≈5×8.314/2=20.785J/(mol K)
ΔU=0.048×20.785×70≈70.0J
Using the first law of thermodynamics:
ΔQ1=ΔU+W=70.0J+135.68J≈205.68J
When the gas is cooled back to 30°C, the process is at constant volume, so:
ΔQ2=ΔU=nCvΔT=0.048×20.785×(−70)≈−70.0J
ΔQ=ΔQ1−ΔQ2=205.68−(−70.0)=205.68+70.0=275.68J
The difference in heat added and heat lost is approximately 275.68 J.
Topper's Solved these Questions
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Multiple Corrects
Explore 29 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Assertion-Reasoning
Explore 6 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Subjective
Explore 22 Videos
- KINETIC THEORY OF GASESBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASESExercise:Compression
Explore 2 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBook:CENGAGE PHYSICS ENGLISHChapter:LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONExercise:Single correct anwer type
Explore 14 Videos
Similar Questions
A vertical hollow cylinder contains an ideal gas. The gas is enclosed by a 5kg movable piston with an area of cross-section 5×10−3m2. Now, the gas is heated slowly from 300K to 350K and the piston rises by 0.1m. The piston is now clamped at this position and the gas is cooled back to 300K. Fid the difference between the heat energy added during heating process and energy lost during the cooling process. (1atm pressire=105Nm−2]
View SolutionIf an ideal gas is heated at constant pressure :
View SolutionFive moles of hydrogen gas are heated from 30∘C to 60∘C at constant pressure. Heat given to the gas is (given R=2cal/mol degrees)
View SolutionFive moles of hydrogen gas are heated from 30∘C to 60∘C at constant pressure. Heat given to the gas is (given R=2cal/mol degrees)
View SolutionA movable piston separates a sample of gas from vacuum. In this state, pressure of gas is P, then find pressure after piston is released
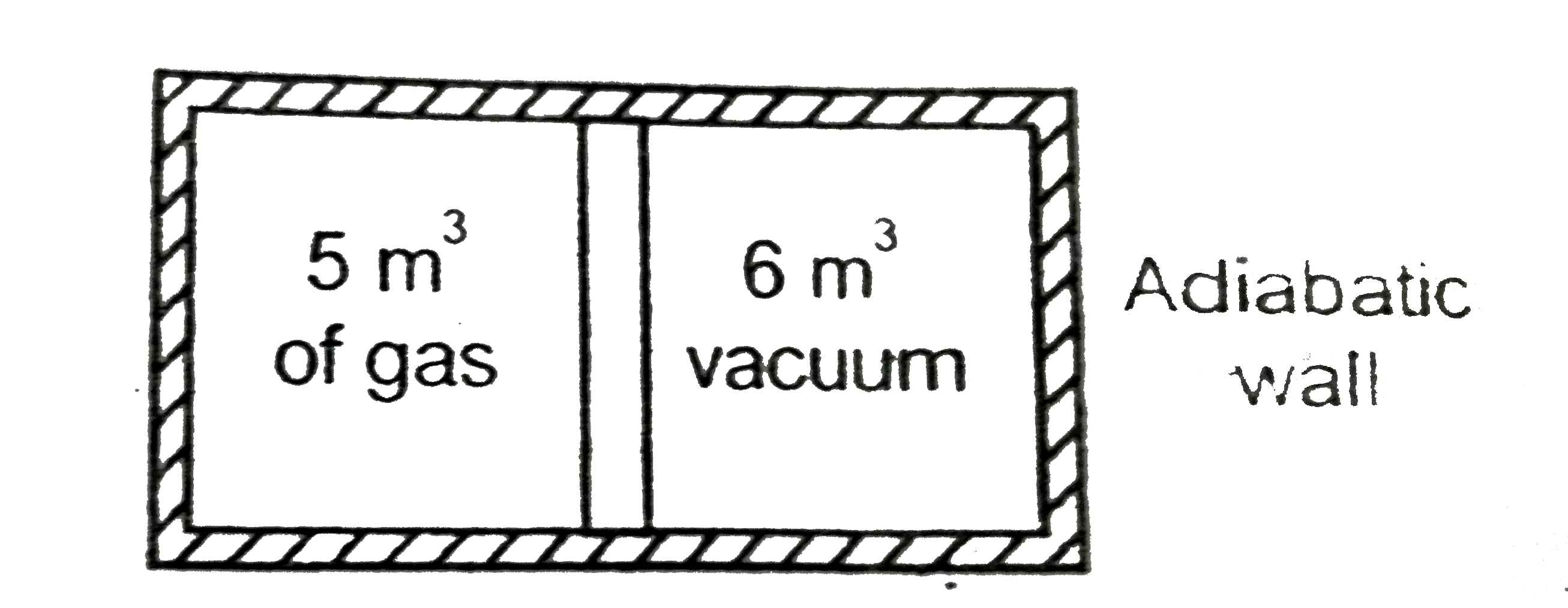
View SolutionA gas is contained in a metallic cylinder fitted with a piston.The piston is suddenly moved in to compress the gas and is maintained at this position. As time passes the pressure of the gas in the cylinder
View SolutionA gas is contained in a metallic cylinder fitted with a piston.The piston is suddenly moved in to compress the gas and is maintained at this position. As time passes the pressure of the gas in the cylinder
View SolutionWhen an ideal gas is heated at constant pressure, the fraction of the heat energy supplied whicn increases the internal energy of the gas is :
View Solution2.00 mole of a monatomic ideal gas (U=1.5nRT) is enclosed in an adiabatic, fixed , vertical cylinder fitted with a smooth, light adiabatic piston. The piston is connected to a vertical spring of spring constant 200Nm−1 as shown in .the area of cross section of the cylinder is 20.0cm2. initially, the spring is at its natural length and the temperature of the gas is 300K at its natural length. the atmosphere pressure is 100 kPa. the gas is heated slowly for some time by means of an electric heater so as to move the position up through 10 cm. find (a) the work done by the gas (b) the final temperature of the gas and (c ) the heat supplied by the heater.
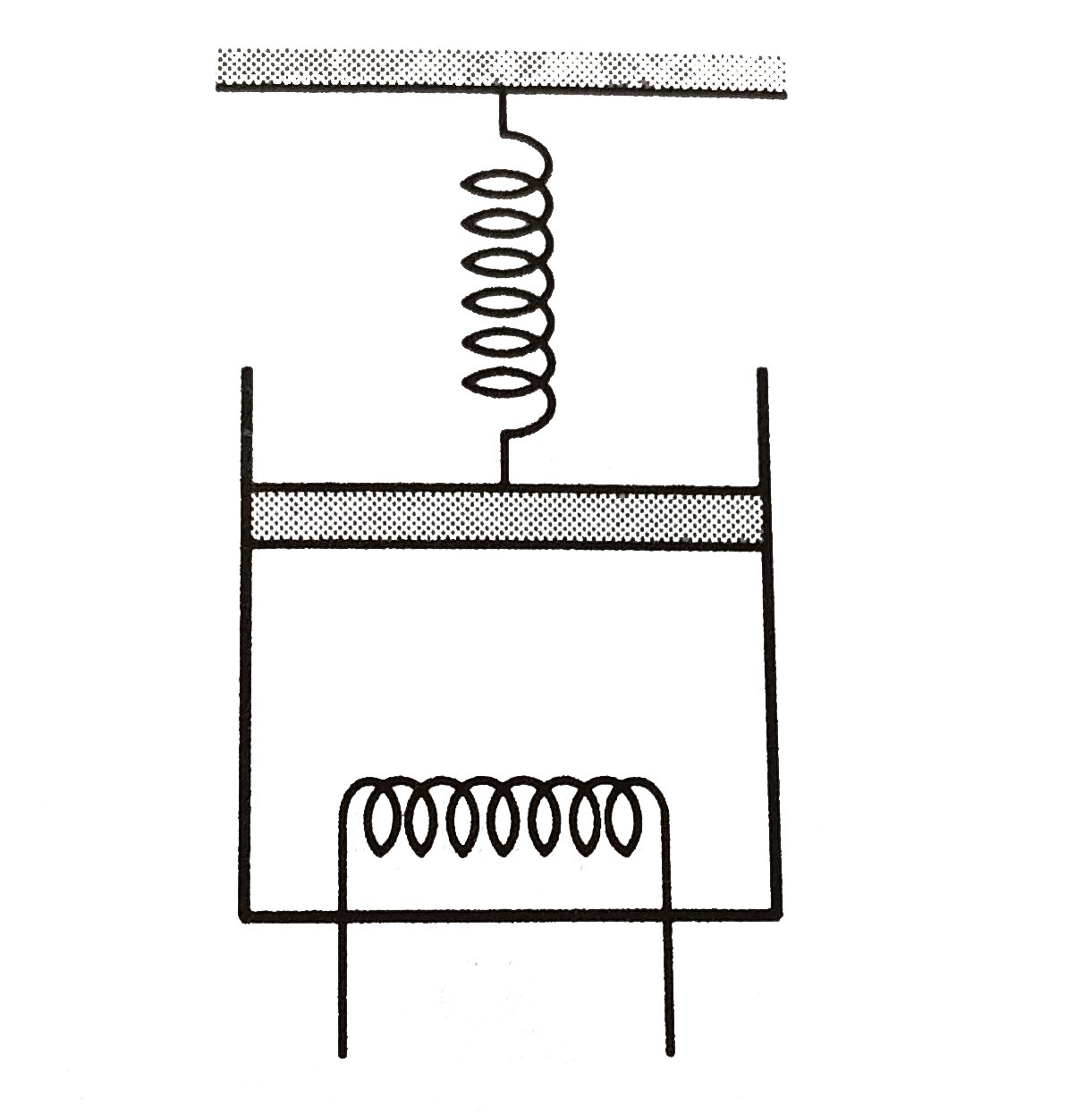
View SolutionA gas is contained in a metallic cylinder fitted with a piston.the piston is suddenly moved in to compress the gas and is maintained at this position. As time passes the pressure of the gas in the cylinder
View Solution
