Question

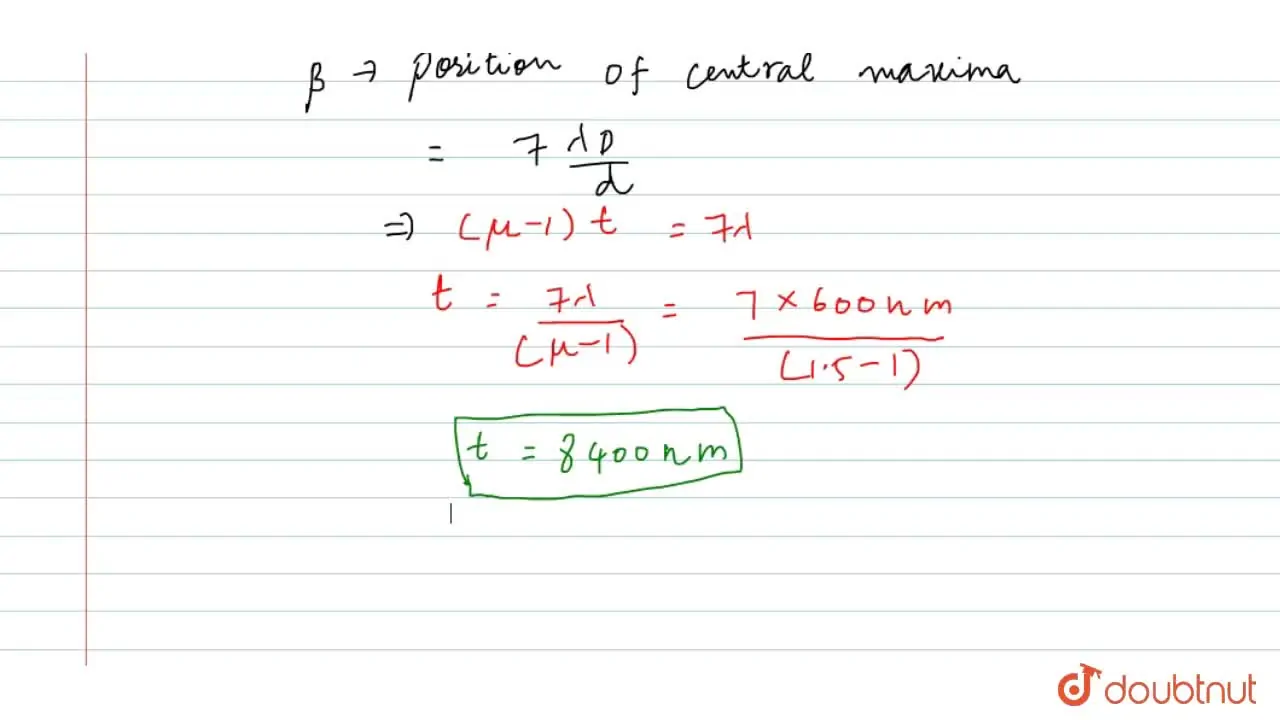
A flake of glass (refractive index 1.5) is placed over one of the opening of a double-slit apparatus. The interference pattern displaced itself through seven successive maxima toward the side where the flake is placed. If wavelength of the light is $\lambda =600nm$, then the thickness of the flake is

Options

A. 2100 nm
B. 4200 nm
C. 8400 nm
D. none of above

Answer

c

Explanation

To find the thickness of the glass flake placed over one of the openings of a double-slit apparatus, we can follow these steps:

1. Understand the Problem:
We have a double-slit experiment where a glass flake with a refractive index (μ) of 1.5 is placed over one of the slits. The interference pattern shifts by 7 maxima towards the side where the flake is placed.

2. Identify the Relevant Formula:
The shift in the interference pattern can be expressed as:
\( \Delta y = (μ - 1) \frac{t}{d} \)
where:
- \(\Delta y\) is the shift in the position of the maxima,
- \(μ\) is the refractive index of the glass,
- \(t\) is the thickness of the glass flake,
- \(d\) is the distance between the slits.

In this case, since the shift corresponds to 7 maxima, we can express it as:
\( \Delta y = 7\beta \)
where \(\beta\) is the fringe width.

3. Calculate the Fringe Width (\(\beta\)):
The fringe width (\(\beta\)) is given by:
\( \beta = \frac{\lambda D}{d} \)
where \(D\) is the distance from the slits to the screen and \(\lambda\) is the wavelength of the light used (600 nm in this case).

4. Substitute the Shift:
We can now relate the shift to the thickness of the flake:
\( 7\beta = (μ - 1) t \)
Rearranging gives:
\( t = \frac{7\beta}{μ - 1} \)

5. Substitute \(\beta\):
Now we substitute \(\beta\) into the equation:
\( t = \frac{7 \left(\frac{\lambda D}{d}\right)}{μ - 1} \)

6. Substitute Known Values:
We know:
- \(\lambda = 600 \text{ nm} = 600 \times 10^{-9} \text{ m}\)
- \(μ = 1.5\)
Therefore:
\( μ - 1 = 1.5 - 1 = 0.5 \)
Now substituting into the equation for \(t\):
\( t = \frac{7 \cdot \lambda}{μ - 1} = \frac{7 \cdot 600 \times 10^{-9}}{0.5} \)

7. Calculate Thickness:
\( t = \frac{4200 \times 10^{-9}}{0.5} = 8400 \times 10^{-9} \text{ m} = 8400 \text{ nm} \)

Final Answer:
The thickness of the glass flake is \(8400 \text{ nm}\).

---