A light of wavelength 6000Å shines on two narrow slits separeted by a distance 1.0 mm and illuminates a screen at a distance 1.5 m away. When one slit is covered by a thin glass plate of refractive index 1.8 and other slit by a thin glass plate of refractive index μ, the central maxima shifts by 0.1 rad. Both plates have the same thickness of 0.5 mm. The value of refractive index μ of the glass is
1.4
1.5
1.6
none of these
The correct Answer is:c
To solve the problem step-by-step, we will follow these steps:
Step 1: Understand the Problem
We have two slits illuminated by light of wavelength λ=6000Å=6000×10−10m. The distance between the slits is d=1.0mm=1.0×10−3m and the distance to the screen is D=1.5m. One slit is covered with a glass plate of refractive index μ1=1.8 and the other with a glass plate of refractive index μ2. The thickness of both plates is t=0.5mm=0.5×10−3m. The central maximum shifts by an angle of θ=0.1rad.
Step 2: Calculate the Shift in Position
The shift in position Δx on the screen due to the angle θ can be calculated using the formula:
Δx=Dtan(θ)
For small angles, tan(θ)≈θ, so:
Δx≈Dθ=1.5×0.1=0.15m
Step 3: Calculate the Optical Path Difference
The optical path difference caused by the glass plates can be expressed as:
Δx=(μ2−μ1)tDd
Where μ1=1.8 and μ2 is the unknown refractive index.
Step 4: Set Up the Equation
From the previous steps, we can equate the shifts:
0.15=(μ2−1.8)(0.5×10−3)1.51.0×10−3
Step 5: Simplify the Equation
Now, simplifying the equation:
0.15=(μ2−1.8)(0.5×10−3)⋅1500
0.15=(μ2−1.8)⋅0.75
μ2−1.8=0.150.75=0.2
μ2=1.8+0.2=2.0
Step 6: Check for Validity
Since the problem states that the central maxima shifts by 0.1rad, we need to check if this value of μ2 is valid.
Step 7: Final Calculation
If we take the negative shift (as the shift could be in either direction):
μ2−1.8=−0.2⟹μ2=1.8−0.2=1.6
Conclusion
Thus, the value of the refractive index μ is:
μ=1.6
We have two slits illuminated by light of wavelength λ=6000Å=6000×10−10m. The distance between the slits is d=1.0mm=1.0×10−3m and the distance to the screen is D=1.5m. One slit is covered with a glass plate of refractive index μ1=1.8 and the other with a glass plate of refractive index μ2. The thickness of both plates is t=0.5mm=0.5×10−3m. The central maximum shifts by an angle of θ=0.1rad.
The shift in position Δx on the screen due to the angle θ can be calculated using the formula:
Δx=Dtan(θ)
For small angles, tan(θ)≈θ, so:
Δx≈Dθ=1.5×0.1=0.15m
The optical path difference caused by the glass plates can be expressed as:
Δx=(μ2−μ1)tDd
Where μ1=1.8 and μ2 is the unknown refractive index.
From the previous steps, we can equate the shifts:
0.15=(μ2−1.8)(0.5×10−3)1.51.0×10−3
Now, simplifying the equation:
0.15=(μ2−1.8)(0.5×10−3)⋅1500
0.15=(μ2−1.8)⋅0.75
μ2−1.8=0.150.75=0.2
μ2=1.8+0.2=2.0
Since the problem states that the central maxima shifts by 0.1rad, we need to check if this value of μ2 is valid.
If we take the negative shift (as the shift could be in either direction):
μ2−1.8=−0.2⟹μ2=1.8−0.2=1.6
Thus, the value of the refractive index μ is:
μ=1.6
Topper's Solved these Questions
- WAVE OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:WAVE OPTICSExercise:Multiple Correct
Explore 8 Videos
- WAVE OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:WAVE OPTICSExercise:Assertion- Reasoning
Explore 13 Videos
- WAVE OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:WAVE OPTICSExercise:Subjective
Explore 11 Videos
- SOURCES OF MAGNETIC FIELDBook:CENGAGE PHYSICS ENGLISHChapter:SOURCES OF MAGNETIC FIELDExercise:single correct Ansewer type
Explore 12 Videos
Similar Questions
Light of wavelength 5000 Å is incident on a slit of width 0.1 mm. Find out the width of the central bright line on a screen distance 2m from the slit?
View SolutionLight of wavelength 6000Å is incident on a thin glass plate of refractive index 1.5 such that angle of refraction into the plate is 60∘. Calculate the smallest thickness of plate which will make it appear dark by reflection.
View SolutionIn a young 's double slit experiment, a glass plate of refractive index 1.5 and thickness 5×10−4 cm is kept in the path of one of the light rays. Then
View SolutionIn a Young.s double slit experiment, the fringes are displaced by a distance x when a glass plate of refractive index 1.5 is introduced in the path of one of the beams. When this plate is replaced by another plate of same thickness, the shift of fringes is (3/2)x. The refractive index of second plate is
View SolutionIn a Young's double slit experiment, the light sources is at distance l1=20μm and l2=40μm form the main slit. The light of wavelength lambda = 500nm is incident on slits separated at a distance 10μm. A screen is placed at a distance D = 2 away from the slits as shown in figure. Find
(a) the values of θ relative to the central line where maxima appear on the screen?
(b) how many maxima will appear on the screen?
what should be the minimum thickness of a slab of refractive index 1.5 placed on the path of one of the ray so that minima occurs at C ?
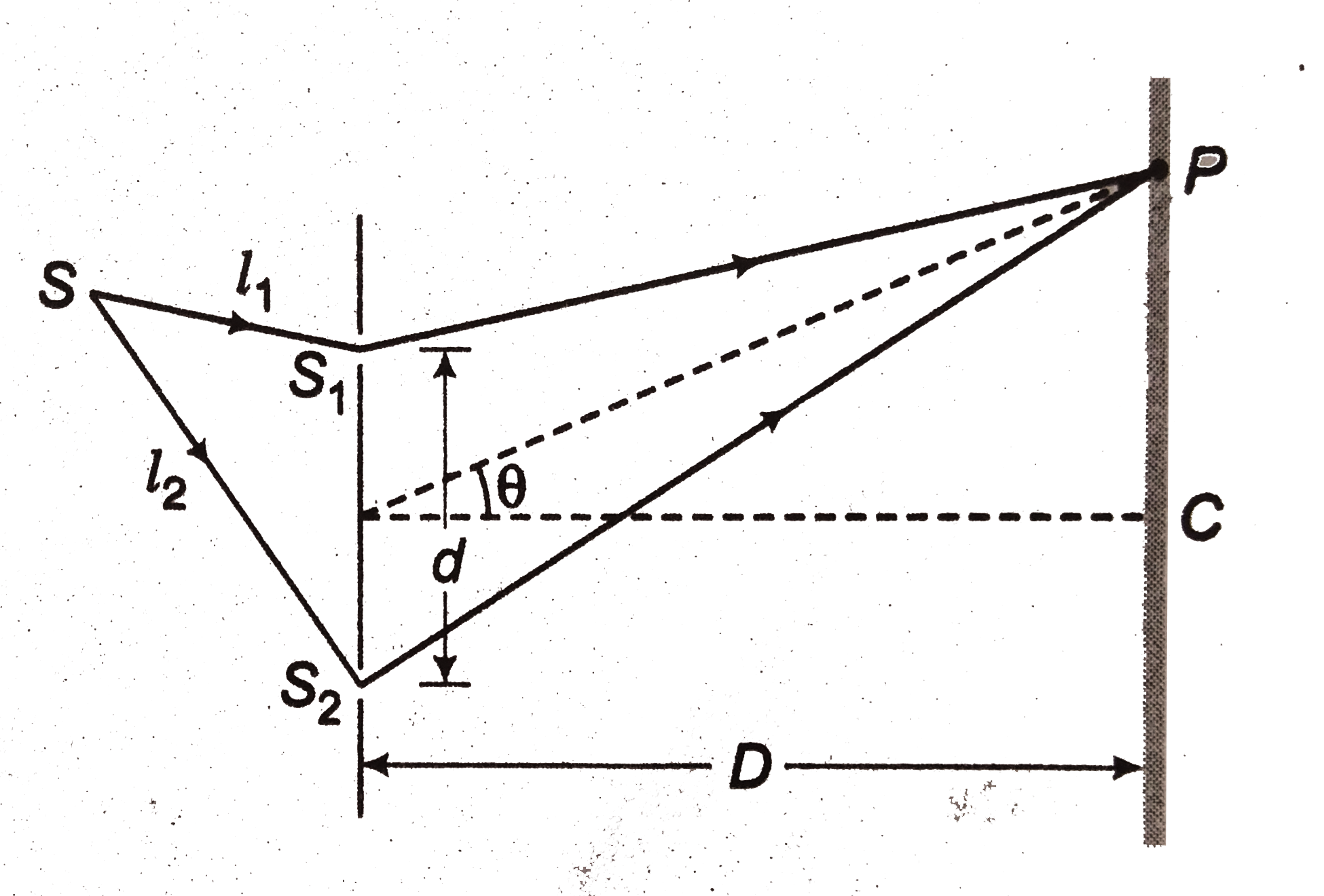 .
.
View SolutionIn a double-slit experiment, fringes are produced using light of wavelength 4800A∘. One slit is covered by a thin plate of glass of refractive index 1.4 and the other slit by another plate of glass of double thickness and of refractive index 1.7. On doing so, the central bright fringe shifts to a position originally occupied by the fifth bright fringe from the center. find the thickness of the glass plates.
View SolutionLight of wavelength 6000A is incident on a thin glass plate of refractive index 0.5 such that angle of refraction into plate is 60° .calculate the thickness of plate which will make it appear dark by reflection?
View SolutionA monochromatic light of λ=5000Å is incident on two identical slits separated by a distance of 5×10−4 m. The interference pattern is seen on a screen placed at a distance of 1 m from the plane of slits. A thin glass plate of thickness 1.5×10−6 m and refractive index μ=1.5 is placed between one of the slits and the screen . Find the intensity at the center of the screen.
View SolutionA monochromatic light of λ=500nm is incident on two identical slits separated by a distance of 5×10−4m. The interference pattern is seen on a screen placed at a distance of 1m from the plane of slits. A thin glass plate of thickness 1.5×10−6m and refractive index μ=1.5 is placed between one of the slits and the screen. Find the intensity at the center of the screen if the intensity is I0 in the absence of the plate. Also find the lateral shift of the central maxima and number of fringes crossed through center.
View SolutionLight of wavelength 3000A is incident on a thin glass plate of refractive index 0.5 such that angle of refraction into plate is 60° .calculate the thickness of plate which will make it appear dark by reflection?
View Solution