In Figure. , the length of object AB is 9cm. Find the nature and position of the final image and also its length. Assum that each lens is a thin lens.
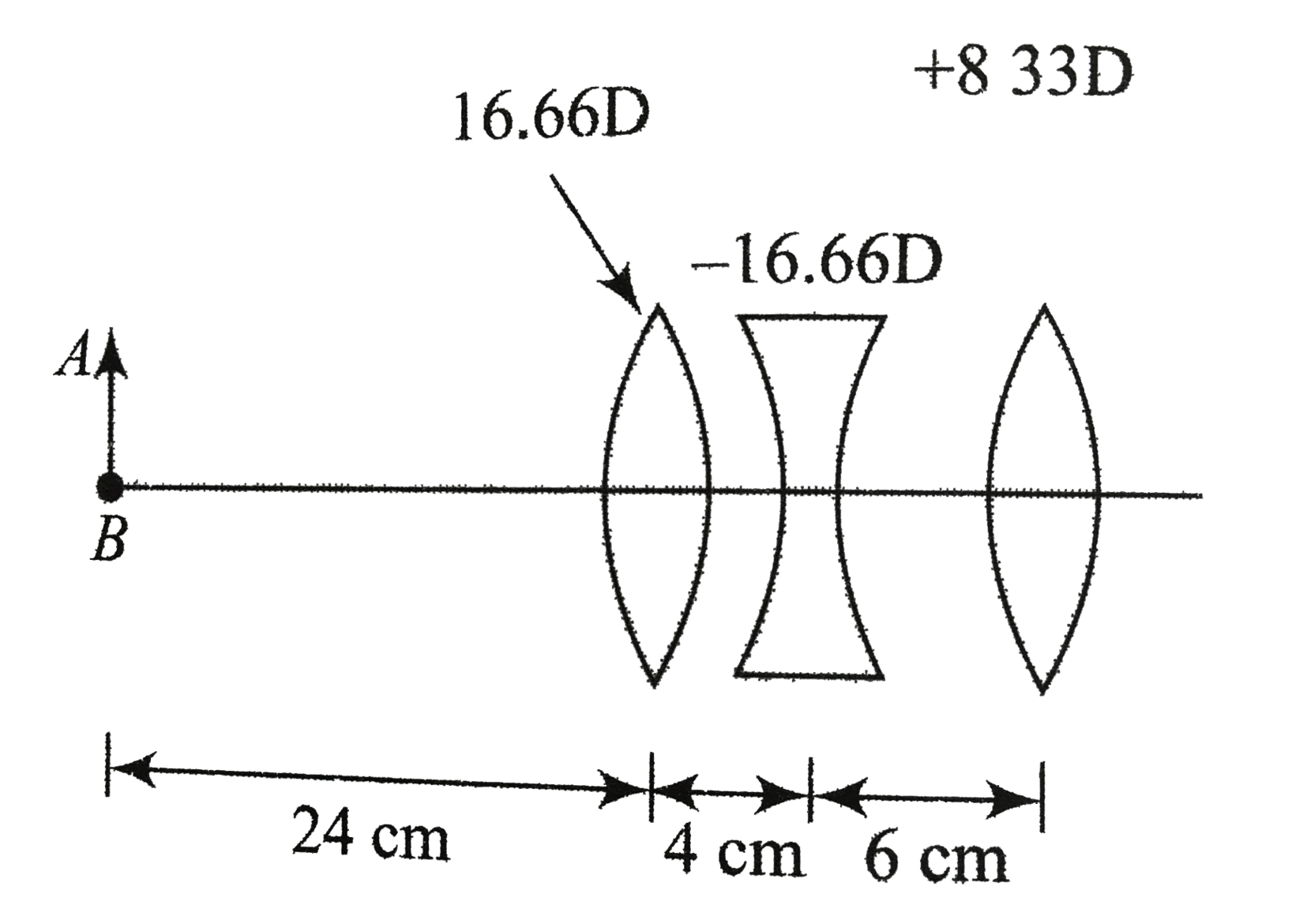
Action of the first lens:
Focal length =116.66=0.06m=6cm
u=−24cm
Using 1v−1u=1f
1v+124=16
1v=16−124orv=8cm
This image would be real and inverted (with respect to object AB).
Its magnification, m1=vu=8−24=−13
Action of the second lens:
Image formed by the first lens, being 8cm to the right of the first lens, would at as virtual object for the second lens.
Focal length=1−16.66=−0.006m=−6cm
u=+4cm [ distance of image formed by the first lens from the second lens ]
1v−14=−16
1v=14−16=112orv=12cm
Thus, the second lens forms an image 12cm to its right. This image is real and its magnification,
m2=vu=124=3
Thus, it is also erect relative to the image formed by the first lens.
Action of the third lens:
Image formed by the second lens, being 12 cm to its right, will act as a virtual object for the third lens.
Focal length =18.33=0.12m=12cm
u=+6cm [ distance of image formed by the second lens from the third lens ]
1v−16=112
1v=112+16orv=4cm
Thus, the final image forms 4cm to the right of the third lens. The image will be real and its magnifications,
m3=vu=46=23
This image is erect relative to teh image formed by the second lens.
Total magnification m=m1×m2×m3
=−13×3×23
⇒m=−23
Hence, the final image is, relative to the given object, inverted and also reduced in size. It forms 4cm to the right of the third lens and it is a real image.
To obtain length of the final image,
m=IO=−23
I=23×9=−6cm

Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Solved Examples
15 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.1
14 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
An object is placed at a distance of 10cm to the left on the axis of convex lens A of focal length 20cm. A second convex lens of focal length 10cm is placed coaxially to the right of the lens A at a distance of 5cm from A. Find the position of the final image and its magnification. Trace the path of the rays.
View SolutionFocal length of the convex lens shown in figure is 20cm. Find the image position and image velocity.
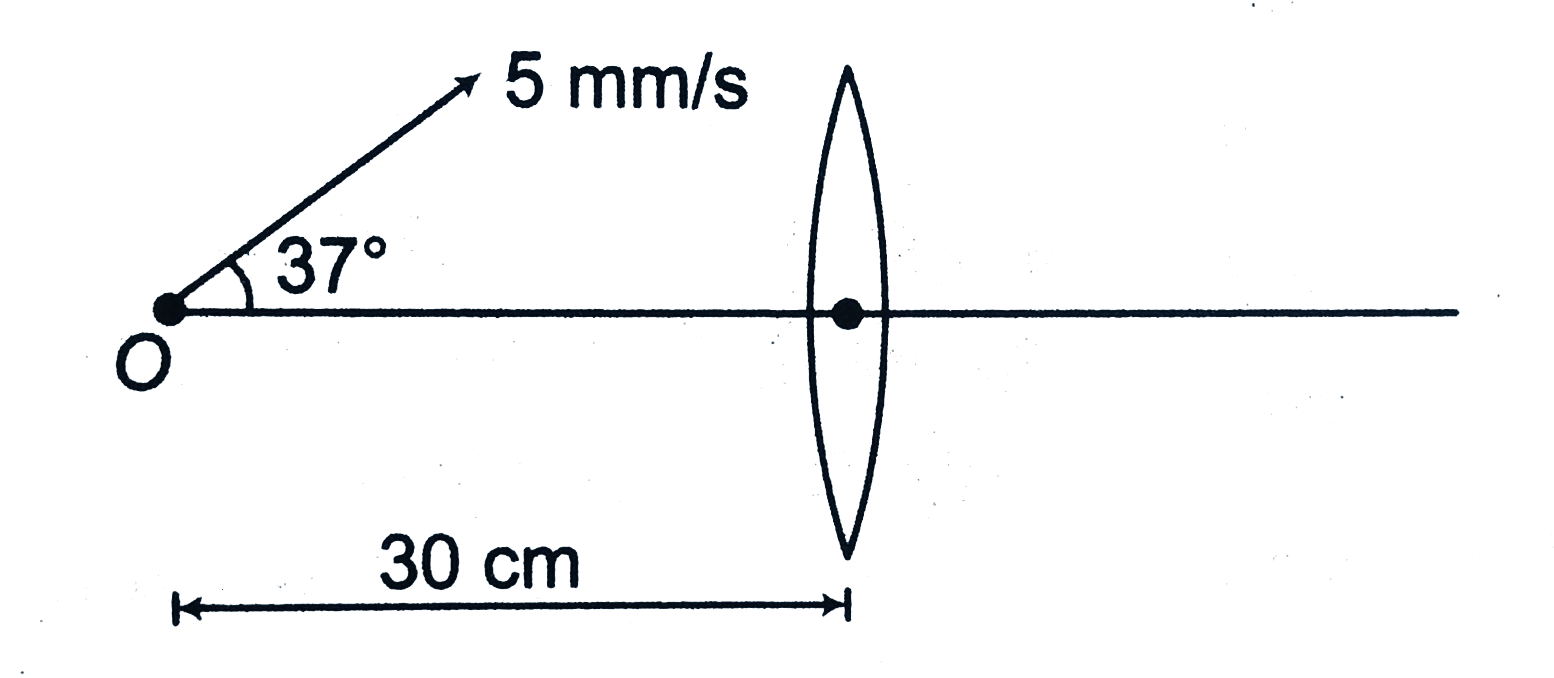
View SolutionIn a terrestrial telescope, the focal length of objective is 90cm and of eye lens is 6cm. If the final image is at 30cm, then the magnification will be
View SolutionAn object of height 2cm is kept 2m in front of a convex lens of focal length 1m. A plane mirror is placed at 3m from the lens on its other side. Find the nature, position, and magnification of the final image that will be seen by an observer looking toward the mirror through the lens.
View SolutionIf the focal length of the lens is 20 cm, find the distance of the image from the lens in the following figure?

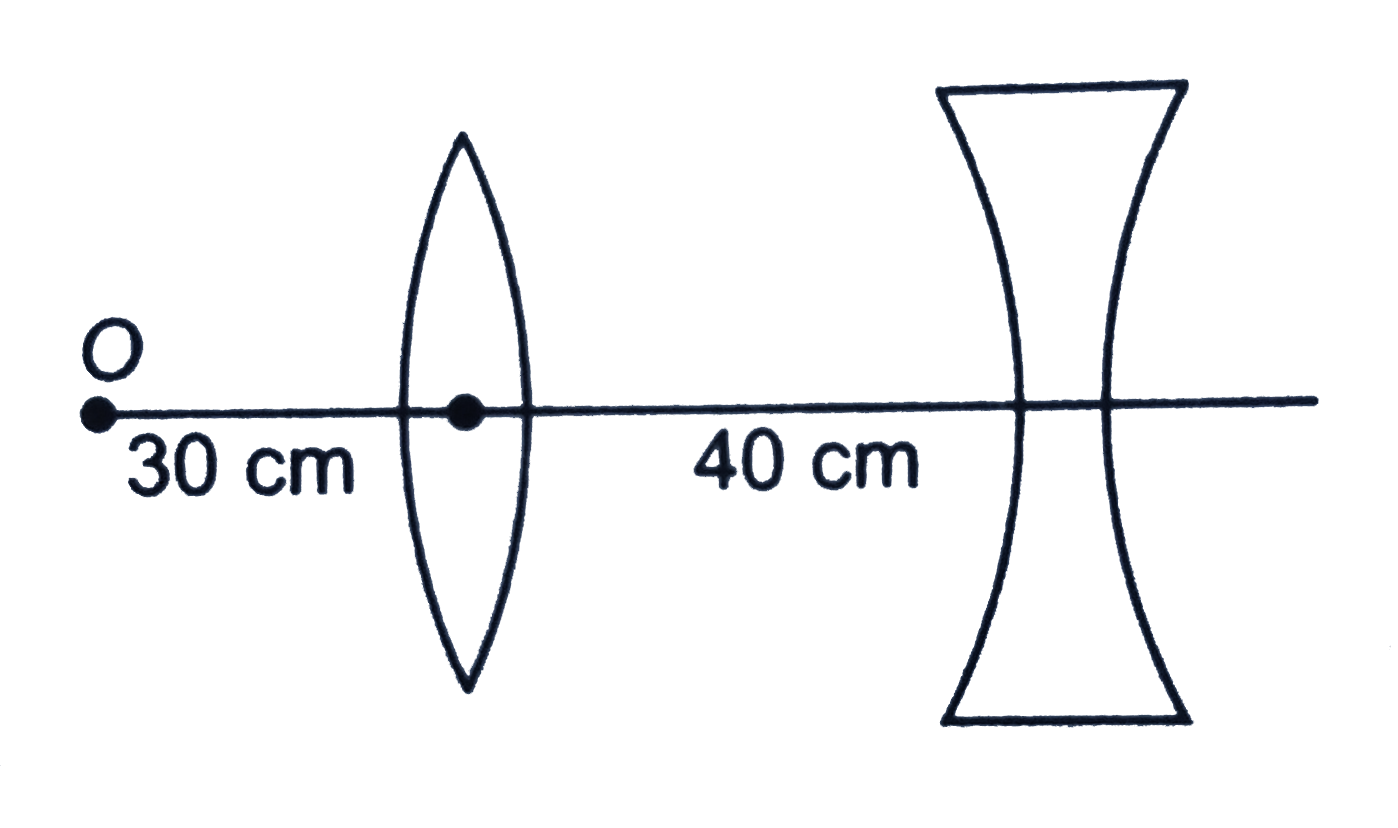
View SolutionFocal length of a convex lense in air is 20cm. and of concave lens 40cm. Find the position of final image.
View SolutionA 2.0cm tall object is placed perpendicular to the principal axis of a convex lens of focal length 10 cm. The distance of the object from the lens is 15 cm. Find the nature, position and size of the image. Also, find its magnification.
View SolutionIn a displacement method the distance between the object and the screen is 70 cm and the focal length of the lens is 16 cm, find the separations of the magnified and diminished image position of the lens.
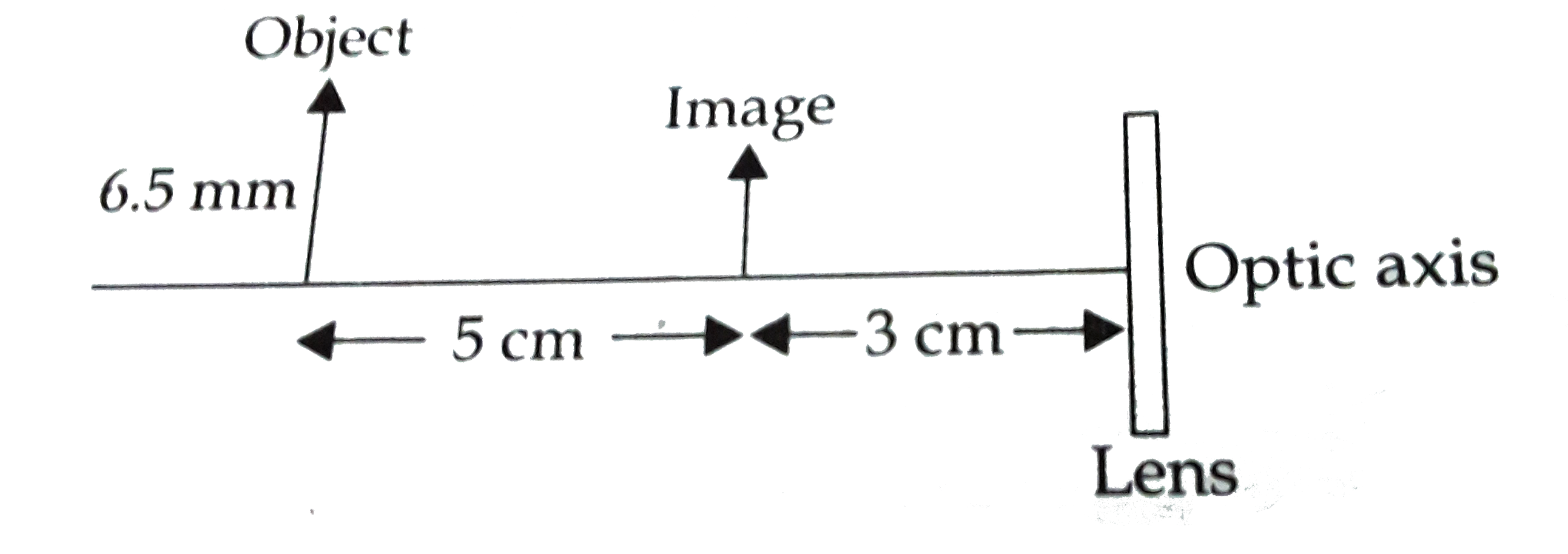
View SolutionShown an object and its image formed by a thin lens. Then the nature and focal length of lens is
View SolutionA convex lens has a focal length of 10 cm. Find the location and nature of the image if a point object is placed on the principal axis at a distance of (a) 9.8 cm, (b) 10.2 cm from the lens.
View Solution
