For a prism, A=60∘,n=√7/3 . Find the minimum possible angle of incidence, so that the light ray is refracted from the second surface. Also, find δmax.
To solve the given problem, we will follow these steps:
Step 1: Understand the Problem
We are given a prism with an angle A=60∘ and a refractive index n=√73. We need to find the minimum angle of incidence I for which the light ray is refracted from the second surface of the prism, as well as the maximum deviation δmax.
Step 2: Apply Snell's Law at the First Surface
At the first surface of the prism, we apply Snell's law:
n2sinR2=n1sin90∘
where n2=√73 (the refractive index of the prism) and n1=1 (the refractive index of air).
Since sin90∘=1, we can rewrite the equation as:
√73sinR2=1
From this, we can solve for R2:
sinR2=1√73=√3√7
Thus,
R2=sin−1(√3√7)≈40.89∘
Step 3: Find R1
Using the relationship between the angles in the prism:
A=R1+R2
we can find R1:
R1=A−R2=60∘−40.89∘=19.11∘
Step 4: Apply Snell's Law at the Second Surface
Now, we apply Snell's law at the second surface (point C):
n1sinI=n2sinR1
Substituting the known values:
1⋅sinI=√73⋅sin(19.11∘)
Calculating sin(19.11∘):
sin(19.11∘)≈0.327
Now substituting this value:
sinI=√73⋅0.327
Calculating √73≈1.527:
sinI≈1.527⋅0.327≈0.500
Thus,
I=sin−1(0.500)=30∘
Step 5: Calculate Maximum Deviation δmax
The formula for maximum deviation δmax is given by:
δmax=I+E−A
where E=90∘:
δmax=30∘+90∘−60∘=60∘
Final Answers
- Minimum angle of incidence I=30∘
- Maximum deviation δmax=60∘
---
We are given a prism with an angle A=60∘ and a refractive index n=√73. We need to find the minimum angle of incidence I for which the light ray is refracted from the second surface of the prism, as well as the maximum deviation δmax.
At the first surface of the prism, we apply Snell's law:
n2sinR2=n1sin90∘
where n2=√73 (the refractive index of the prism) and n1=1 (the refractive index of air).
√73sinR2=1
From this, we can solve for R2:
sinR2=1√73=√3√7
Thus,
R2=sin−1(√3√7)≈40.89∘
Using the relationship between the angles in the prism:
A=R1+R2
we can find R1:
R1=A−R2=60∘−40.89∘=19.11∘
Now, we apply Snell's law at the second surface (point C):
n1sinI=n2sinR1
Substituting the known values:
1⋅sinI=√73⋅sin(19.11∘)
Calculating sin(19.11∘):
sin(19.11∘)≈0.327
Now substituting this value:
sinI=√73⋅0.327
Calculating √73≈1.527:
sinI≈1.527⋅0.327≈0.500
Thus,
I=sin−1(0.500)=30∘
The formula for maximum deviation δmax is given by:
δmax=I+E−A
where E=90∘:
δmax=30∘+90∘−60∘=60∘
- Minimum angle of incidence I=30∘
- Maximum deviation δmax=60∘
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Solved Examples
15 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.1
14 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
A prism (n=2) of apex angle 90∘ is palced in air (n=1). What should be the angle of incidence so that light ray strikes the second surface at an angle of incidence 60∘.
View SolutionLight ray is incident on a prism of angle A=60∘ are refractive index μ=√2. The angle of incidence which the emergent rays grazes the surface is given
View SolutionAngle of a prism is 30∘ and its refractive index is √2 and one of the surface is silvered. At what angle of incidence, a ray should be incident on one surface so that after reflection from the silvered surface, it retraces its path ?
View SolutionRefractive index of a prism is √7/3 and the angle of prism is 60∘. The minimum angle of incidence of a ray that will be transmitted through the prism is
View SolutionA ray of light is normally incident on one face of an equilateral glass prism. Answer the following :
(a) What is the angle of incidence on the first face of the prism?
(b) What is the angle of refraction from the first face of the prism ?
(c) What will be the angle of incidence at the second face of the prism ?
(d) Will the light ray suffer minimum deviation by the prism ?
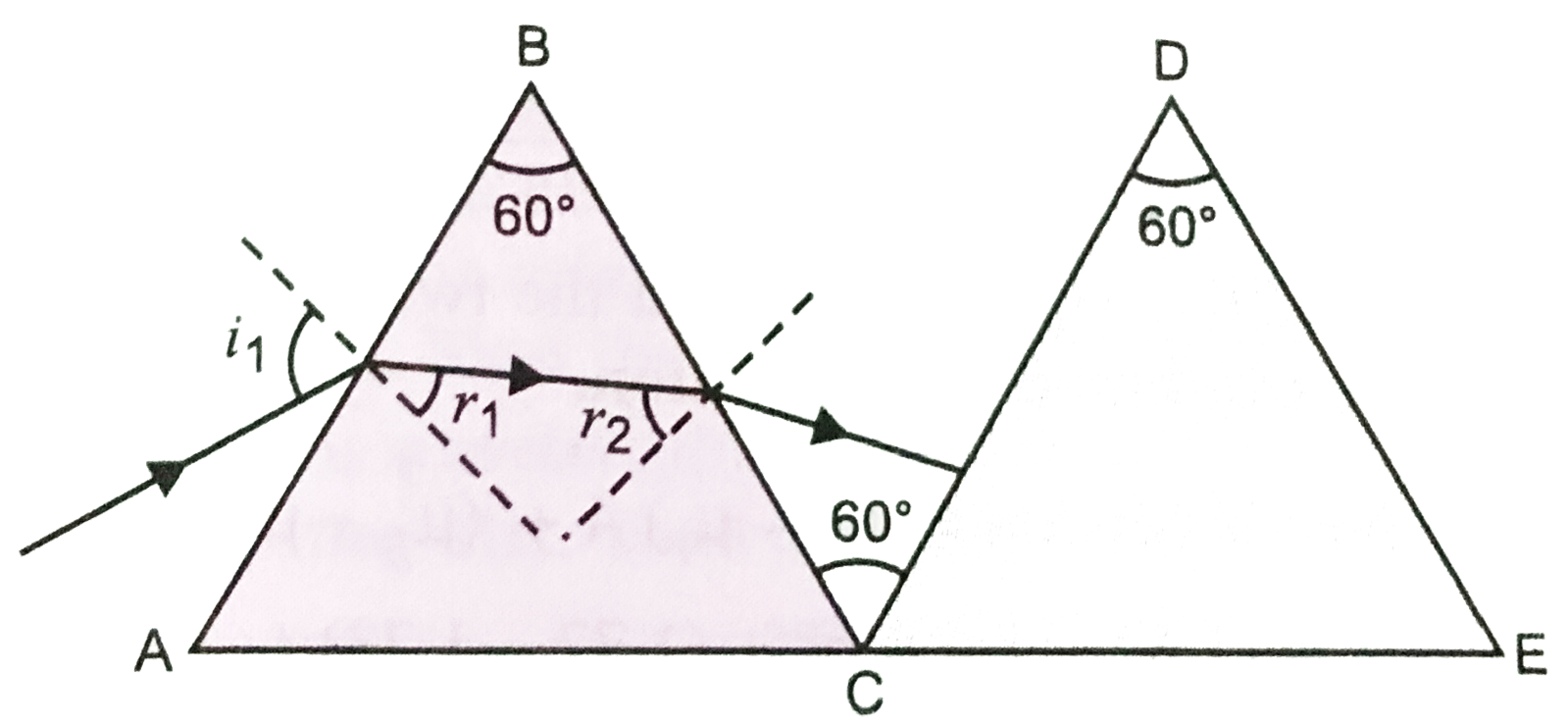
View SolutionA ray of light is incident on a prism ABC of μ=√3 as shown in Fig. Find the angle of incidence for which the deviation of light by the prism ABC is minimum.
By what angle should the second prism be rotated so that final ray suffers net minimum deviation ?
View SolutionRefractive index of a prism is √73 and the angle of prism is 60∘. The limiting angle of incidence of a ray that will be transmitted through the prism is approximately
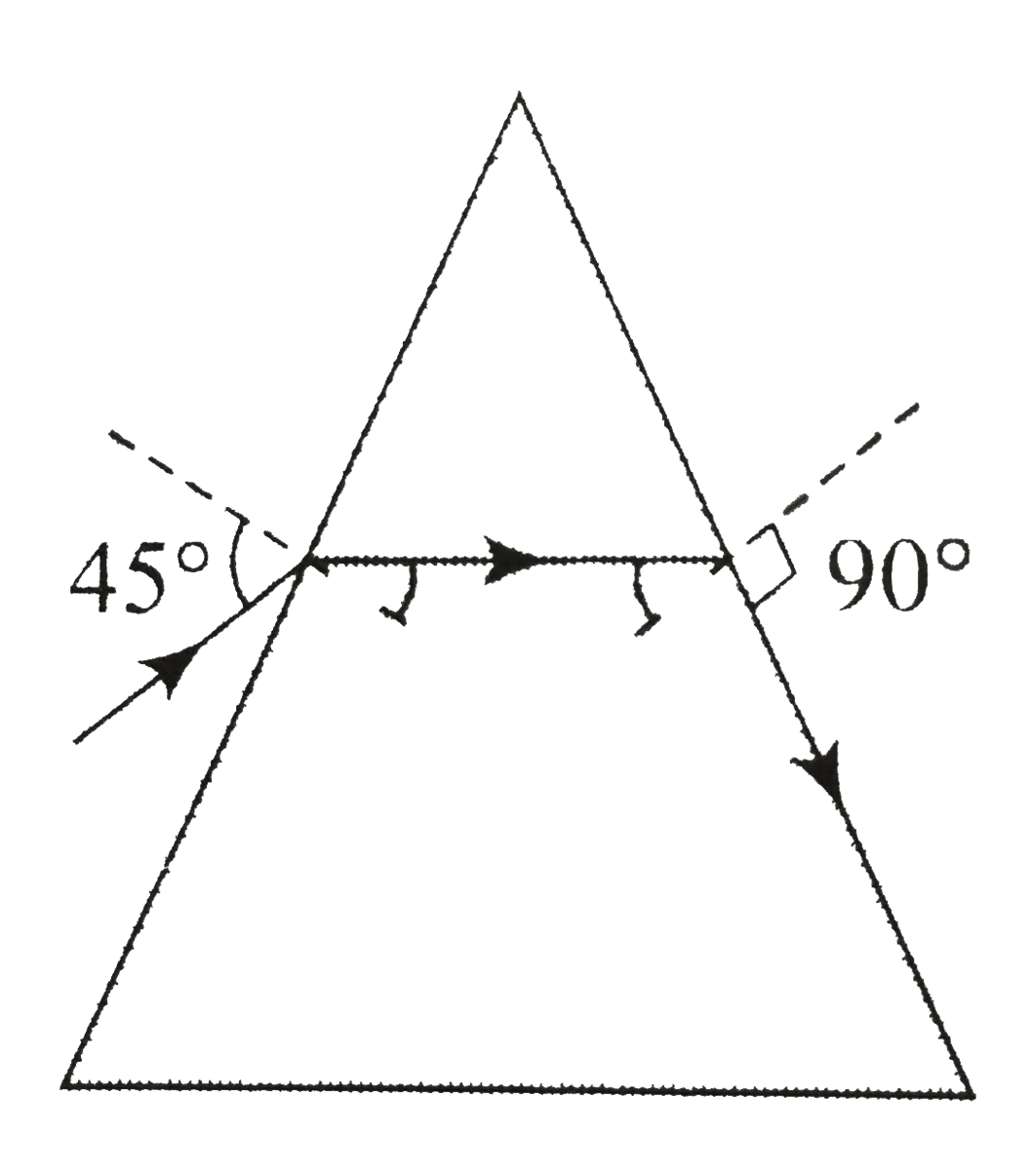
View SolutionIn an isosceles prism of angle 45∘, it is found that when the angle of incidence is same as the prism angle, the emergen ray grazes the emergent surface.
Find the refractive index of the material of the prism. For what angle of incidenc, the angle of deviation will be minimum?
View SolutionWhat is the angle of incidence for an equilateral prism of refractive index √3 so that the ray si parallel to the base inside the prism?
View SolutionThe refractive index of the material of a prism of refracting angle 45∘ is 1.6 for a certain monochromatic ray. What will be the minimum angle of incidence of this ray on the prism so that no TIR takes place as the ray comes out of the prism.
View Solution
