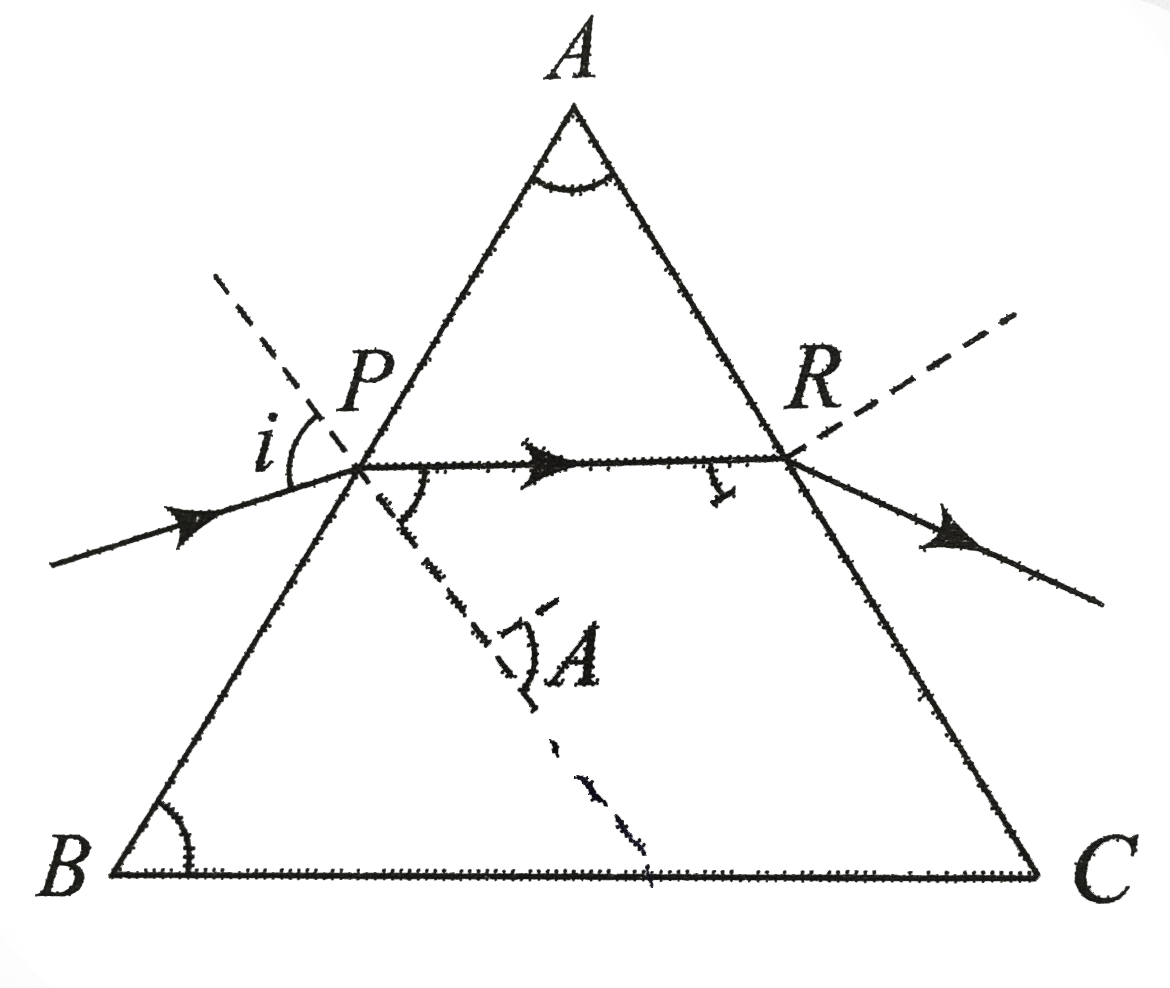
What is the angle of incidence for an equilateral prism of refractive index √3 so that the ray si parallel to the base inside the prism?
30∘
45∘
60∘
Either 30∘ or 60∘
The correct Answer is:c.
To solve the problem of finding the angle of incidence for an equilateral prism with a refractive index of √3 such that the ray is parallel to the base inside the prism, we can follow these steps:
Step 1: Understand the Geometry of the Prism
The prism is equilateral, meaning each angle is 60∘. We denote the angle of the prism as A=60∘.
Step 2: Use the Formula for Refractive Index
The refractive index (μ) of the prism can be expressed in terms of the angle of deviation (δ) and the angle of the prism (A) using the formula:
μ=sin(A+δ2)sin(A2)
Since the ray is parallel to the base inside the prism, the angle of deviation (δ) can be determined.
Step 3: Substitute Known Values
Given that μ=√3 and A=60∘:
- A2=60∘2=30∘
- sin(30∘)=12
Now, substituting these values into the refractive index formula:
√3=sin(60∘+δ2)sin(30∘)=sin(60∘+δ2)12
This simplifies to:
√3=2sin(60∘+δ2)
Step 4: Solve for δ
Rearranging gives:
sin(60∘+δ2)=√32
The angle whose sine is √32 is 60∘. Thus:
60∘+δ2=60∘
Multiplying both sides by 2:
60∘+δ=120∘
Solving for δ:
δ=120∘−60∘=60∘
Step 5: Apply the Angle of Deviation Formula
Using the relationship for prisms:
I+E=A+δ
Where I is the angle of incidence and E is the angle of emergence. For the case where the angle of incidence is equal to the angle of emergence (I=E):
2I=A+δ
Substituting the known values:
2I=60∘+60∘=120∘
Thus:
I=120∘2=60∘
Final Answer
The angle of incidence I is 60∘.
---
The prism is equilateral, meaning each angle is 60∘. We denote the angle of the prism as A=60∘.
The refractive index (μ) of the prism can be expressed in terms of the angle of deviation (δ) and the angle of the prism (A) using the formula:
μ=sin(A+δ2)sin(A2)
Since the ray is parallel to the base inside the prism, the angle of deviation (δ) can be determined.
Given that μ=√3 and A=60∘:
- A2=60∘2=30∘
- sin(30∘)=12
√3=sin(60∘+δ2)sin(30∘)=sin(60∘+δ2)12
This simplifies to:
√3=2sin(60∘+δ2)
Rearranging gives:
sin(60∘+δ2)=√32
The angle whose sine is √32 is 60∘. Thus:
60∘+δ2=60∘
Multiplying both sides by 2:
60∘+δ=120∘
Solving for δ:
δ=120∘−60∘=60∘
Using the relationship for prisms:
I+E=A+δ
Where I is the angle of incidence and E is the angle of emergence. For the case where the angle of incidence is equal to the angle of emergence (I=E):
2I=A+δ
Substituting the known values:
2I=60∘+60∘=120∘
Thus:
I=120∘2=60∘
The angle of incidence I is 60∘.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig
2 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Linked Comprehension
51 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Subjective
26 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
The angle of incidance for an equilateral prism is 60∘. What should be the refractive index of prism so that the ray is parallel to the base inside the prism ?
View SolutionA ray of light undergoes deviation of 30∘ when incident on an equilateral prism of refractive index √2. The angle made by the ray inside the prism with the base of the prism is
View SolutionA ray of light undergoes a deviation of 30∘ when incident on an equilateral prism of refractive index √2.
What is the angle subtended by the ray inside the prism with the base of the prism?
View SolutionA ray of light undergoes deviation of 30∘ when incident on an equilateral prism of refractive index √2. what is the angle subtended by the ray inside the prism with the base of the prism?
View SolutionIf the angle of minimum deviation is of 60∘ for an equilateral prism , then the refractive index of the material of the prism is
View SolutionFind the angle of minimum deviation for an equilateral prism made of a material of refractive index 1.732. What is the angle of incidence for this deviation ?
View SolutionRefractive index of an equilateral prism is √2.
View SolutionA ray of light incident at an angle of incidence 'I' passes through an equilateral glass prism such that the refracted ray inside the prism is parallel to its base and emerges from the prism at an angle of emergence 'e'.
How is the angle of emergence 'e' related to the angle of incidence 'i' ?
View SolutionA ray of light incident at an angle of incidence 'I' passes through an equilateral glass prism such that the refracted ray inside the prism is parallel to its base and emerges from the prism at an angle of emergence 'e'.
What can you say about the value of the angle of deviation in such a situation ?
View SolutionA light ray is normally incident onone face of equilateral glass prism of refractive index √2. The deviation of light ray is
View Solution
