Two capacitors C1andC2(C1>C2) are charged separtately to same potential. Now they are allowed to discharge through similar resistors. Initial rate of discharging will be
more for C1
more for C2
same for both
cannot say
The correct Answer is:C
To solve the problem, we need to analyze the initial rate of discharge of two capacitors, C1 and C2, which are charged to the same potential and then discharged through similar resistors.
1. Understanding the Problem:
We have two capacitors C1 and C2 where C1>C2. Both capacitors are charged to the same potential V0 and are then allowed to discharge through identical resistors.
2. Formula for Current During Discharge:
The current I flowing from a capacitor during discharge can be expressed as:
I(t)=V0Re−tRC
where R is the resistance and C is the capacitance of the capacitor.
3. Initial Rate of Discharge:
We need to find the initial rate of discharge, which corresponds to the current at t=0. Substituting t=0 into the equation gives:
I(0)=V0Re0=V0R
This means that at t=0, the current is simply V0R.
4. Calculating for Both Capacitors:
- For capacitor C1:
I1(0)=V0R
- For capacitor C2:
I2(0)=V0R
5. Conclusion:
Since both capacitors are discharged through the same resistor and have the same initial voltage, the initial rate of discharge (current) will be the same for both capacitors:
I1(0)=I2(0)=V0R
Final Answer:
The initial rate of discharge for both capacitors is the same.
We have two capacitors C1 and C2 where C1>C2. Both capacitors are charged to the same potential V0 and are then allowed to discharge through identical resistors.
The current I flowing from a capacitor during discharge can be expressed as:
I(t)=V0Re−tRC
where R is the resistance and C is the capacitance of the capacitor.
We need to find the initial rate of discharge, which corresponds to the current at t=0. Substituting t=0 into the equation gives:
I(0)=V0Re0=V0R
This means that at t=0, the current is simply V0R.
- For capacitor C1:
I1(0)=V0R
- For capacitor C2:
I2(0)=V0R
Since both capacitors are discharged through the same resistor and have the same initial voltage, the initial rate of discharge (current) will be the same for both capacitors:
I1(0)=I2(0)=V0R
The initial rate of discharge for both capacitors is the same.
Topper's Solved these Questions
- ELECTRIC CURRENT AND CIRCUITBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Multiple Correct
16 Videos
- ELECTRIC CURRENT AND CIRCUITBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Comprehension
35 Videos
- ELECTRIC CURRENT AND CIRCUITBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Subjective
29 Videos
- ELECTRIC CURRENT & CIRCUITSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Kirchhoff s law and simple circuits
15 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s
38 Videos
Similar Questions
Capacitors C1=1μF and C2=2μF are separately charged from the same battery. They are allowed to discharge separately through equal resistors
View SolutionA capacitor is charged to a certain potential and then allowed to discharge through a resistance R. The ratio of charge on the capacitor to current in the circuit
View SolutionTwo identical capacitors A and B are charged to the same potential and then made to discharge through resistances RA and RB respectively, with RA>RB.
View SolutionTwo capacitors C1 and C2 are charged to same potential V, but with opposite polarity as shown in the figure. The switches S1 and S2 are then closed

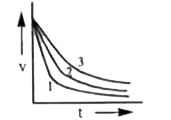
View SolutionThree identical capacitors A, B and C are charged to the same potential and then made to discharge through three resistances RA,RB and RC, where RA>RB>RC . Their potential differences (V) are plotted against time t, giving the curves 1, 2 and 3. Find the correlations between A, B, C and 1, 2, 3.
View SolutionA capacitor C1 of capacitance 1μF and a capacitor C2 of capacitance 2μF are separately charged by a common battery for a long time. The two capacitors are then separately discharged through equal resistors. Both the discharge circuits are connected at t=0.
View SolutionA capacitor C1 of capacitance 1μF and a capacitor C2 of capacitance 2μF are separately charged by a common battery for a long time. The two capacitors are then separately discharged through equal resistors. Both the discharge circuits are connected at t=0.
View SolutionA capacitor of capacitance 100(μ)F is connected across a battery of emf6.0V through a resistance of 20k(Ω)for 4.0s after the battery is disconnecting?
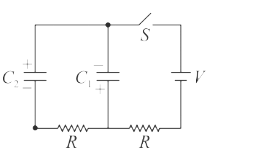
View SolutionTwo capacitors, C1andC2, charged to a potential V2 and V respectively with polarities as shown, are connected in a circuit with an ideal battery of EMF V. The capacitances are C1=2C and aC_(2)=C.Att=0, the switch S is closed. A long time after S is closed,
View SolutionTwo capacitors with capacities C1 and C2 ,are charged to potentials V1 and V2 respectively.When they are connected in parallel,the ratio of their respective charges is ....?
View Solution
