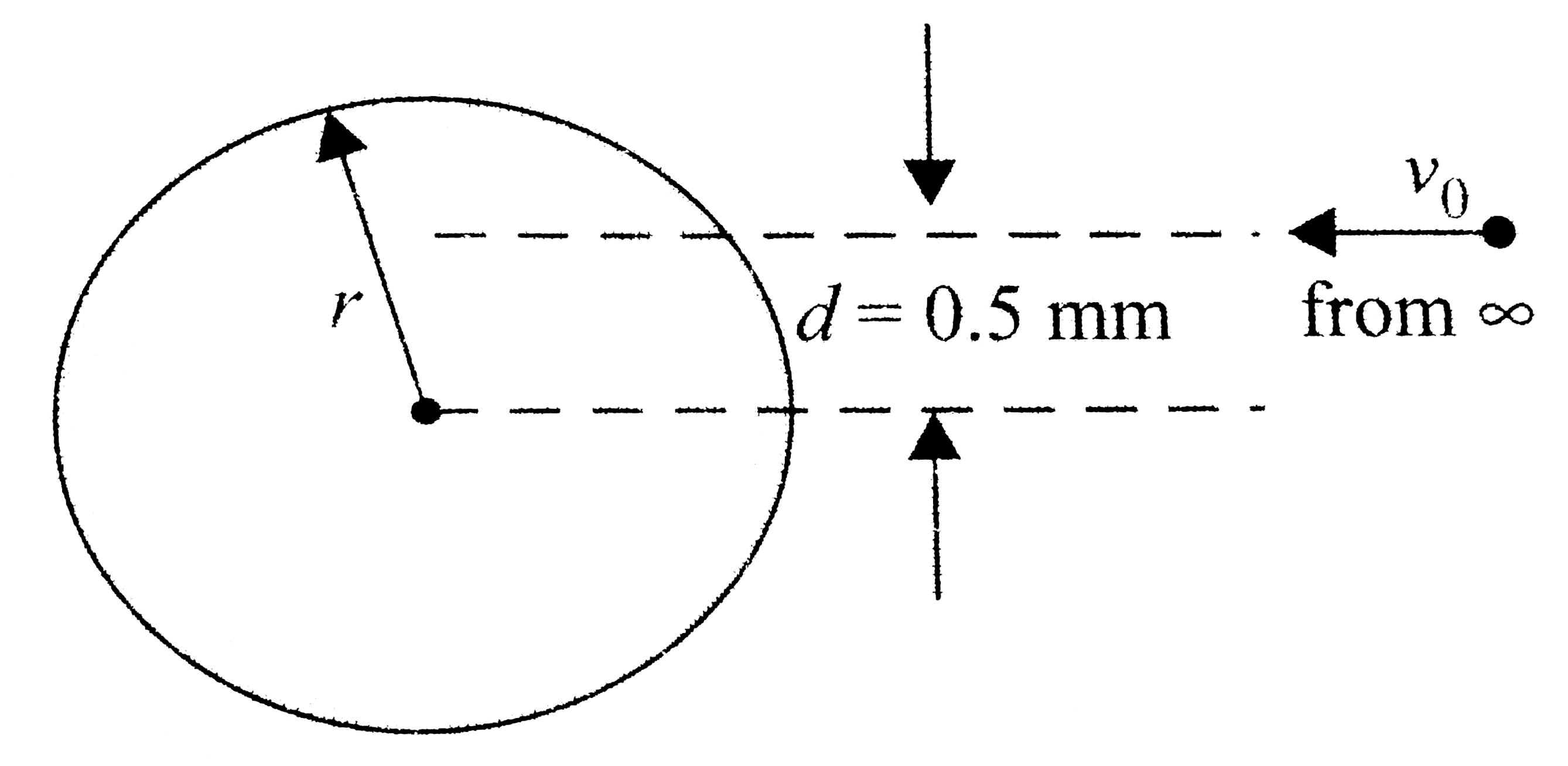
A particle of mass $1 k g$ and charge $1 / 3 μ C$ is projected toward a nonconducing fixed spherical shell of radius $r = 1 m m$ having the same charge uniformly distributed on its surface. Find th minimum initial velocity of projection requires if the particle just grazes the shell.
.

Video Solution

Text Solution

Verified by Experts

Applying conservation principle of angular momentum, we get
$m v_{0} d = m v r$
or $v = \frac{v_{0} d}{r} = \frac{v_{0}}{2} (∵ d = \frac{r}{2} = 0.5 m m)$
.
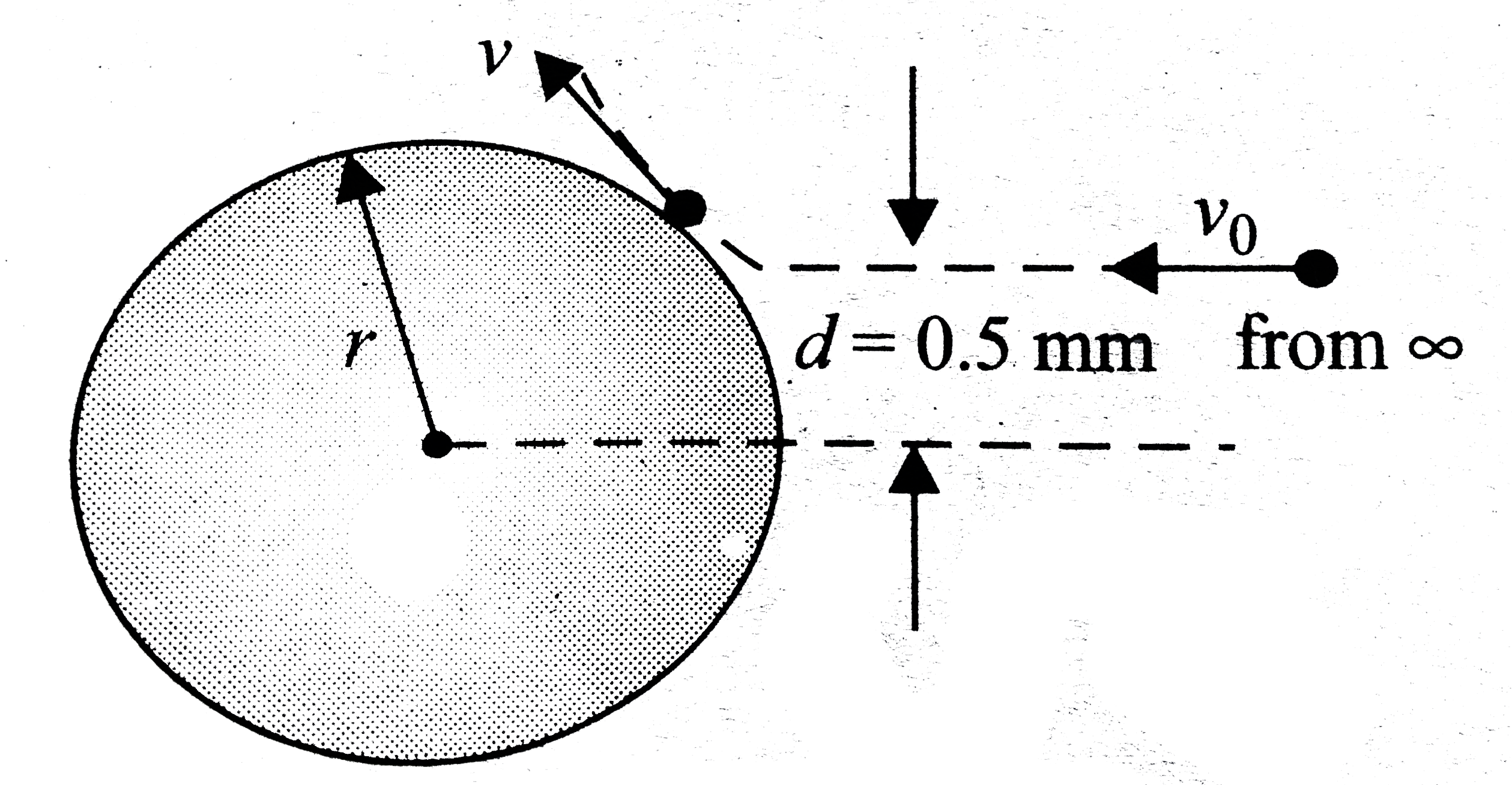
Applying mechanical energy conservation principle, we get
$U_{i} + T_{i} = U_{f} + T_{f}$
or $0 + \frac{1}{2} m v_{0}^{2} = \frac{q^{2}}{4 π ε_{0} r} + \frac{1}{2} m v^{2}$
or $\frac{1}{2} m v_{0}^{2} = \frac{q^{2}}{4 π ε_{0} r} + \frac{1}{2} m v^{2}$
or $\frac{1}{2} m v_{0}^{2} = \frac{q^{2}}{4 π ε_{0} r} + \frac{1}{2} m {(\frac{v_{0}}{2})}^{2}$
or $\frac{1}{2} m v_{0}^{2} - \frac{m v_{0}^{2}}{8} = \frac{q^{2}}{4 π ε_{0} r}$
or $\frac{1}{2} m v_{0}^{2} \times \frac{3}{4} = \frac{q^{2}}{4 π ε_{0} r}$
or $\frac{1}{2} m v_{0}^{2} = \frac{q^{2}}{3 π ε_{0} r}$
or $v_{0}^{2} = \frac{8 q^{2}}{3 \times 4 π ε_{0} m r} = \frac{8}{3}$
or $v_{0} = \sqrt{\frac{8}{3}} = 2 \sqrt{\frac{2}{3}} m s^{- 1}$ .

Updated on:21/07/2023

|

Class 12 PHYSICS ELECTROSTATICS

Topper's Solved these Questions

Similar Questions

CENGAGE PHYSICS ENGLISH-ELECTRIC POTENTIAL-Exercise 3.2

Home
Ask Doubt
Profile