The electric field in a region is radially outward with magnitude E=Ar. Find the charge contained in a sphere of radius a centred at the origin. Take A=100Vm−2 and a=20.0cm.
The correct Answer is:3×10−10C
To find the charge contained in a sphere of radius a centered at the origin, given that the electric field E in the region is radially outward with a magnitude of E=Ar, we can follow these steps:
Step 1: Identify the Electric Field
The electric field is given as:
E=Ar
where A=100V/m2 and r is the distance from the origin.
Step 2: Determine the Electric Field at Radius a
For a sphere of radius a=20.0cm=0.2m, we can substitute r=a into the electric field equation:
E(a)=A⋅a=100⋅0.2=20V/m
Step 3: Calculate the Surface Area of the Sphere
The surface area As of a sphere is given by the formula:
As=4πa2
Substituting a=0.2m:
As=4π(0.2)2=4π(0.04)=0.50265m2
Step 4: Calculate the Electric Flux through the Sphere
The electric flux ΦE through the surface of the sphere is given by:
ΦE=E⋅As
Substituting the values we calculated:
ΦE=20⋅0.50265=10.0529V m
Step 5: Use Gauss's Law to Find the Charge
According to Gauss's law, the total electric flux through a closed surface is equal to the charge enclosed Q divided by the permittivity of free space ϵ0:
ΦE=Qϵ0
Rearranging for Q:
Q=ΦE⋅ϵ0
Where ϵ0=8.85×10−12C2/N m2.
Step 6: Substitute the Values to Find Q
Substituting the values:
Q=10.0529⋅(8.85×10−12)=8.89×10−11C
Final Result
The charge contained in the sphere of radius a is approximately:
Q≈8.89×10−11C
The electric field is given as:
E=Ar
where A=100V/m2 and r is the distance from the origin.
For a sphere of radius a=20.0cm=0.2m, we can substitute r=a into the electric field equation:
E(a)=A⋅a=100⋅0.2=20V/m
The surface area As of a sphere is given by the formula:
As=4πa2
Substituting a=0.2m:
As=4π(0.2)2=4π(0.04)=0.50265m2
The electric flux ΦE through the surface of the sphere is given by:
ΦE=E⋅As
Substituting the values we calculated:
ΦE=20⋅0.50265=10.0529V m
According to Gauss's law, the total electric flux through a closed surface is equal to the charge enclosed Q divided by the permittivity of free space ϵ0:
ΦE=Qϵ0
Rearranging for Q:
Q=ΦE⋅ϵ0
Where ϵ0=8.85×10−12C2/N m2.
Substituting the values:
Q=10.0529⋅(8.85×10−12)=8.89×10−11C
The charge contained in the sphere of radius a is approximately:
Q≈8.89×10−11C
Topper's Solved these Questions
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Single Correct
40 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Multiple Correct
8 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Exercise 2.2
14 Videos
- ELECTRIC CURRENT AND CIRCUITBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Interger
8 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - DPP 3.5
15 Videos
Similar Questions
The electric field in aregion is radially ourward with magnitude E=Ar. Find the charge contained in a sphere of radius a centred at the origin. Take A=100Vm−2 and a=20.0cm.
View SolutionThe electric field in a region is radially outward with magnitude E=Aγ0. The charge contained in a sphere of radius γ0 centered at the origin is
View SolutionThe electric field in a region is radially outward with magnitude E = 2r. The charge contained in a sphere of radius a = 2m centerd at the origin is 4xπε0. Find the value of x.
View SolutionThe electric field in a certain region is acting radially outwards and is given by E=Ar.A charge contained in a sphere of radius 'a' centred at the origin of the field, will given by
View SolutionThe electric field in a region is radially outwards with magnitude E=αr/ε0. IN a sphere of radius R centered at the origin, calculate the value of charge in coulombs α=5π if V/m2 and R=(310)1/3m.
View SolutionElectric field in a region of space is radially outward from origin and varies with distance 'r' from origin as E = kr. Find the charge enclosed in a sphere of radius 'a' centred at origin.
View SolutionA point charge Q is placed at the origin . Find the electrostatic energy stored outside the sphere of radius R centred at the origin.
View SolutionThe electric field in a region is radially outward and varies with distance r as E=250 r ( volt per square metre).Calculate the charge contained in a sphere of radius 0.2 m centere at the origin.
View SolutionThe electric field in a region is directed outward and is proportional to the distance r from the origin, Taking the electric potential at origin to be zero,
View SolutionAn electric field 'E' whose direction is radially outward varies as distance from origin 'r' as shown in the graph E is taken as positive if its direction is away from the origin. Then the work done by electric field on a 2 C charge if it is taken from (1,1,0) to (3,0,0) is :
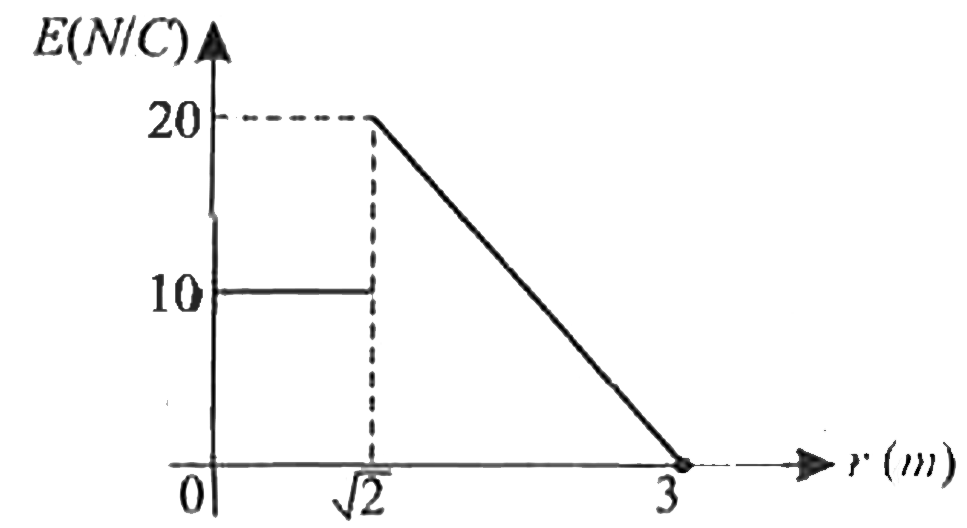
View Solution
