The maximum electric field at a point on the axis of a uniformly charged ring is E0. At how many points on the axis will the magnitude of the electric field be E0/2.
1
2
3
4
The correct Answer is:D
To solve the problem of finding the number of points on the axis of a uniformly charged ring where the electric field is equal to E02, we can follow these steps:
Step 1: Understand the Electric Field of a Charged Ring
The electric field E along the axis of a uniformly charged ring varies with the distance x from the center of the ring. The electric field reaches its maximum value E0 at a certain point along the axis.
Step 2: Identify the Maximum Electric Field
From the problem statement, we know that the maximum electric field at a point on the axis of the ring is E0. This maximum occurs at a specific distance from the center of the ring.
Step 3: Analyze the Electric Field Graph
The electric field E as a function of distance x from the center of the ring can be represented graphically. The graph will show that the electric field starts at zero, increases to a maximum E0, and then decreases back to zero as you move further away from the ring.
Step 4: Determine Points Where E=E02
To find the points where the electric field is E02, we look at the graph of E versus x. The value E02 will intersect the graph at two points: one on the left side of the maximum and one on the right side of the maximum.
Step 5: Count the Intersection Points
From the graph, we can see that there are two points where the electric field equals E02 on either side of the maximum. Thus, there are a total of four points where the electric field is E02:
- Two points on the left side of the maximum (let's call them X1 and X2)
- Two points on the right side of the maximum (let's call them X3 and X4)
Conclusion
Therefore, the total number of points on the axis where the electric field is E02 is four.
Final Answer
The number of points on the axis where the magnitude of the electric field is E02 is 4.
---
The electric field E along the axis of a uniformly charged ring varies with the distance x from the center of the ring. The electric field reaches its maximum value E0 at a certain point along the axis.
From the problem statement, we know that the maximum electric field at a point on the axis of the ring is E0. This maximum occurs at a specific distance from the center of the ring.
The electric field E as a function of distance x from the center of the ring can be represented graphically. The graph will show that the electric field starts at zero, increases to a maximum E0, and then decreases back to zero as you move further away from the ring.
To find the points where the electric field is E02, we look at the graph of E versus x. The value E02 will intersect the graph at two points: one on the left side of the maximum and one on the right side of the maximum.
From the graph, we can see that there are two points where the electric field equals E02 on either side of the maximum. Thus, there are a total of four points where the electric field is E02:
- Two points on the left side of the maximum (let's call them X1 and X2)
- Two points on the right side of the maximum (let's call them X3 and X4)
Therefore, the total number of points on the axis where the electric field is E02 is four.
The number of points on the axis where the magnitude of the electric field is E02 is 4.
Topper's Solved these Questions
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICS ENGLISHChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Multiple Correct
Explore 8 Videos
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICS ENGLISHChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Comprehension
Explore 25 Videos
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICS ENGLISHChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Subjective
Explore 32 Videos
- CENGAGE PHYSICS DPPBook:CENGAGE PHYSICS ENGLISHChapter:CENGAGE PHYSICS DPPExercise:subjective type
Explore 51 Videos
- ELECTRIC CURRENT & CIRCUITSBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRIC CURRENT & CIRCUITSExercise:Kirchhoff s law and simple circuits
Explore 15 Videos
Similar Questions
The maximum electric field intensity on the axis of a uniformly charged ring of charge q and radius R will be
View SolutionConsider a uniformly charged ring of radius R. Find the point on the axis where the electric field is maximum.
View SolutionThe electric field of a point charge is uniform. Is it true or false?
View SolutionFind the electric field on the axis of a charged disc.
View SolutionThe largest magnitude the electric field on the axis of a uniformly charged ring of radius 3m is at a distance h from its centre. What is the value of h? (Take 1√2=0.7)
View SolutionFind the electric field at the centre of a uniformly charged semicircular ring of radius R. Linear charge density is λ
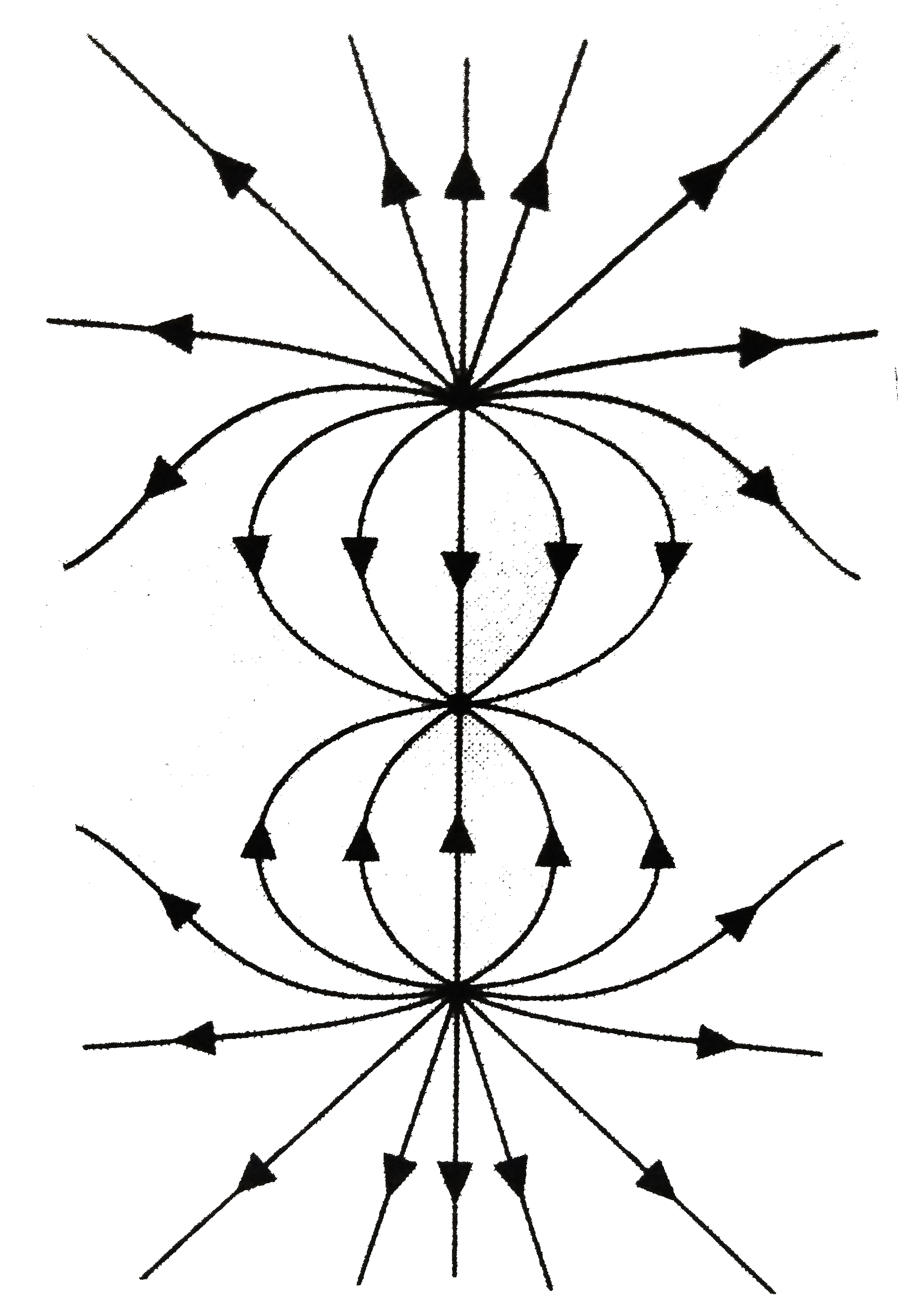
View SolutionFig. shows some of the electric field lines due to three point charges arranged along the vertical axis. All three charges have the same magnitude.
(a) What are the signs of each of the three charges? Explain your reasoning.
(b) At what points(s) is the magnitude of the electric field the smallest? Explain your reasoning. Explain how the fields produced by each individual point charge combine to give a small net field at this point or points.
(c ) Two point charges q and -q are placed at a distance d apart. What are the points at which the resultant electric field is parallel to the line joining the two charge?
 .
.
View SolutionAssertion : For a non-uniformly charged thin circular ring with net charge zero, the electric field at any point on axis of the ring is zero.
Reason : For a non-uniformly charged thin circular ring with net charge zero, the electric potential at each point on axis of the ring is zero.
View SolutionWhat is the electric field intensity at any point on the axis of a charged rod of length 'L' and linear charge density λ? The point is separated from the nearer end by a.
View SolutionA nonconducting sphere with a cavity has volume charge density ρ. O1andO2 represent the two centres as shown. The electric fields inside the cavity is E0. Now, an equal and opposite charge is given uniformly to the sphere on its outer surface. The magnitude of electric field inside the cavity becomes

View Solution
