A system consits fo a thin charged wire ring of radius R and a very long uniformly charged thread oriented along the axis of the ring, with one of its ends coinciding with the centre of the ring. The total charge of the ring, with one of the ring so equal to q. The charge of the thread (per unit length) is equal to λ. Find the interaction froce between the ring and the thread.
λq4πε0r
λq4√2πε0r
2√2λqπ2ε0r
4λqπ2ε0r
The correct Answer is:B
To find the interaction force between a charged ring and a uniformly charged thread, we can follow these steps:
Step 1: Understand the System
We have:
- A thin charged wire ring of radius R with total charge q.
- A very long uniformly charged thread with linear charge density λ, extending along the axis of the ring, with one end at the center of the ring.
Step 2: Electric Field Due to the Ring
The electric field E at a distance x from the center of the ring along its axis can be derived using the formula:
E=k⋅q⋅x(R2+x2)3/2
where k=14πϵ0.
Step 3: Charge Element of the Thread
Consider a small element of the thread of length dx at a distance x from the center of the ring. The charge on this small element is given by:
dq=λ⋅dx
Step 4: Force on the Charge Element
The force dF acting on this small charge element dq due to the electric field E produced by the ring is:
dF=dq⋅E=λ⋅dx⋅k⋅q⋅x(R2+x2)3/2
Step 5: Total Force on the Thread
To find the total force F acting on the thread, we integrate dF from x=0 to x=∞:
F=∫∞0dF=∫∞0k⋅q⋅λ⋅x⋅dx(R2+x2)3/2
Step 6: Substituting Variables
Let t2=R2+x2, then 2xdx=2tdt or xdx=tdt. The limits change from x=0 (where t=R) to x=∞ (where t=∞):
F=k⋅q⋅λ4πϵ0∫∞Rtdtt3=k⋅q⋅λ4πϵ0∫∞R1t2dt
Step 7: Evaluate the Integral
The integral ∫1t2dt=−1t:
F=k⋅q⋅λ4πϵ0(−1t)∞R=k⋅q⋅λ4πϵ0(0+1R)
Thus,
F=k⋅q⋅λ4πϵ0R
Step 8: Final Result
Substituting k=14πϵ0:
F=q⋅λ4πϵ0R
This is the interaction force between the ring and the thread.
Summary
The interaction force between the charged ring and the uniformly charged thread is given by:
F=q⋅λ4πϵ0R
We have:
- A thin charged wire ring of radius R with total charge q.
- A very long uniformly charged thread with linear charge density λ, extending along the axis of the ring, with one end at the center of the ring.
The electric field E at a distance x from the center of the ring along its axis can be derived using the formula:
E=k⋅q⋅x(R2+x2)3/2
where k=14πϵ0.
Consider a small element of the thread of length dx at a distance x from the center of the ring. The charge on this small element is given by:
dq=λ⋅dx
The force dF acting on this small charge element dq due to the electric field E produced by the ring is:
dF=dq⋅E=λ⋅dx⋅k⋅q⋅x(R2+x2)3/2
To find the total force F acting on the thread, we integrate dF from x=0 to x=∞:
F=∫∞0dF=∫∞0k⋅q⋅λ⋅x⋅dx(R2+x2)3/2
Let t2=R2+x2, then 2xdx=2tdt or xdx=tdt. The limits change from x=0 (where t=R) to x=∞ (where t=∞):
F=k⋅q⋅λ4πϵ0∫∞Rtdtt3=k⋅q⋅λ4πϵ0∫∞R1t2dt
The integral ∫1t2dt=−1t:
F=k⋅q⋅λ4πϵ0(−1t)∞R=k⋅q⋅λ4πϵ0(0+1R)
Thus,
F=k⋅q⋅λ4πϵ0R
Substituting k=14πϵ0:
F=q⋅λ4πϵ0R
This is the interaction force between the ring and the thread.
The interaction force between the charged ring and the uniformly charged thread is given by:
F=q⋅λ4πϵ0R
Topper's Solved these Questions
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Multiple Correct
8 Videos
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Comprehension
25 Videos
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Subjective
32 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type
51 Videos
- ELECTRIC CURRENT & CIRCUITSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Kirchhoff s law and simple circuits
15 Videos
Similar Questions
A very long uniformly charge thred oriented along the axis of a circle of radius R=1m rests on its centre with one of the ends. The charge per unit length on the thread is λ=16ε0. Find the flux of the vector E through the circle area in (Vm).
View SolutionA very long uniformly charged wire oriented along the axis of a circular ring of radius R rests on its centre with one of the ends (as shown in figure). The linear charge density on the wire is λ. Evalute the flux of the vector →E across the circle area.
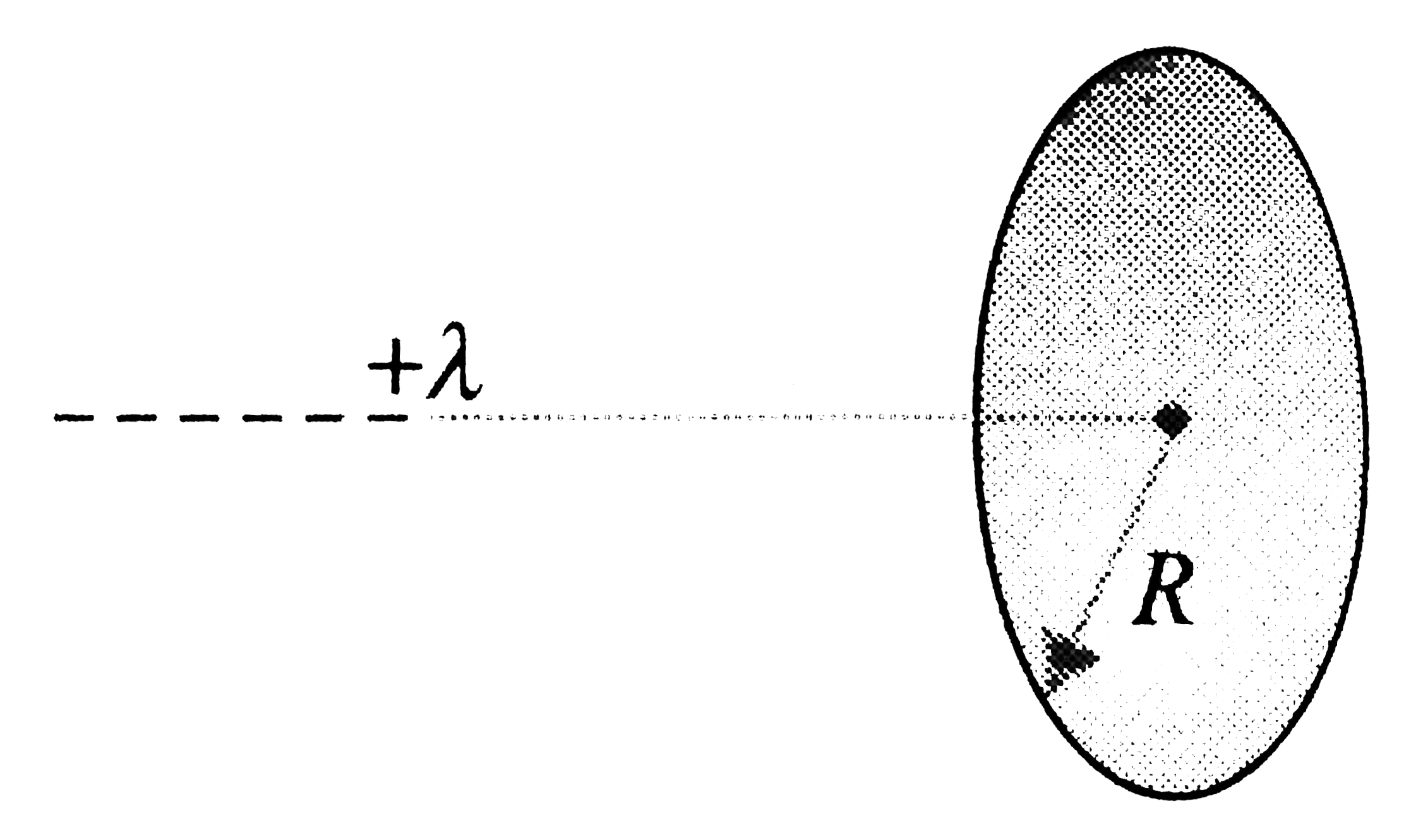
View SolutionAn unchanged conducting sphere of radius R is placed near a uniformly charge ring of radius R. Total charge on ring is Q. The centre of sphere lies on axis of ring and distance of centre of sphere from centre of ring is R

View SolutionAn electric dipole is kept on the axis of a uniformly charged ring at distance from the centre of the ring. The direction of the dipole moment is along the axis. The dipole moment is p, charge of the ring is Q & radius of the ring is R. The force on the dipole is
View SolutionElectric charge Q is uniformly distributed around a thin ring of radius a. find the potential a point P on the axis of the ring at a distance x from the centre of the ring .

View SolutionA positive charge Q is uniformly distributed along a circular ring of radius R.a small test charge q is placed at the centre of the ring.

View SolutionA positive charge Q is uniformly distributed along a circular ring of radius R.a small test charge q is placed at the centre of the ring .The

View SolutionA nonconducting ring of radius R has uniformly distributed positive charge Q. A small part of the ring.of length d, is removed (d<<R). The electric field at the centre of the ring will now be
View SolutionA half ring of radius r has a linear charge density λ.The potential at the centre of the half ring is
View SolutionA ring of radius R carries a non - uniform charge of linear density λ=λ0cosθ sec in the figure) Magnitude of the net dipolement of the ring is :

View Solution
