A cylindrical vessel of area of cross section A is filled with a liquid up to a height H. A very small hole of area of cross section a is made at the bottom of the vellel. Find the time taken by the vessel to become empty.
To find the time taken by a cylindrical vessel to become empty when a small hole is made at the bottom, we can follow these steps:
Step 1: Understand the Problem
We have a cylindrical vessel with a cross-sectional area A filled with liquid to a height H. A small hole with a cross-sectional area a is made at the bottom. We need to find the time t it takes for the vessel to empty completely.
Step 2: Apply the Continuity Equation
According to the continuity equation, the flow rate of the liquid must be constant. Therefore, we can write:
A⋅V1=a⋅V2
Where:
- V1 is the velocity of the liquid in the vessel,
- V2 is the velocity of the liquid exiting through the hole.
Since the area of the hole a is much smaller than the area of the vessel A, we can assume V2 is much greater than V1.
Step 3: Apply Bernoulli's Principle
Using Bernoulli's equation, we can relate the potential energy and kinetic energy of the liquid:
Patmosphere+ρgh+12ρV21=Patmosphere+12ρV22
Where:
- ρ is the density of the liquid,
- g is the acceleration due to gravity,
- h is the height of the liquid above the hole.
Cancelling Patmosphere and rearranging gives:
ρgh+12ρV21=12ρV22
Step 4: Simplify the Equation
Assuming V1 is negligible compared to V2, we can simplify to:
gh=12V22
Thus, we can express V2:
V2=√2gh
Step 5: Relate the Change in Height to Time
The rate of change of volume can be expressed as:
−dVdt=aV2
The volume V of the liquid in the vessel is given by:
V=Ah
Thus, we have:
−d(Ah)dt=aV2
Substituting V2:
−Adhdt=a√2gh
Step 6: Rearranging and Separating Variables
Rearranging gives:
dh√h=−aA√2gdt
Step 7: Integrate
Integrate both sides:
∫0Hdh√h=−aA√2g∫t0dt
The left side integrates to:
2√h∣∣∣0H=2√0−2√H=−2√H
The right side integrates to:
−aA√2gt
Setting both sides equal gives:
−2√H=−aA√2gt
Step 8: Solve for Time t
Rearranging for t:
t=2Aa√H√2g
Thus, the time taken for the vessel to become empty is:
t=2Aa√H2g
We have a cylindrical vessel with a cross-sectional area A filled with liquid to a height H. A small hole with a cross-sectional area a is made at the bottom. We need to find the time t it takes for the vessel to empty completely.
According to the continuity equation, the flow rate of the liquid must be constant. Therefore, we can write:
- V1 is the velocity of the liquid in the vessel,
- V2 is the velocity of the liquid exiting through the hole.
Using Bernoulli's equation, we can relate the potential energy and kinetic energy of the liquid:
- ρ is the density of the liquid,
- g is the acceleration due to gravity,
- h is the height of the liquid above the hole.
Assuming V1 is negligible compared to V2, we can simplify to:
The rate of change of volume can be expressed as:
Rearranging gives:
Integrate both sides:
Rearranging for t:
Topper's Solved these Questions
- FLUID MECHANICSBook:CENGAGE PHYSICS ENGLISHChapter:FLUID MECHANICSExercise:Solved Examples
Explore 7 Videos
- FLUID MECHANICSBook:CENGAGE PHYSICS ENGLISHChapter:FLUID MECHANICSExercise:Exercise 4.1
Explore 11 Videos
- DIMENSIONS & MEASUREMENTBook:CENGAGE PHYSICS ENGLISHChapter:DIMENSIONS & MEASUREMENTExercise:Integer
Explore 2 Videos
- GRAVITATIONBook:CENGAGE PHYSICS ENGLISHChapter:GRAVITATIONExercise:INTEGER_TYPE
Explore 1 Video
Similar Questions
A cylindrical vessel of area of cross-section A is filled with water to a height H. It has capillary tube of length l and radius r fitted horizontally at its bottom. If the coeffiecient of viscosity of water is η, then time required in which level will fall to a height H2 is (density of water is ρ)
View SolutionA cylindrical container of cross-section area, A is filled up with water upto height 'h' Water may exit through a tap of cross sectional area 'a' in the bottom of container. Find out

(a) Velocity of water just after opening of tap.
(b) The area of cross-section of water stream coming out of tape at depth h0 below tap in terms of 'a' just after opening of tap.
(c) Time in which conainer becomes empty.
(Given : (aA)1/2=0.22,h=20cm,h0=20cm
View SolutionA cylindrical vessel contains a liquid of density ρ up to height h. The liquid is closed by a piston of mass m and area of cross section A. There is a small hole at the bottom of the vessel. The speed v with which the liquid comes out of the hole is

View SolutionA cylindrical vessel contains a liquid of density ρ up to height h. The liquid is closed by a piston of mass m and area of cross section A. There is a small hole at the bottom of the vessel. The speed v with which the liquid comes out of the hole is

View SolutionA cylindrical vessel is filled with a liquid up to a height H. A small hole is made in the vessel at a distance y below the liquid surface as shown in figure. The liquid emerging from the hole strike the ground at distance x
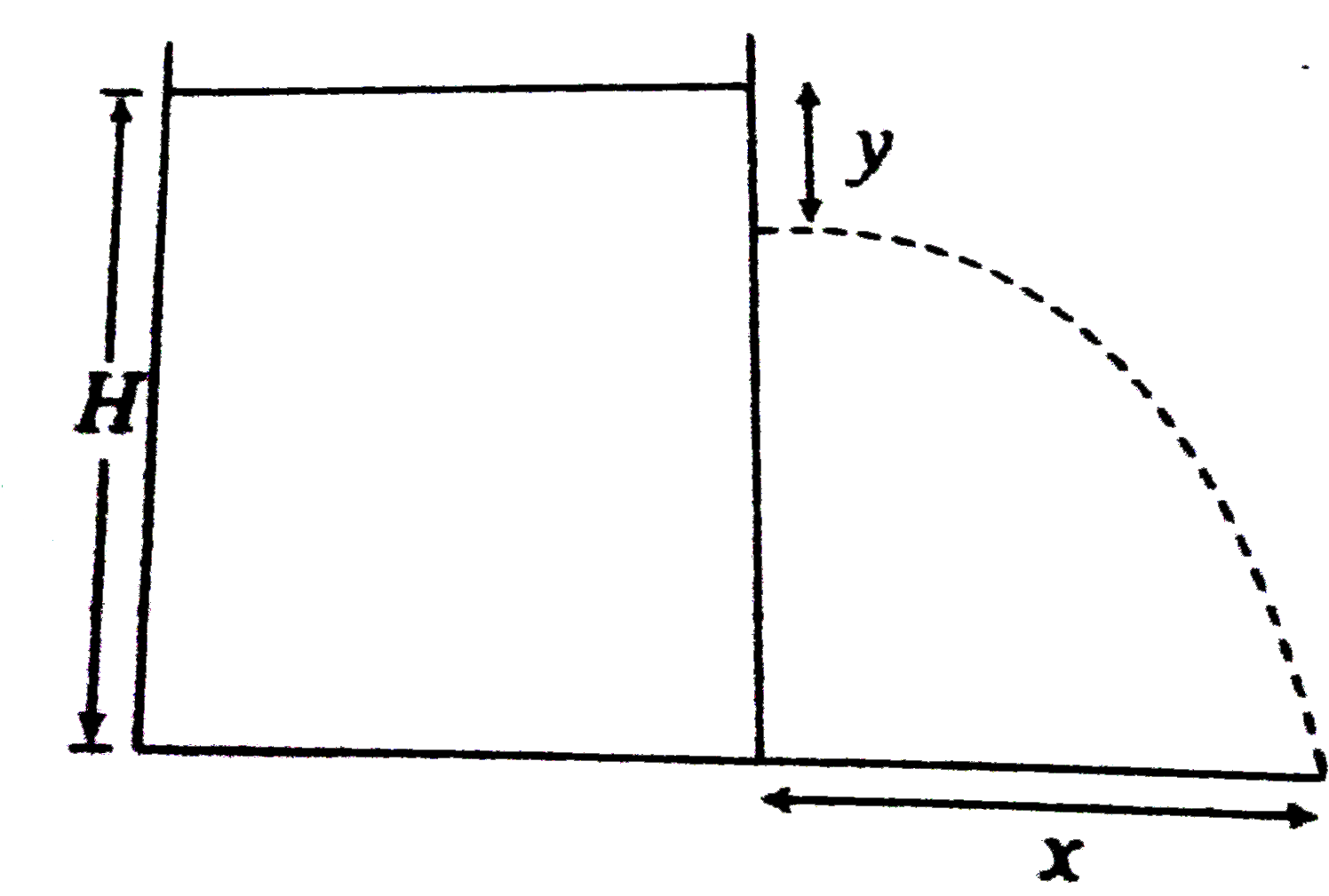
View SolutionLiquid is filled in a container upto a height of H. A small hole is made at the bottom of the tank. Time taken to empty from H to H3 is t0. Find the time taken to empty tank from H3 to zero.
View SolutionA cylindrical vessel is filled with water upto a height of 1m. The cross-sectional area of the orifice at the bottom is (1/400) that of the vessel.
(a) What is the time required to empty the tank through the orifice at the bottom?
(b) What is the time required for the same amount of water to flow out if the water level in tank is maintained always at a height of 1m from orifice?
View SolutionA cylindrical vessel open at the top is 20cm high and 10cm in diameter. A circular hole of cross-sectional area 1cm2 is cut at the centre of the bottom of the vessel. Water flows from a tube above it into the vessel at the rate of 102cm3/s. The height of water in the vessel under steady state is (Take g=10m/s2).
View SolutionThe magnetic energy stored in a long solenoid of area of cross-section A in a small region of length L is
View SolutionA fixed container of height H with large cross-sectional area A is completely filled with water. Two small orifice of cross-sectional area a are made, one at the bottom and the other on the vertical side of the container at a distance H/2 from the top of the container find the time taken by the water level to reach a height of H/2 from the bottom of the container.
View Solution