A thin bar of mass M and length L is free to rotate about a fixed horizontal axis through a point at its end. The bar is brought to a horizontal position and then released. The axis is perpendicular to the rod. The angular velocity when it reaches the lowest point is
directly proportional to its length and inversely proportional to its mass
independent of mass and inversely proportional to the square root of its length
dependent only upon the acceleration due to gravity and the length of the bar
directly proportional to its length and inversely proportional to the acceleration due to gravity
The correct Answer is:B, C, D
To solve the problem, we will use the principle of conservation of energy and the work-energy theorem. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the Initial and Final Conditions
- The bar is initially horizontal and then released. At this point, it has potential energy and no kinetic energy.
- When the bar reaches the lowest point (vertical position), it has kinetic energy due to its rotation about the fixed axis.
Step 2: Calculate the Work Done by Gravity
- The center of mass of the bar is located at a distance of L2 from the pivot point.
- The change in height of the center of mass when the bar moves from horizontal to vertical is L2.
- The work done by gravity W can be calculated as:
W=mgh=mg(L2)=mgL2
Step 3: Apply the Work-Energy Theorem
- According to the work-energy theorem, the work done by gravity is equal to the change in kinetic energy:
W=ΔKE
- Since the initial kinetic energy is zero (the bar is released from rest), we have:
mgL2=KEfinal−KEinitial=KEfinal−0=KEfinal
Step 4: Express the Final Kinetic Energy
- The final kinetic energy of the rotating bar can be expressed as:
KEfinal=12Iω2
- The moment of inertia I of a thin bar about an end is given by:
I=13mL2
- Therefore, substituting for KEfinal:
mgL2=12(13mL2)ω2
Step 5: Simplify the Equation
- Cancel m from both sides (assuming m≠0):
gL2=16L2ω2
- Rearranging gives:
ω2=3gL
Step 6: Solve for Angular Velocity
- Taking the square root of both sides, we find:
ω=√3gL
Final Answer
The angular velocity ω when the bar reaches the lowest point is:
ω=√3gL
- The bar is initially horizontal and then released. At this point, it has potential energy and no kinetic energy.
- When the bar reaches the lowest point (vertical position), it has kinetic energy due to its rotation about the fixed axis.
- The center of mass of the bar is located at a distance of L2 from the pivot point.
- The change in height of the center of mass when the bar moves from horizontal to vertical is L2.
- The work done by gravity W can be calculated as:
W=mgh=mg(L2)=mgL2
- According to the work-energy theorem, the work done by gravity is equal to the change in kinetic energy:
W=ΔKE
- Since the initial kinetic energy is zero (the bar is released from rest), we have:
mgL2=KEfinal−KEinitial=KEfinal−0=KEfinal
- The final kinetic energy of the rotating bar can be expressed as:
KEfinal=12Iω2
- The moment of inertia I of a thin bar about an end is given by:
I=13mL2
- Therefore, substituting for KEfinal:
mgL2=12(13mL2)ω2
- Cancel m from both sides (assuming m≠0):
gL2=16L2ω2
- Rearranging gives:
ω2=3gL
- Taking the square root of both sides, we find:
ω=√3gL
The angular velocity ω when the bar reaches the lowest point is:
ω=√3gL
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Multiple Correct
26 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Linked Comprehension
71 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Subjective
19 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
Similar Questions
A thin bar of mass m and length l is free to rotate about a fixed horizontal axis through a point at its end.The bar is brought to a horizontal position. (θ=90∘) and then released. The angular velocity when it reaches the lowest point is
View SolutionA uniform rod of mass m and length L is free to rotate in the vertical plane about a horizontal axis passing through its end. The rod initially in horizontal position is released. The initial angular acceleration of the rod is:

View SolutionA rod of length L is hinged from one end. It is brought to a horizontal position and released. The angular velocity of the rod, when it is in vertical position, is
View SolutionA thin uniform rod of mass M and length L is free to rotate in vertical plane about a horizontal axis passing through one of its ends. The rod is released from horizontal position shown in the figure. Calculate the shear stress developed at the centre of the rod immediately after it is released. Cross sectional area of the rod is A. [For calculation of moment of inertia you can treat it to very thin]

View SolutionA uniform rod of mass M and length L, which is free to rotate about a fixed vertical axis through O, is lying on a frictionless horizontal table. A particle of equal mass strikes the rod with a velocity V0 and sticks to it. The angular velocity of the combination immediately after the collision is:
View Solution
A uniform disc of mass M and radius R is pivoted about the horizontal axis through its centre C A point mass m is glued to the disc at its rim, as shown in figure. If the system is released from rest, find the angular velocity of the disc when m reaches the bottom point B.
View Solution
A uniform rod of length L is free to rotate in a vertical plane about a fixed horizontal axis through B. The rod begins rotating from rest. The angular velocity ω at angle θ is given as
View Solution
A uniform rod of legth L is free to rotate in a vertica plane about a fixed horizontal axis through B. The rod begins rotating from rest. The angular velocity ω at angle θ is given as
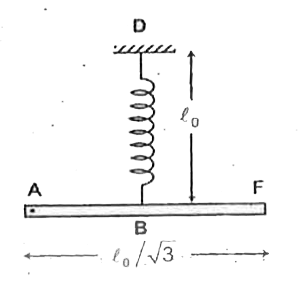
View SolutionIn the figure shown one end of a light spring of natural length l0=√58m(≈0.8m) is fixed at point D and other end is attached to the centre B of a uniform rod AF of length l0/√3 and mass 10kg. The rod is free to rotate in a vertical plane about a fixed horizontal axis passing through the end A of the rod. The rod is held at rest in horizontal position and the spring is in relaxed state. It is found that, when the rod is released to move it makes an angle of 60∘ with the horizontal when it comes to rest for the first time. Find the
(a) the maximum elogation in the spring . (Approximately)
(b) the spring constant. (Approximately)
View SolutionA uniform rod of length l and mass m is free to rotate in a vertical plane about A as shown in Fig. The rod initially in horizontal position is released. The initial angular acceleration of the rod is

View Solution
