A uniform solid cylinder of mass m rests on two horizontal planks. A thread is wound on the cylinder. The hanging end of the thread is pulled vertically down with a constant force F.
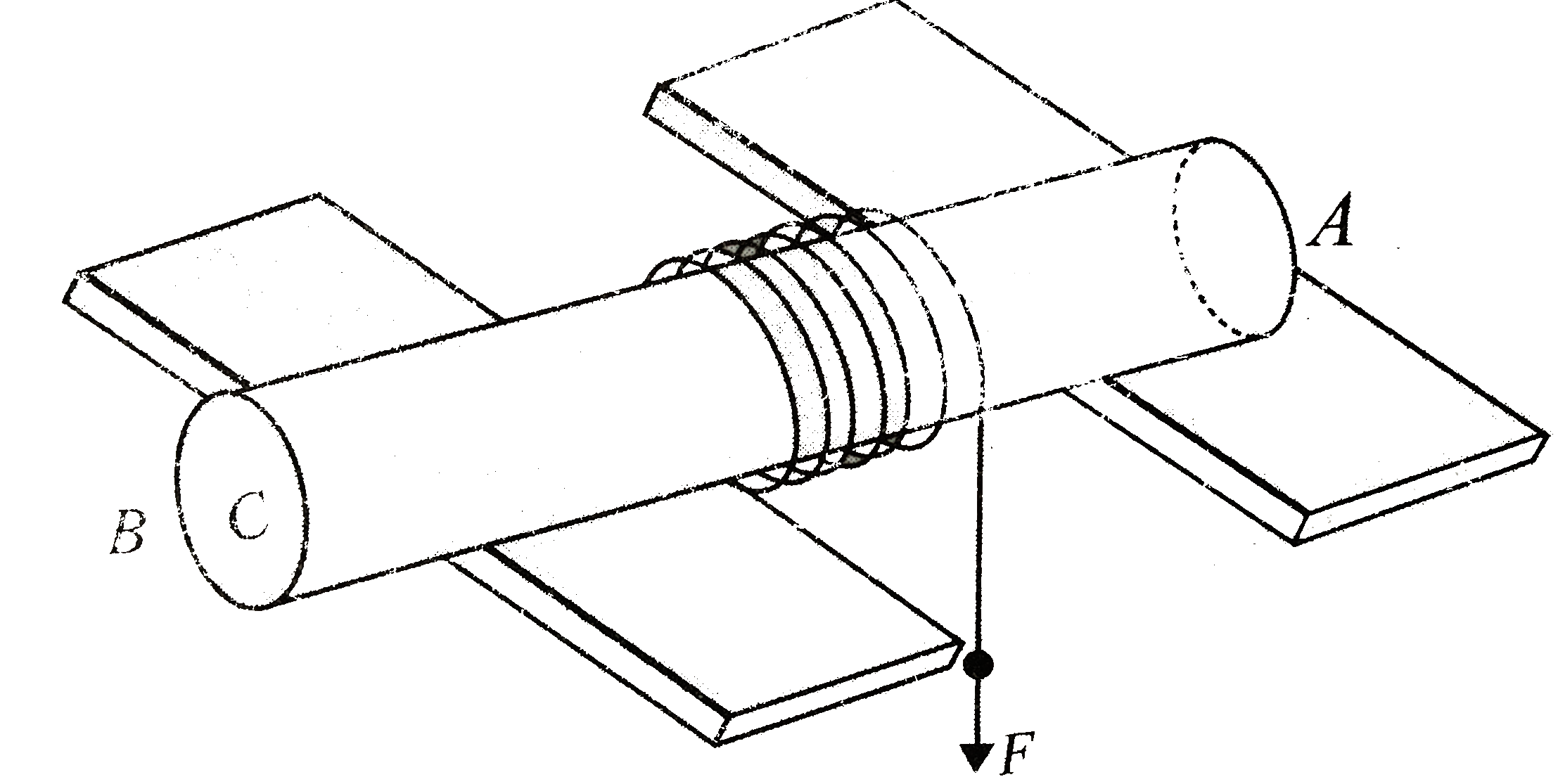
Find the maximum magnitude of the force F which may be applied without bringing about any sliding of the cylinder, if the coefficient of friction between the plank and the cylinder is equal to μ. What is the maximum acceleration of the centre of mass over the planks?
Since horizontal acceleration is provided by the friction acting on the cylinder, its translation kinetic energy (12mv2) is equal to work done by this friction,
Since moment about instantaneous axis of rotation is produced by force F. kinetic energy (12Iω2) is equal to work done by F where I is moment of inertia about the instantaneous axis of rotation and ω is the-angular velocity.
Since cylinder is moving, energy is lost against friction.
Work done by F+ work done by friction on cylinder =totalKE of the cylinder.
The correct Answer is:B, D
When force F acts vertically downwards on the thread, the thread unwinds and the cylinder rotates clockwise. Hence, its surface tries to slip over the planks in a backward direction. Hence, a friction comes into existence and acts on the cylinder in a forward direction (rightward direction). It is the only horizontal force acting on the cyliner. Due to the friction, cylinder experiences a rightward translational acceleration. But the point at which the friction acts remains always at rest, therefore no energy is lost against the friction.
since the work is done by F and no energy is lost against the friction therefore total kinetic enegy of the cylinder at any instant is equal to work done by F. Hence options a and c are incorrect and option d is correct.
If I is the moment of inertia of a body about its instantaneous axis of rotation, then the total KE of the rotating body is equal to Iω2/2. Hence option b is correct.
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Multiple Correct
26 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Linked Comprehension
71 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Subjective
19 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
Similar Questions
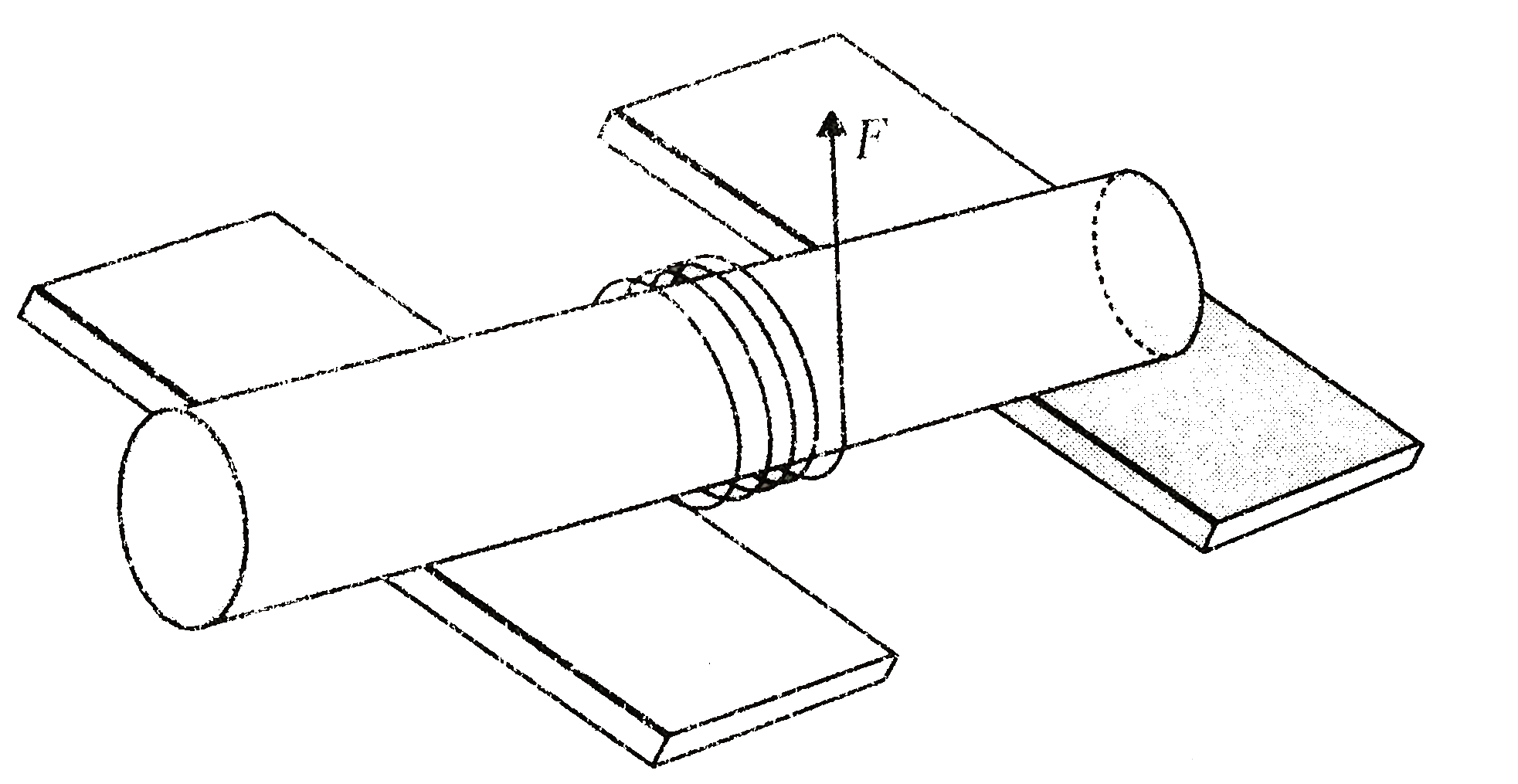
A uniform solid cylinder of mass m rests on two horizontal planks as shown in Fig. A thread is wound on the cylinder. The hanging end of the thread is pulled vertically up with a force F. What is the maximum magnitude of the force F which still does not bring about any sliding of the cylinder, if the coefficient of friction between the cylinder and the planks is equal to μ. What is the maximum acceleration of the axis of the cylinder?
View SolutionA solid cylinder is rolling down on an inclined plane of angle θ. The minimum value of the coefficient of friction between the plane and the cylinder to allow pure rolling
View Solution
Knowledge Check
A cylinder of radius R and mass M rolls without slipping down a plane inclined at an angle θ. Coeff. of friction between the cylinder and the plane is μ. For what maximum inclination θ, the cylinder rolls without slipping ?
Atanθ gt 3μsBtanθ ≤ 3μsCtanθ lt 3μsDNone of theseA rope is wound round a hollow cylinder of mass 3kgandradius40cm. If the rope is pulled with a force of 30N, what is the angualr acceleration of the cylinder ?
A15rads−2B20rads−2C25rads−2D30rads−2
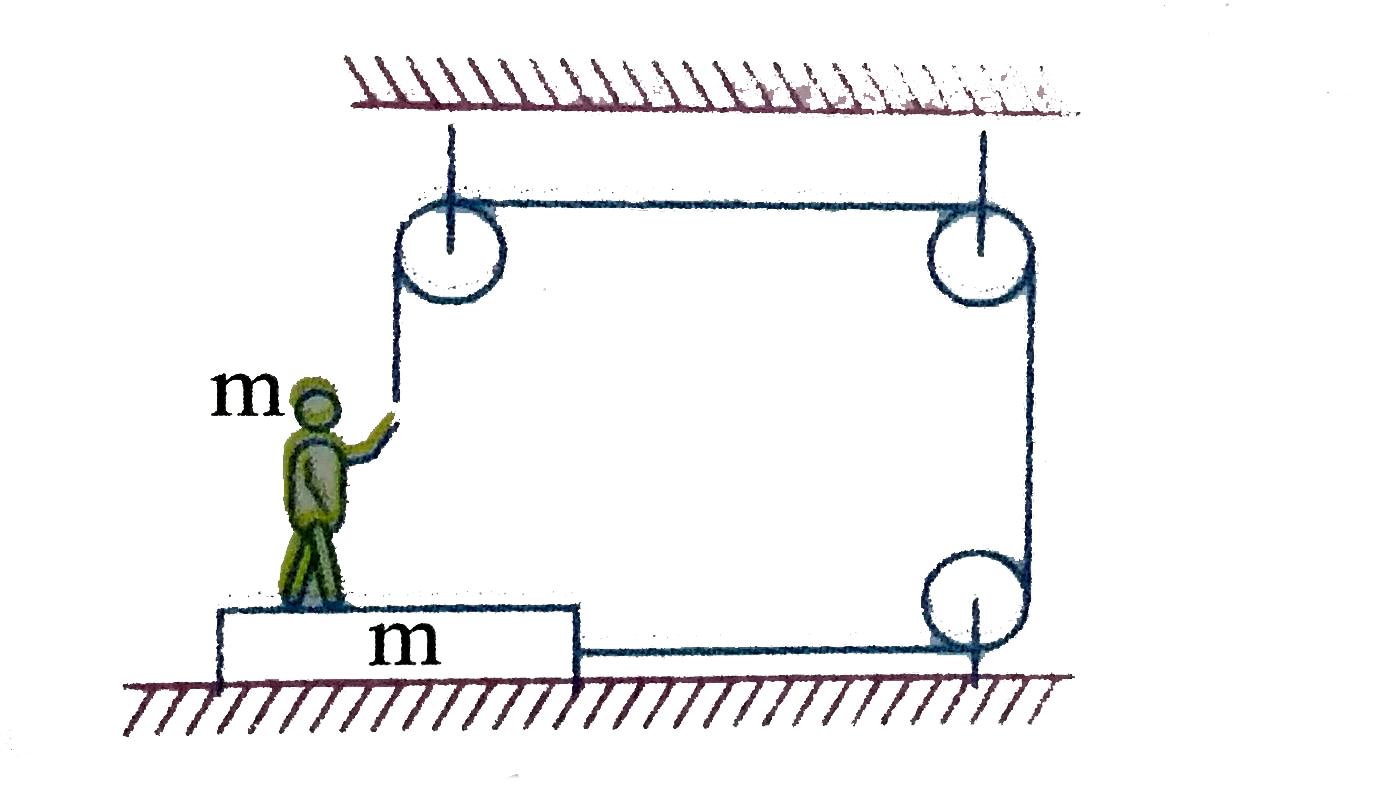
The friction coefficient between plank and floor is μ. The man applies, the maximum possible force on the string and the system remains at rest. Then
View Solution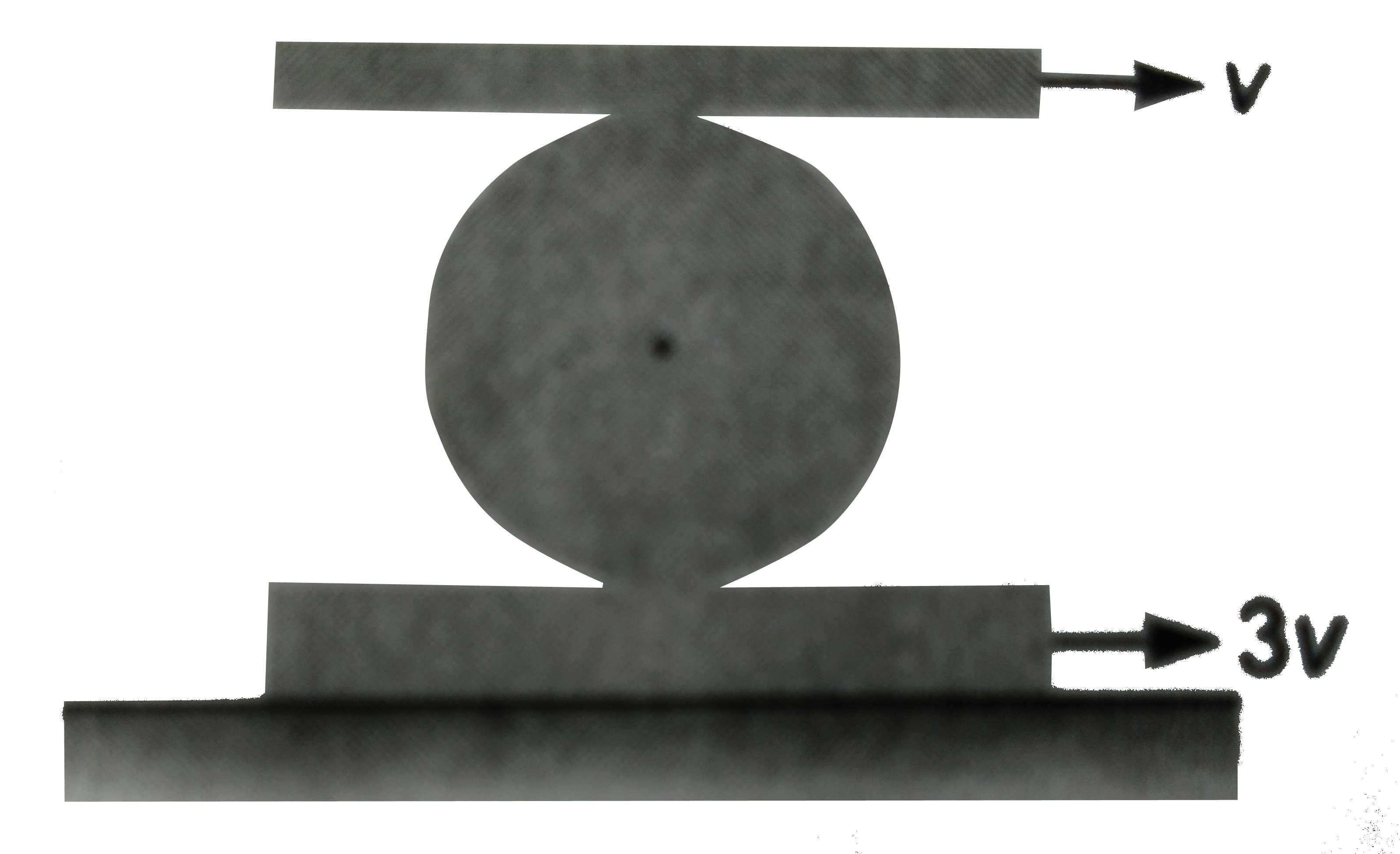
A cylinder of radius R is to roll without slipping between two planks as shown in the figure. Then
View Solution
A uniform solid cylinder of mass m and radius R is placed on a rough horizontal surface. A horizontal constant force F is applied at the top point P of the cylinder so that it start pure rolling. The acceleration of the cylinder is
View Solution
A uniform solid cylinder having mass M and radius R is pulled by a horiozntal force F acting through the center as shown. The cylinder rolls to the right without slipping. What is the magnitude of the force of friction between the cylinder and the ground?
View SolutionUniform solid cylinder of mass m and radius R is placed on a rough horizontal surface. A horizontal constant force is applied at the top point P of the cylinder so that it start pure rolling .In the above question, the frictional force on the cylinder is
View SolutionThe figure shows a spool placed at rest on a horizontal rough surface. A tightly wound string on the inner cylinder is pulled horizontally with a force F. identify the correct alternative related to the friction f acting on the spool
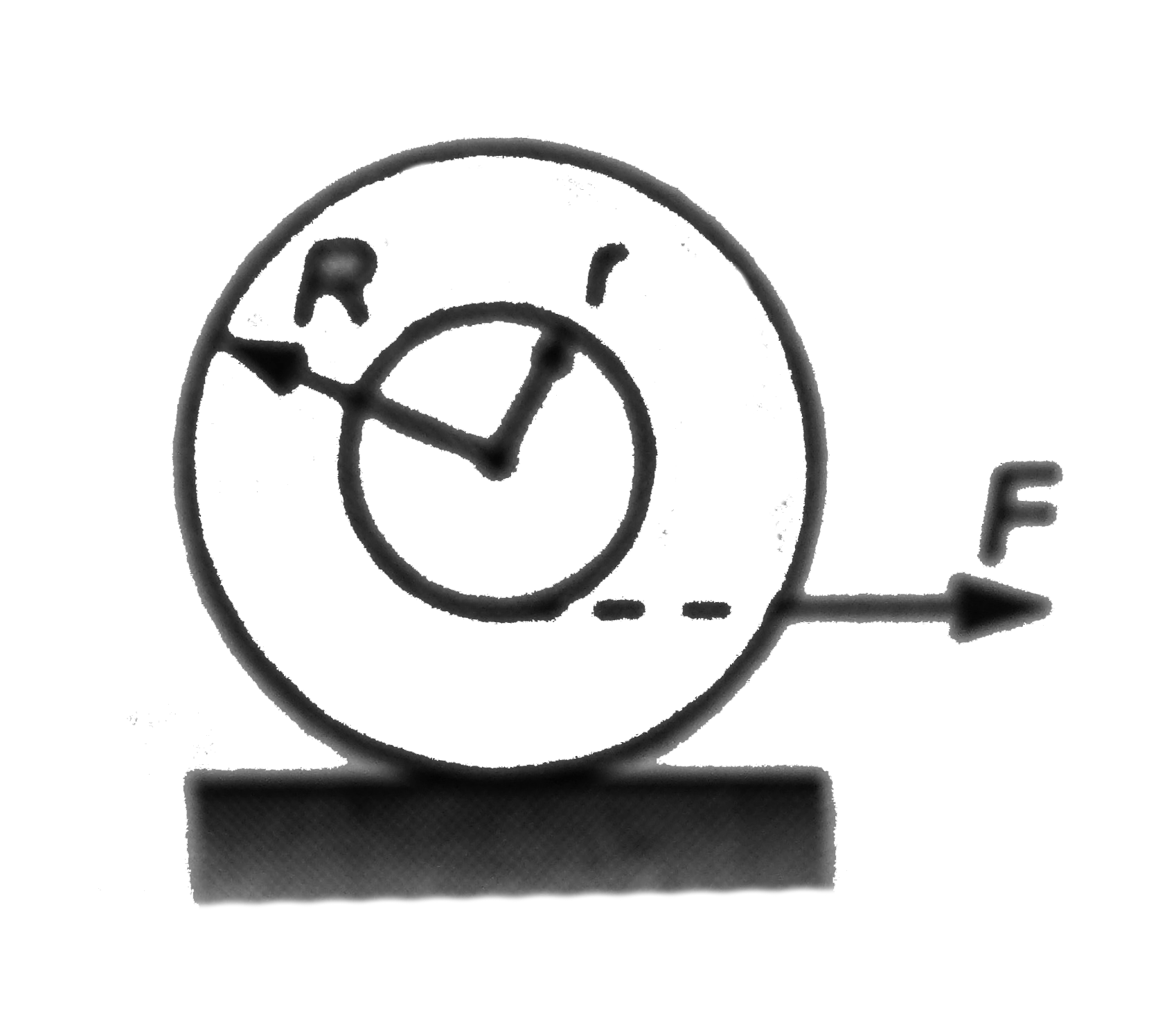
View Solution
