A trolley intially at rest with a solid cylinder placed on its bed such that cylinder axis makes angle θ with direction of motion of trolley as shown in the figure starts to move forward with constant acceleration a. If initial distance of midpoint of cylinder axis from rear edge of trolley bed is d, calculate the distance s which the trolley goes before the cylinder rolls off the edge of its horizontal bed. Assume dimensions of cylinder to be very small in comparision to other dimensions. Neglect slipping.
Calculate als, frictional force acting on the cylinder.

Since axis of cylinder is inclined at angle θ with the direction of motion of trolley, therefore components of acceleration a of trolleys, are acosθ along axis of cylinder and asinθ norma to axis of the cylinder. Cylinder rolls backward due to this normal component asinθ.

Due to angular acceleration cylinder axis has acceleration relative trolley bed, which will be equal to rα normal to cylinder axis. But component of accelerations of trolley normal to cylinder axis is sinθ. Therefore, net acceleration of cyinnder axis (asinθ−rα)
normal to axis.
Consider free body diagram of the cylinder as shown in the figure.
F2=masinθ
F2 is not shown in the free body diagram because in this diagram forces acting nromjal to cylinder axis are shown.
For horizontal forces. F1=m(asinθ−rα).........i
Taking moment about cyinder axis F1r=Iα
I=mr22⇒F1=12mrα ..............ii
From eqn i and ii rα=23asinθ
The cylinder will roll off the edge of trolley bed when its centre of mass reaches the edge. Since, cylinder axis is inclined at an angle θ withdirectionof motion of trolley, therefore, its centre of mass follows a straightline path relative to the trolley bed, and that staightline is normal to cylinder axis. Hence, displacement of centre of mass of the cylinder, relative to trolley is equal to (dcosecθ).
Considering motion of cylinder relative to the trolley,
u=0
Acceleration =rα=23asinθ
s=dcosecθ,t=?
Using, s=ut+12at2,t=√3dasin2θ
Now considering motion of trolley during this interval of time u=0 acceleration =a
t=√3dasin2θ,s=?
Using s=ut+12at2,s=32dcosec2θ
F1=12mrα=13masinθ
total frictional force acting on the cylinder is
F=√F21+F22=13ma√sin2θ+9cos2θ
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 2Exercise:Exercise 3.1
Explore 11 Videos
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 2Exercise:Exercise 3.2
Explore 13 Videos
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 2Exercise:Interger
Explore 2 Videos
- RIGID BODY DYNAMICS 1Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 1Exercise:Integer
Explore 11 Videos
- SOUND WAVES AND DOPPLER EFFECTBook:CENGAGE PHYSICS ENGLISHChapter:SOUND WAVES AND DOPPLER EFFECTExercise:Integer
Explore 16 Videos
Similar Questions
If the work done in pushing a trolley by applying a force of 850 N is 1275 J. calculate the distance moved by the trolley.
View SolutionA cylinder rolls down an inclined plane of inclination 30∘, the acceleration of cylinder is
View SolutionIf a solid cylinder rolls down an inclined plane, then its:
View SolutionWhen a magnet is released from rest along the axis of a hollow conducting cylinder situated vertically as shown in the figure
View SolutionA cylinder rolls up an inclined plane, reaches some height, and then rolls down (without slipping throughout these motions). The directions of the frictional force acting on the cylinder are.
View SolutionA cylinder rolls up an inclined plane, reaches some height, and then rolls down (without slipping throughout these motions). The directions of the frictional force acting on the cylinder are.
View SolutionA solid cylinder is rolling down a rough inclined plane of inclination θ. Then
View SolutionA spring block system is kept inside the smooth surface of a trolley as shown in figure.

At t=0 trolley is given an acceleration 'a' in the direction shown in figure. Write S−t equation of the block
(a) with respect to trolley.
(b) with respect to ground.
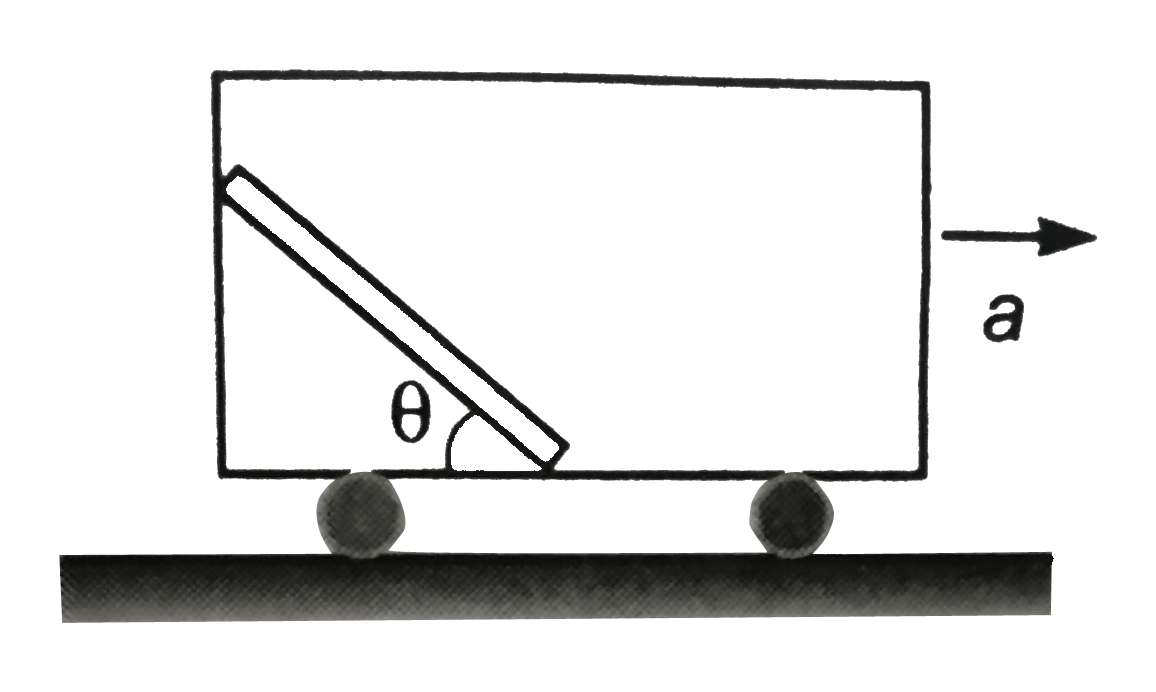
View SolutionA smooth rod length l is kept inside a trolley at an angle θ as shown in the figure. What should be the acceleration a of the trolley so that the rod remain in equilibrium with respect to it?
View SolutionA trolley moves down a slope from rest with constant acceleration. In the first second of its motion it travels 1.6 m. Its acceleration (in m/s2) is
View Solution