There are two pendulums with bobs having indencital size and mass. The pendulum A is released from rest in the position as shown in the figure. If the maximum angle formed by cord BO' with vertical in the subsequent motion of sphere B is equal to the angle θ0 If the coefficient of restitution between sphere A and sphere B is l. find

a. the velocities of sphere A and sphere B just after collisions
b. the ratio of lengths of pendulums lB/lA.
Velocity of A just before collision
v0=√2glA(1−cosθA)………..i
Collision of A and B
Using C.O.L.M. , m.v0+0=mvA+mvB
vA+vB=v0……ii
Using Newton's restitution law
vB−vA=v0
vB−vA=ev0………ii
Solving eqn i and ii vA=v02(1−e) and vB=v02(1+e)
As B swings angle θB, hence
vB=√2ghB(1−cosθB) ............iii
andv02(1+e)=√2glB(1−cosθB)
(2glA(1−cosθA)2(1+e)√2glB(1−cosθB)
⇒lBlA=(1+e2)2
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.1
20 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.2
23 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
Sphere A of mass m moving with a constant velocity u hits another stationary sphere B of the same mass. If e is the co-efficient of restitution, then ratio of velocities of the two spheres vA:vB after collision will be :
View SolutionA simple pendulum of length 1 oscillates aboμt the mean position as shown in the figure. If the total energy of the pendulum is E, the velocity of the pendulum bob of mass m at point P is:
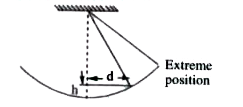
View Solution
Knowledge Check
A sphere of mass m moving with a constant velocity u hits another stationary sphere of the same mass. If e is the coefficient of restitution, then ratio of velocities of the two spheres after collision will be
A1−e1+eB1+e1−eCe+1e−1De−1e+1
A sphere A of mass m, travelling with speed v, collides directly with a stationary sphere B. If A is brought to rest and B is given a speed V, find (a) the mass of B (b) the coefficient of restitution between A and B?
View SolutionA solid sphere of mass m is lying at rest on a rough horizontal surface. The coefficient of friction between the ground and sphere is μ. The maximum value of F, so that the sphere will not slip, is equal to

View SolutionThree solid spheres each of mass m and radius R are released from the position shown in Fig. What is the speed of any one sphere at the time of collision?
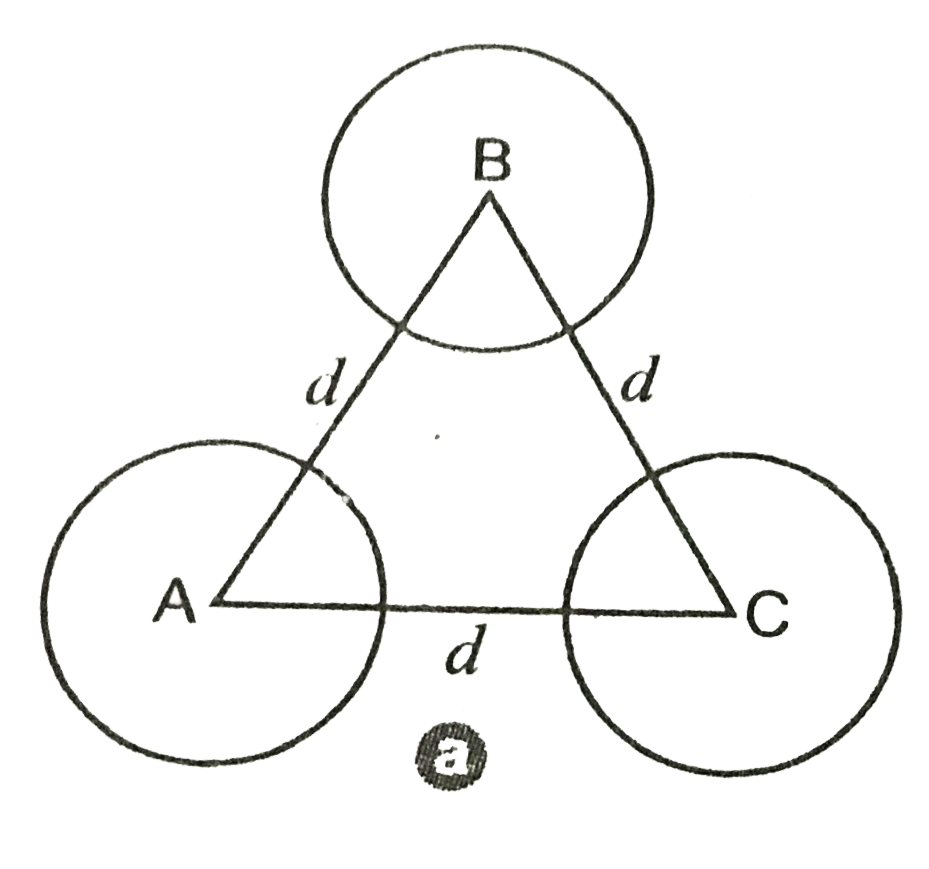
View SolutionA simple pendulum is released from rest with the string in horizontal position. The vertical component of the velocity of the bob becomes maximum, when the string makes an angle θ with the vertical. The angle θ is equal to
View SolutionA hollow spherre is released from rest on a rough inclined plane as shown in the figure. Find the velocity of point of contact as a function of time. ( m = mass of hollow sphere, R = radius of sphere )

View SolutionIn a simple pendulum, the breaking strength of the string is double the weight of the bob. The bob is released from rest when the string is horizontal. The string breaks when it makes an angle θ with the vertical.
View SolutionA sphere of mass m moving with velocity v collides head-on with another sphere of the same mass at rest. If the coefficient of resistitution e=1/2, then what is the ratio of final velocity of the second sphere to the intial velocity of the first sphere ?
View Solution
