A rope thrown over a pulley has a on one of its ends and a counterbalancing mass M on its other end. The man whose mass is m, climbs upwards by →△r relative to the ladder and then stops. Ignoring masses of the pulley and the rope, as well as the friction the pulley axis, find the displacement of the centre of mass of this system.
To solve the problem of finding the displacement of the center of mass of the system when the man climbs up the ladder, we will follow these steps:
Step 1: Understand the System
We have a man of mass m climbing a ladder, which is connected to a counterbalancing mass M via a rope over a pulley. The man climbs a distance Δr relative to the ladder.
Step 2: Define Displacements
- Let the displacement of the man relative to the ground be xm.
- The displacement of the counterbalancing mass M will be xM.
- The ladder will also move down by a distance r due to the climbing of the man.
Step 3: Relate the Displacements
When the man climbs up by Δr relative to the ladder, the displacement of the man can be expressed as:
xm=Δr−r
The displacement of the counterbalancing mass M is:
xM=−r
Step 4: Calculate the Center of Mass Displacement
The center of mass xcm of the system can be calculated using the formula:
xcm=m⋅xm+M⋅xMm+M
Substituting the values of xm and xM:
xcm=m⋅(Δr−r)+M⋅(−r)m+M
Step 5: Simplify the Expression
Expanding the equation:
xcm=mΔr−mr−Mrm+M
Combining the terms involving r:
xcm=mΔr−(m+M)rm+M
Step 6: Analyze the Result
The displacement of the center of mass is given by:
xcm=mΔrm+M−(m+M)rm+M
This indicates that the center of mass will move in the upward direction when the man climbs, as the negative term represents the downward movement of the counterbalancing mass.
Conclusion
The final displacement of the center of mass of the system when the man climbs up by Δr is:
xcm=mΔrm+M−r
This shows that the center of mass moves upward.
---
We have a man of mass m climbing a ladder, which is connected to a counterbalancing mass M via a rope over a pulley. The man climbs a distance Δr relative to the ladder.
- Let the displacement of the man relative to the ground be xm.
- The displacement of the counterbalancing mass M will be xM.
- The ladder will also move down by a distance r due to the climbing of the man.
When the man climbs up by Δr relative to the ladder, the displacement of the man can be expressed as:
xm=Δr−r
The displacement of the counterbalancing mass M is:
xM=−r
The center of mass xcm of the system can be calculated using the formula:
xcm=m⋅xm+M⋅xMm+M
Substituting the values of xm and xM:
xcm=m⋅(Δr−r)+M⋅(−r)m+M
Expanding the equation:
xcm=mΔr−mr−Mrm+M
Combining the terms involving r:
xcm=mΔr−(m+M)rm+M
The displacement of the center of mass is given by:
xcm=mΔrm+M−(m+M)rm+M
This indicates that the center of mass will move in the upward direction when the man climbs, as the negative term represents the downward movement of the counterbalancing mass.
The final displacement of the center of mass of the system when the man climbs up by Δr is:
xcm=mΔrm+M−r
This shows that the center of mass moves upward.
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.1
20 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.2
23 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
A rope thrown over a pulley has a ladder with a man of mass m on one of its ends and a counter balancing mass M on its other end. The man climbs with a velocity vr relative to ladder. Ignoring the masses of the pulley and the rope as well as the friction on the pulley axis, the velocity of the centre of mass of this system is:
View SolutionA stationary pulley carries a rope whose one end supports a ladder with a man and the other end the counterweight of mass M. The man of mass m climbs up a distance l with respect to the ladder and then stops. Neglecting the mass of the rope and the friction in the pulley axle, find the displacement I of the centre of inertia of this system.
View SolutionA pulley fixed to the ceiling carried a thread with bodies of masses m1 and m2 attached to its ends. The mases of the pulley and the thread are negligible and friction is absent. Find the acceleration of the centre of mass of this system.
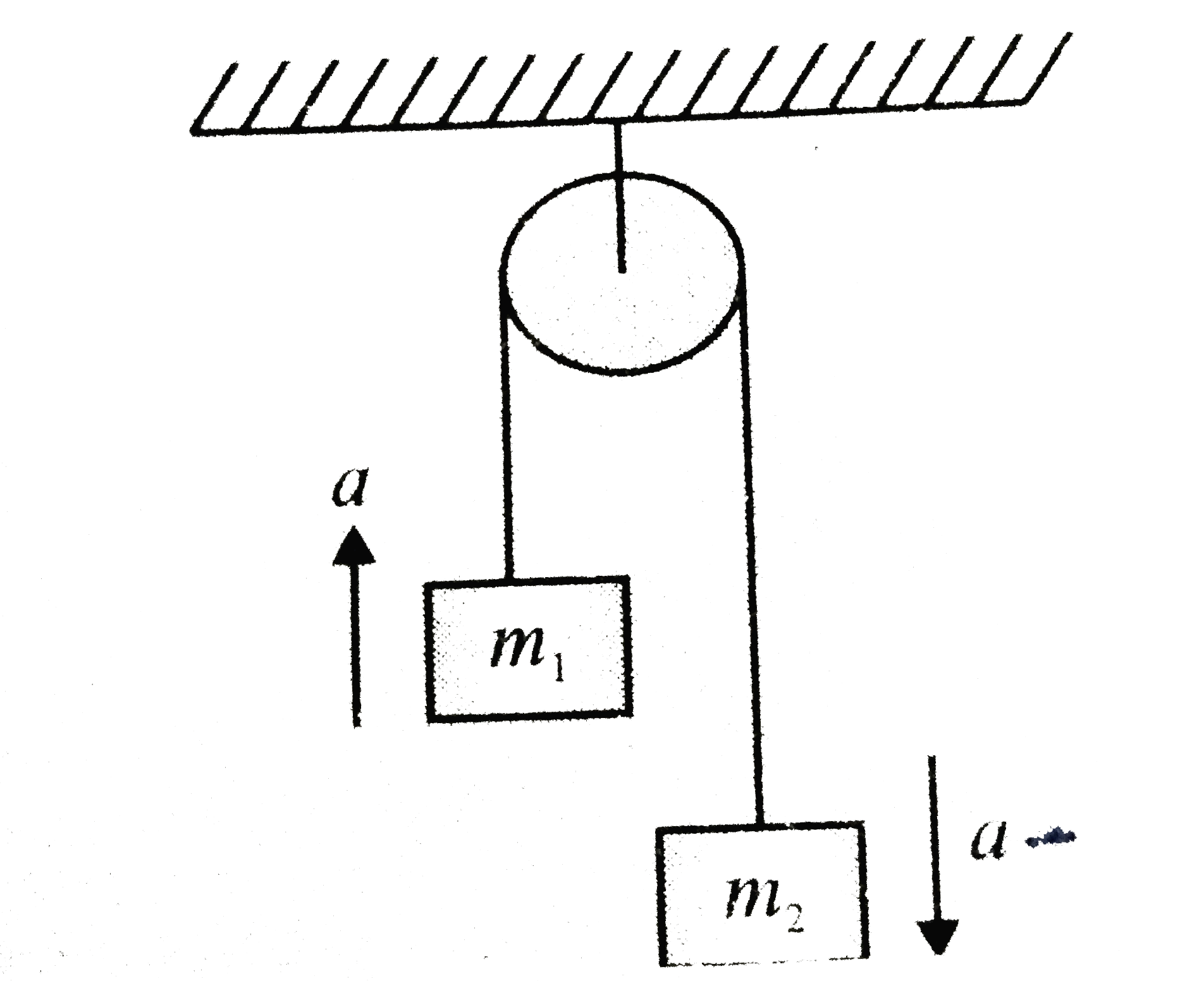
View SolutionTwo blocks of masses 6kg and 4kg are attached to the two ends of a massless string passing over a smooth fixed pulley. if the system is released, the acceleration of the centre of mass of the system will be
View SolutionTwo ladders are hanging from ands of a light rope passing over a light and smooth pulley. A monkey of mass 2m hangs near the bottom of one ladder whose mass is M-2m. Another monkey of mass m hangs near the bottom of the other ladder whose mass is M-m. The monkey of mass 2m moves up a distance l with respect to the ladder. The monkey of mass m moves up a distance l/2 with respect to the ladder. The displacement of centre of mass of the system is kml4M. Find the value of k.

View SolutionTwo bodies with masses m1 and m2(m1>m2) are joined by a string passing over fixed pulley. Assume masses of the pulley and thread negligible. Then the acceleration of the centre of mass of the system (m1+m2) is
View SolutionA rope passess over a pulley which is sufficiently high. Two monkeys of equal mass climb the rope from opposite ends, one of them climbing quickly than the other relative to the rope. Assuming that the pulley and the rope are weightless and the rope is in extensible, which of monkeys will reach the top first?
View SolutionA light rope is passed over a pulley such that at its one end a block is attached , and on the other end a boy is climbing up with acceleration g2 relative to rope. Mass of the block is 30 kg and that of the boy is 40 kg. Find the tension and acceleration of the rope .
View SolutionA pulley fixed to the ceiling of an elevator car carries a thread whose ends are attached to the masses m1andm2. The car starts going up with an acceleration a0. Assuming the masses of the pulley and the thread as well as the friction to be negligible, find:
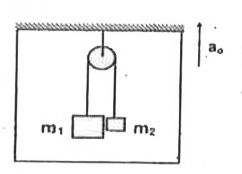
(a) the acceleration of the load m1 relative to the elevator shaft and relative to the car
(b) the force exerted by the pulley on the ceiling of the car. Given m1>m2.
View SolutionTwo blocks are resting on ground with masses 5 kg and 7 kg. A string connected them which goes over a massless pulley A. There is no friction between pulley and string A. Find acceleration of centre of mass of the system of two masses (in m/s2
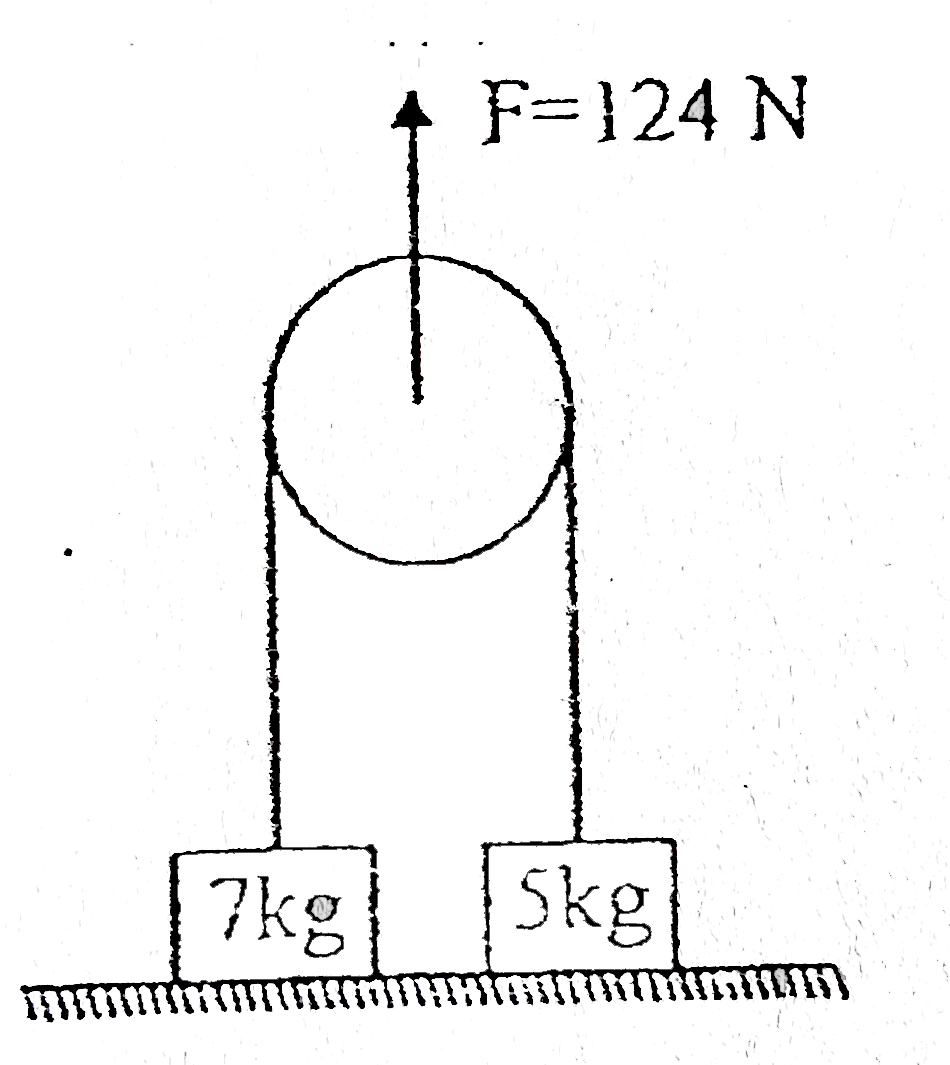
View Solution
