At what angle with the horizontal should a ball be thrown so that the range R is related to the time of flight as R=5T2 ? (Takeg=10ms6−2).
30∘
45∘
60∘
90∘
The correct Answer is:B
To find the angle at which a ball should be thrown so that the range R is related to the time of flight T by the equation R=5T2, we can follow these steps:
Step 1: Write the equations for range and time of flight
The range R of a projectile is given by the formula:
R=u2sin2θg
where u is the initial velocity, θ is the angle of projection, and g is the acceleration due to gravity.
The time of flight T is given by:
T=2usinθg
Step 2: Substitute T into the range equation
From the equation for time of flight, we can express usinθ in terms of T:
usinθ=gT2
Now, substituting this into the range equation, we get:
R=u2sin2θg=u2(2sinθcosθ)g
Substituting usinθ=gT2:
u2=(gT2sinθ)2
Thus, we can write:
R=(gT2sinθ)2(2sinθcosθ)g
This simplifies to:
R=g2T24sin2θ⋅2sinθcosθg
R=gT2cosθ2sinθ
Step 3: Set the equation R=5T2
Now, we set the expression for R equal to 5T2:
gT2cosθ2sinθ=5T2
Dividing both sides by T2 (assuming T≠0):
gcosθ2sinθ=5
Step 4: Solve for θ
Rearranging gives:
gcosθ=10sinθ
Dividing both sides by g (where g=10m/s2):
cosθ=sinθ
This implies:
tanθ=1
Thus, the angle θ is:
θ=45∘
Conclusion
The angle at which the ball should be thrown is 45∘.
---
The range R of a projectile is given by the formula:
R=u2sin2θg
where u is the initial velocity, θ is the angle of projection, and g is the acceleration due to gravity.
T=2usinθg
From the equation for time of flight, we can express usinθ in terms of T:
usinθ=gT2
Now, substituting this into the range equation, we get:
R=u2sin2θg=u2(2sinθcosθ)g
Substituting usinθ=gT2:
u2=(gT2sinθ)2
Thus, we can write:
R=(gT2sinθ)2(2sinθcosθ)g
This simplifies to:
R=g2T24sin2θ⋅2sinθcosθg
R=gT2cosθ2sinθ
Now, we set the expression for R equal to 5T2:
gT2cosθ2sinθ=5T2
Dividing both sides by T2 (assuming T≠0):
gcosθ2sinθ=5
Rearranging gives:
gcosθ=10sinθ
Dividing both sides by g (where g=10m/s2):
cosθ=sinθ
This implies:
tanθ=1
Thus, the angle θ is:
θ=45∘
The angle at which the ball should be thrown is 45∘.
Topper's Solved these Questions
- KINEMATICS-2Book:CENGAGE PHYSICS ENGLISHChapter:KINEMATICS-2Exercise:Exercise Multiple Correct
Explore 11 Videos
- KINEMATICS-2Book:CENGAGE PHYSICS ENGLISHChapter:KINEMATICS-2Exercise:Exercise Assertion - Reasoning
Explore 5 Videos
- KINEMATICS-2Book:CENGAGE PHYSICS ENGLISHChapter:KINEMATICS-2Exercise:Exercise Subjective
Explore 40 Videos
- KINEMATICS-1Book:CENGAGE PHYSICS ENGLISHChapter:KINEMATICS-1Exercise:Integer
Explore 9 Videos
- KINETIC THEORY OF GASESBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASESExercise:Compression
Explore 2 Videos
Similar Questions
A body is projected at an angle of 30∘ with the horizontal and with a speed of 30ms−1. What is the angle with the horizontal after 1.5s ? (g=10ms−2).
View SolutionA fighter plane is flying horizontally at an altitude of 1.5 km with speed 720kmh−1. At what angle of sight (w.r.t horizontal) when the target is seen, should the pilot drop the bomb in order to attack the target? (Takeg=10ms−2)
View SolutionA particle is projected from the ground with velocity u at angle θ with horizontal. The horizontal range, maximum height and time of flight are R,H and T respectively. They are given by, R=u2sin2θg,H=u2sin2θ2g and T=2usinθg Now keeping u as fixed, θ is varied from 30∘ to 60∘. Then,
View SolutionA rod AB of length 2L and mass m is lying on a horizontal frictionless surface. A particle of same mass m travelling along the surface hits the rod at distance L2 from COM with a velocity v0 in a direction perpendicular to rod and sticks to it.
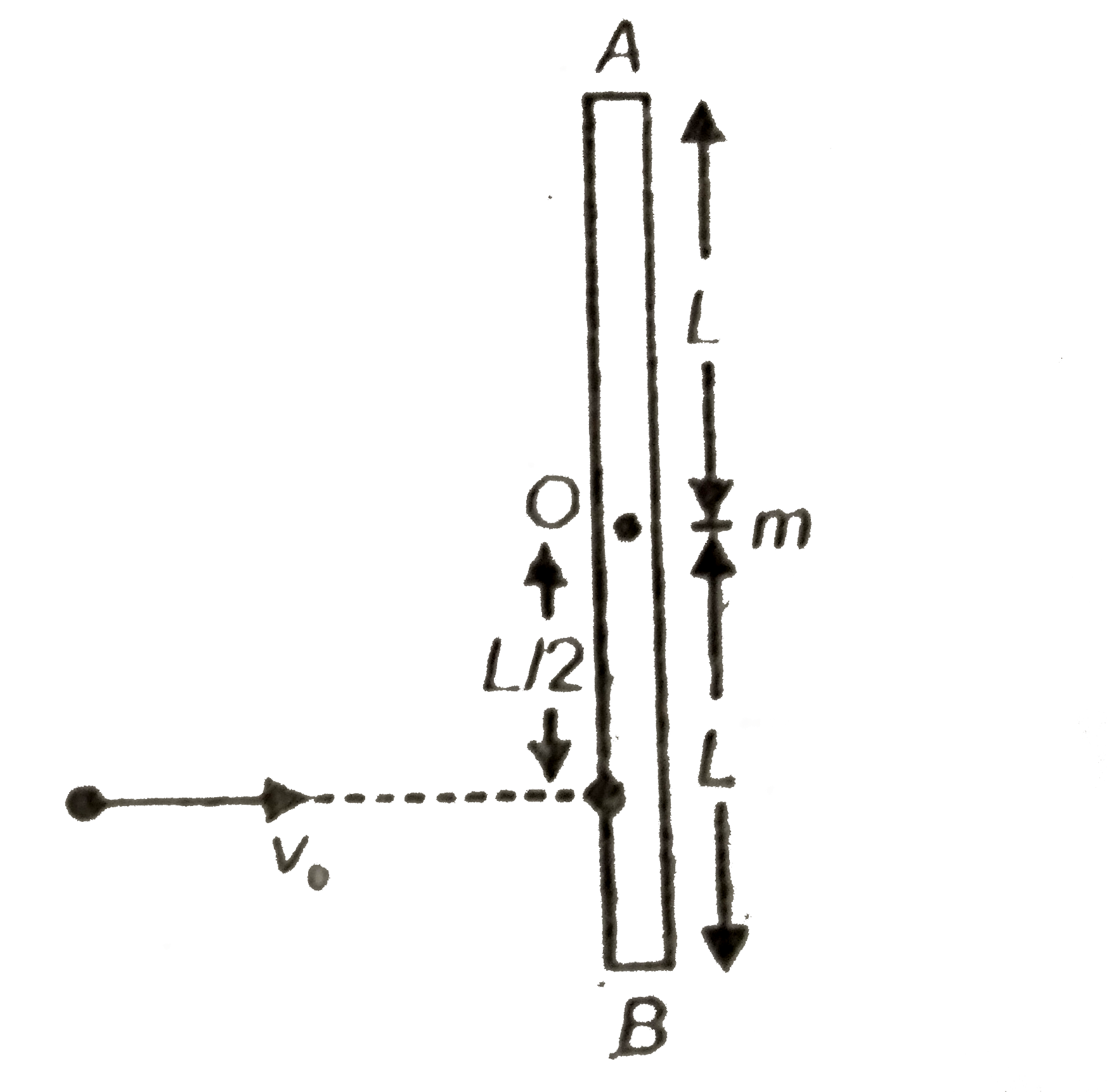
Distance of point P on rod from B which is at rest immediately afte collision is
View SolutionA ball is projected with a velocity 20√3ms−1 at angle 60∘ to the horizontal. The time interval after which the velocity vector will make an angle 30∘ to the horizontal is (Take, g=10ms−2)
View SolutionA particle is projected with a speed 10√2ms−1 and at an angle 45∘ with the horizontal. The rate of change of speed with respect to time at t=1s is (g=10ms−2)
View SolutionA ball is thrown at a speed of 40 m/s at an angle of 600 with the horizontal. Find a. the maximum height reached and b. the range of te ball. Take g=10ms2.
View SolutionThe ceiling of a tunnel is 5 m high. What is the maximum horizontal distance that a ball thrown with a speed of 20ms−1 can go without hitting the ceiling of the tunnel (g=10ms−2)
View SolutionTwo projectiles A and B are projected with same speed at an angle 30∘ and 60∘ to the horizontal, then which of the following is not valid where T is total time of flight, H is max height and R is horizontal range.
View SolutionA ball is thrown upwards from the ground with an initial speed of u. The ball is at height of 80m at two times, the time interval being 6 s. Find u. Take g=10m/s2.
View Solution
