Two particle are projected with same initial velocities at an angle 30∘ and 60∘ with the horizontal .Then
Method 1 : Foe bullet A. Let t be the time taken by bullet A to reach P
Vertical motion : uy=0,S=ut+12at2,Sy=−(10−y)
ay=−10ms−2⇒10−y=5t2 …(i)
Horizontal motion : x=5√3t …(ii)
For bullet B. Let (t+l) be the time taken by bullet B to reach P.
Vertical motion : ↑⏐⏐+uy=+5√3sin60∘=+7.5ms−1
S=ut+12at2,ay=−10ms−2
y−10=7.5(t+t')−5(t+t')2 ...(iii)
Horizontal motion : x=(5√3cos60∘)(t+t')
⇒5√3t+5√3t'=2x ...(iv)
Substituting the value of x from (ii) in (iv), we get
5√3t+5√3t'=10√3)t⇒t=t'
(a) Putting t=t' in (iii),
y−10=15t−20t2 ...(v)
Adding (i) and (v), 0=15t−15t2⇒t=1s
So time interval between the firings is t'=1s
(b) Putting t−1 in (ii), we get x=s√3m.
Putting t=1 in (i), we get y=5.
Therefore, the coordinates of point
Pare(5(√3,5)) in meters.
Method 2 : We take the point of firing as origin and x−andy - axis as shown in (Fig. 5.174). Equation of trajectory of the projectile is
y=xtanθ−gx22v2icos2θ
For gun 1,θ=60∘. Therefore,
y=xtan60∘−gx22v2icos260∘=x√3−2gx2v2i
For gun 2, θ=0∘. Therefore,
y=−gx22v2i
Two shots collide at point P. Therefore, their coordinates must be same.
−gx22v2i=x√3−2gx22v2i
x√3=2gx2v2i−gx22v2i=3gx22v2i
x=0 and x=2v2i√3g=2(5√3)2√3(10)=5√3
y=−gx22v2i=−10(5√3)22(5√3)2=−5m
If the origin is assigned at ground, the coordinates of point P will be (5√3)m,5m).
Now we consider the x - component of displacement for both the shots.
Gun 1 : x=5√3m=vit(5√3ms−1)t
or t1=1s
Gun 2 : x=5√3m=vicos60∘t2=5√32t2
or t2=2s
Time interval between two shots, Δt=t2−t1=1s.
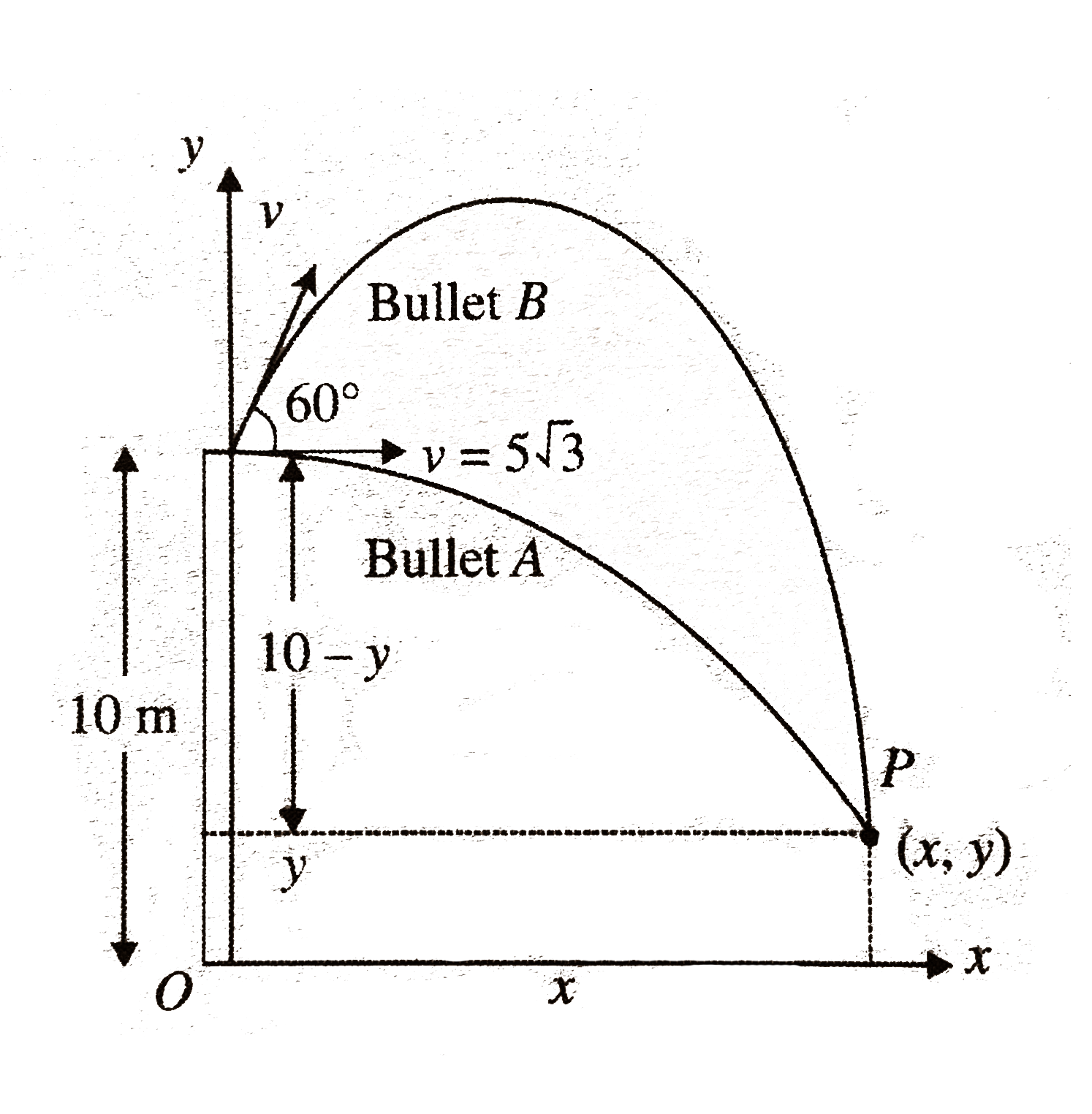 ,
, .
.
Topper's Solved these Questions
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.1
15 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.2
25 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer
9 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
Similar Questions
Two projectiles A and B are projected with same speed at an angle 30∘ and 60∘ to the horizontal, then which of the following is not valid where T is total time of flight, H is max height and R is horizontal range.
View SolutionTwo projectiles of same mass and with same velocity are thrown at an angle 60∘ and 30∘ with the horizontal, then which quantity will remain same:-
View SolutionTwo particles are projected with same initial velocity one makes angle θ with horizontal while other makes an angle θ with vertical. If their common range is R then product of their time of flight is directly proportional to :
View SolutionTwo bodies of same mass are projected with the same velocity at an angle 30∘ and 60∘ respectively.The ration of their horizontal ranges will be
View SolutionTwo particles are projected with same velocity but at angles of projection 25° and 65° with horizontal. The ratio of their horizontal ranges is
View SolutionTwo particles are projected with same velocity but at angles of projection 35° and 55°. Then their horizontal ranges are in the ratio of
View SolutionA particle A is projected from the ground with an initial velocity of 10m/s at an angle of 60∘ with horizontal. From what height should an another particle B be projected horizontally with velocity 5m/s so that both the particles collide in ground at point C if both are projected simultaneously g=10m/s2.

View SolutionA particle A is projected with an initial velocity of 60m/s at an angle 30∘ to the horizontal. At the same time a second particle B is projected in opposite direction with initial speed of 50m/s from a point at a distance of 100 m from A. If the particles collide in air, find (a)the angle of projection α of particle B, (b) time when the collision takes place and (c) the distance of P from A, where collision occurs. (g=10m/s2)
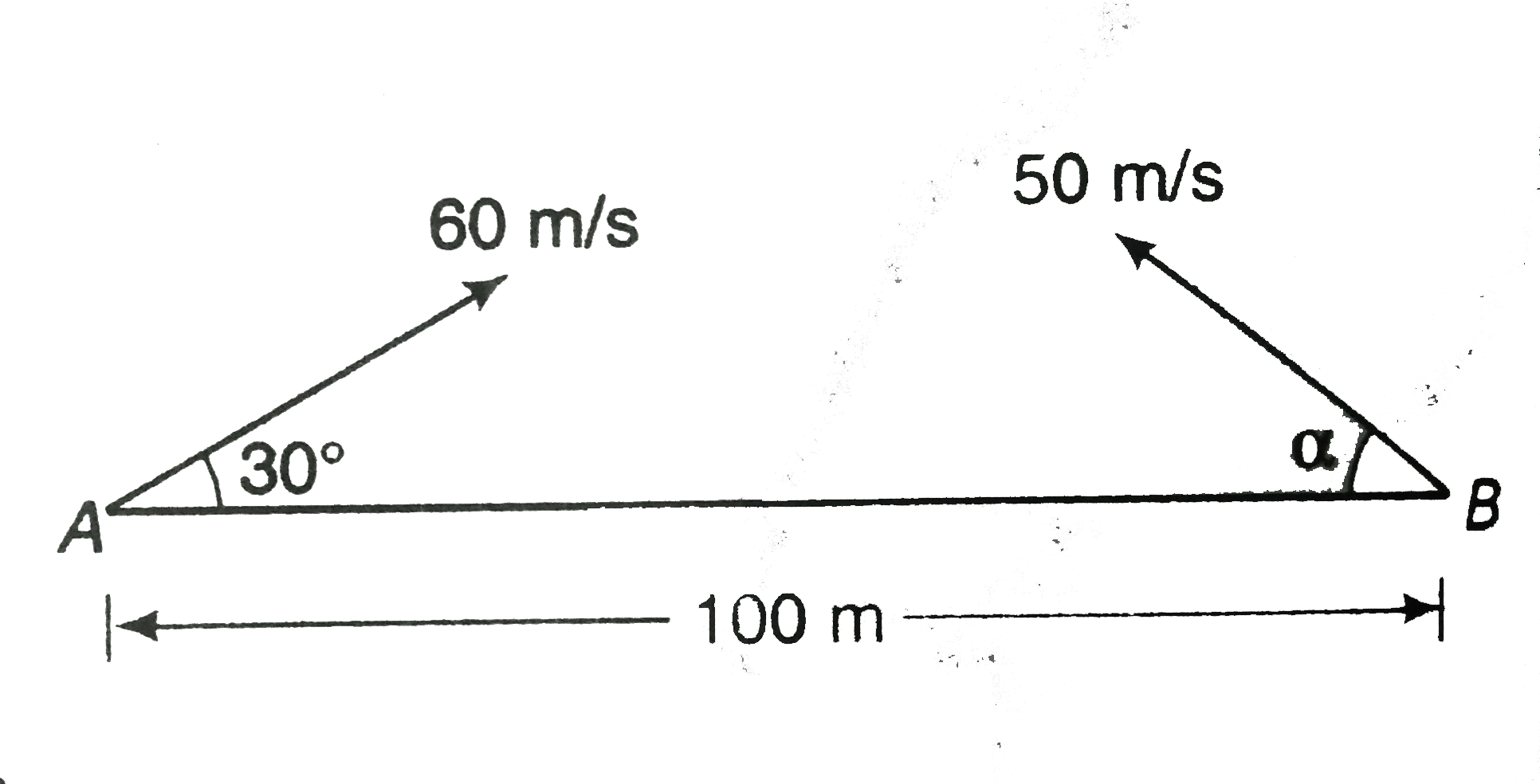
View SolutionA particle is projected from ground with an initial velocity 20m/sec making an angle 60∘ with horizontal . If R1 and R2 are radius of curvatures of the particle at point of projection and highest point respectively, then find the value of R1R2.
View SolutionFour projectiles are projected with the same speed at angles 20∘,35∘,60∘and75∘
with the horizontal. The range will be the maximum for the projectile whose angle of projection is
View Solution
