A block slides down a smooth inclined placne when released from the top, while another falls freely from the same point. Which of the following is/are correct ?
Sliding block will reach the ground first
Freely falling block will reach the ground first.
Both the blocks will reach the ground with different speeds
Both the block will reach the ground with same speed .
The correct Answer is:B, D
To solve the problem, we need to analyze the motion of both blocks: one sliding down an inclined plane and the other falling freely from the same height.
1. Identify the Setup:
- We have an inclined plane with an angle θ and a height h from which both blocks are released.
- Block 1 slides down the incline, and Block 2 falls freely.
2. Determine the Distance Traveled by Each Block:
- For Block 1 (the one on the incline), the distance L it travels can be related to the height h by the sine of the angle θ:
sin(θ)=hL⟹L=hsin(θ)
- Since θ is less than 90 degrees, sin(θ) is less than 1, which means:
L>h
- Block 2, which falls freely, travels a vertical distance of h.
3. Compare the Distances:
- Since L (the distance Block 1 travels) is greater than h (the distance Block 2 travels), Block 2 will reach the ground first because it has a shorter distance to cover.
4. Determine the Speeds of Both Blocks:
- We can use the principle of conservation of energy to find the speed of both blocks when they reach the ground.
- For Block 1 (the inclined plane):
Potential Energy lost=Kinetic Energy gained
mgh=12mv21⟹v1=√2gh
- For Block 2 (the free fall):
mgh=12mv22⟹v2=√2gh
- Thus, both blocks will have the same speed when they reach the ground.
5. Conclusion:
- Block 2 (the freely falling block) reaches the ground first.
- Both blocks reach the ground with the same speed.
Final Answers:
- Block 2 reaches the ground first (True).
- Both blocks reach the ground with the same speed (True).
- We have an inclined plane with an angle θ and a height h from which both blocks are released.
- Block 1 slides down the incline, and Block 2 falls freely.
- For Block 1 (the one on the incline), the distance L it travels can be related to the height h by the sine of the angle θ:
sin(θ)=hL⟹L=hsin(θ)
- Since θ is less than 90 degrees, sin(θ) is less than 1, which means:
L>h
- Block 2, which falls freely, travels a vertical distance of h.
- Since L (the distance Block 1 travels) is greater than h (the distance Block 2 travels), Block 2 will reach the ground first because it has a shorter distance to cover.
- We can use the principle of conservation of energy to find the speed of both blocks when they reach the ground.
- For Block 1 (the inclined plane):
Potential Energy lost=Kinetic Energy gained
mgh=12mv21⟹v1=√2gh
- For Block 2 (the free fall):
mgh=12mv22⟹v2=√2gh
- Thus, both blocks will have the same speed when they reach the ground.
- Block 2 (the freely falling block) reaches the ground first.
- Both blocks reach the ground with the same speed.
- Block 2 reaches the ground first (True).
- Both blocks reach the ground with the same speed (True).
Topper's Solved these Questions
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Assertion-reasoning
5 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Linked Comprehension
30 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Graphical cancept
1 Video
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Video
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer
9 Videos
Similar Questions
A block slides down a smooth inclined plane and then moves on to a rough horizontal surface. Which of the following is/are correct? (Neglect impulsive effect at the bottom of incline)

View SolutionA block of mass m is directly pulled up slowly on a smooth inclined plane of height h and inclination θ with the help of a string parallel to the incline. Which of the following statement is incorrect for the block when it moves up from the bottom to the top the incline? .
 .
.
View Solution
Knowledge Check
A block mass 3 kg slides down a frictionless inclined plane of length 6 m and height 4 m. if the block is released from rest at the top of the incline, what is its speed at the bottom?
A5m/sB6m/sC8m/sD9m/sA body sliding on a smooth inclined plane requires 4s to reach the bottom, starting from rest at the at the top. How much time does it take to cover ont-foruth the distance startion from rest at the top?
A1 sB4 sC2 sD16 s
A sphere is released on a smooth inclined plane from the top. When it moves down, its angular momentum is
View SolutionSTATEMENT-1: A ball of mass m is released from the top of a smooth inclined plane. At the same time another ball of same mass allowed to fall freely from same height. When both balls reaches the bottom of the plane, momentum will not be equal
because
STATEMENT-2: Momentum depends on the direction of velocity as well as its magnitude.
View Solution(a) Show that the velocity acquired by a particle in sliding down an inclined plane is the same as that acquired by a particle falling freely from frst though a distance equal to the height of the inclined plane. (b) Find the time taken in sliding a particle down the whole length of the incline.
View SolutionA body of mass m slides down an smooth incline and reaches the bottom with a velocity, Now smooth incline surface is made rough and the same mass was in the form of a ring which rolls down this incline, the velocity of the ring at the bottom would have been:
View SolutionA block is released from the top of a smooth inclined plane of inclination θ as shown in figure. Let υ be the speed of the particle after travelling a distance s down the plane. Then which of the following will remain constant ?
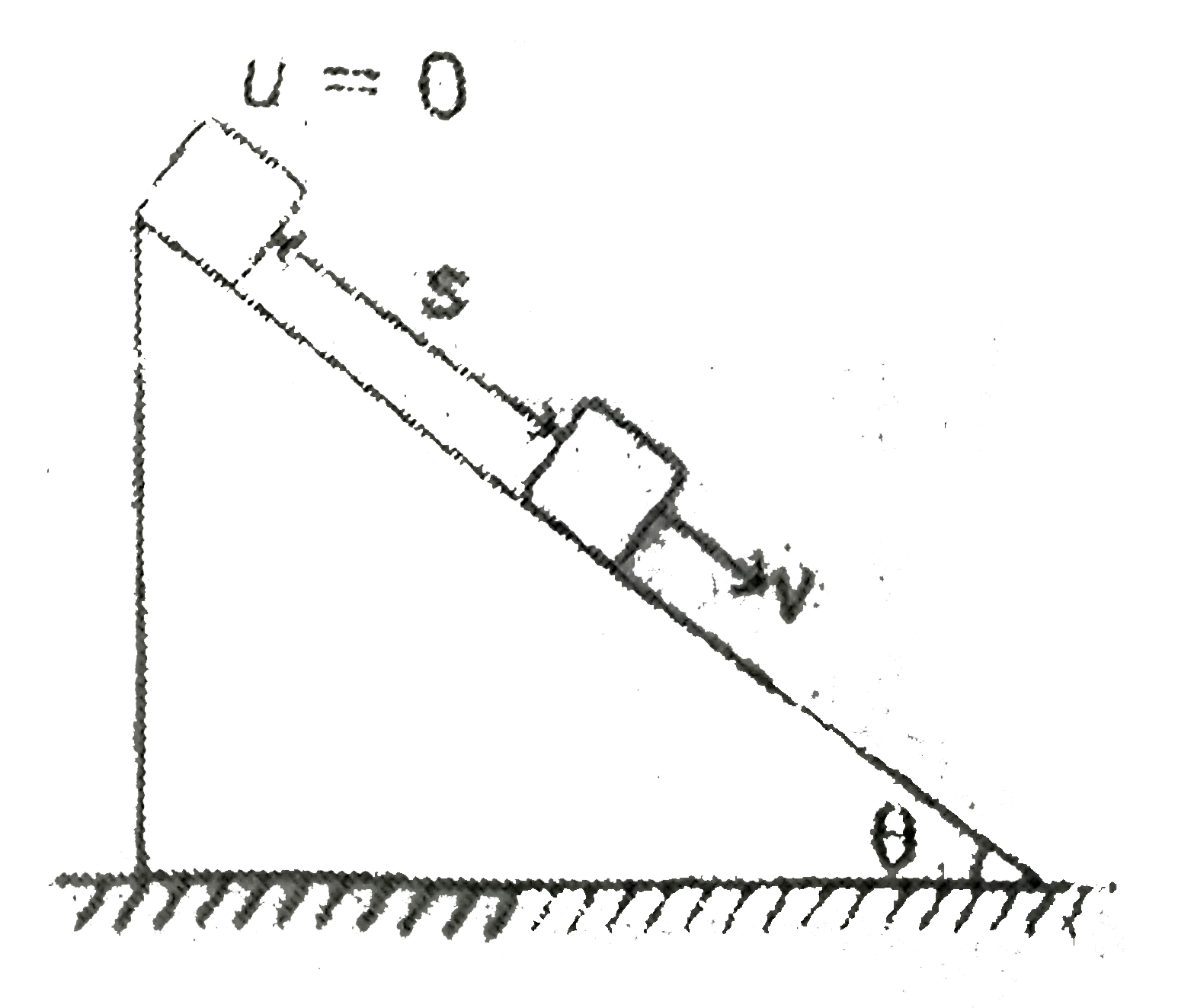
View SolutionA block slides down from top of a smooth inclined plane of elevation ● fixed in an elevator going up with an acceleration a0 The base of incline hs length L Find the time taken by the block to reach the bottom
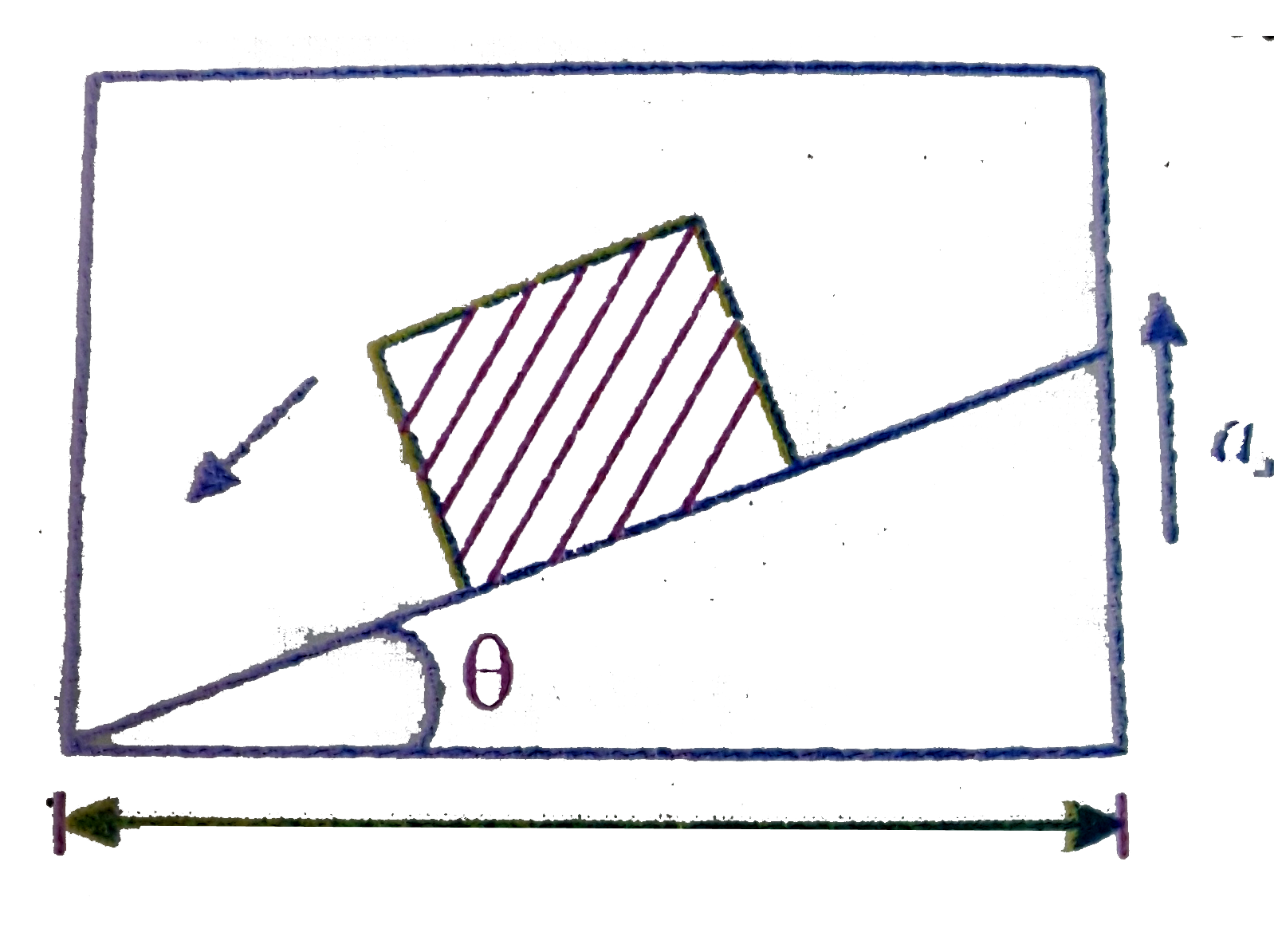 .
.
View Solution
