A body of mass m slides down an smooth incline and reaches the bottom with a velocity, Now smooth incline surface is made rough and the same mass was in the form of a ring which rolls down this incline, the velocity of the ring at the bottom would have been:
√2v
v
(√25)v
v/√2
The correct Answer is:D
To solve the problem of a ring rolling down a rough incline, we can follow these steps:
Step 1: Understand the Initial Conditions
When a body of mass m slides down a smooth incline, it reaches the bottom with a certain velocity. The velocity can be derived from energy conservation principles. The potential energy at the top is converted into kinetic energy at the bottom.
Step 2: Calculate the Velocity on a Smooth Incline
For a smooth incline, the potential energy (PE) at height h is given by:
\( PE = mgh \)
The kinetic energy (KE) at the bottom is:
\( KE = \frac{1}{2} mv^2 \)
By conservation of energy:
\( mgh = \frac{1}{2} mv^2 \)
This simplifies to:
\( v^2 = 2gh \)
Thus, the velocity \( v \) at the bottom of the incline is:
\( v = \sqrt{2gh} \)
Step 3: Transition to a Rough Incline with a Ring
When the incline is made rough and the mass is in the form of a ring, the ring rolls down the incline. The forces acting on the ring include gravitational force, normal force, and friction.
Step 4: Analyze Forces on the Ring
The gravitational force acting down the incline is:
\( F_{\text{gravity}} = mg \sin \theta \)
The frictional force provides the torque necessary for rolling. The equation of motion for the ring can be expressed as:
\( mg \sin \theta - F_{\text{friction}} = ma \)
Where \( a \) is the linear acceleration of the center of mass of the ring.
Step 5: Relate Linear and Angular Acceleration
For a ring, the moment of inertia \( I \) is given by:
\( I = mR^2 \)
The frictional force \( F_{\text{friction}} \) also contributes to the angular acceleration \( \alpha \):
\( F_{\text{friction}} \cdot R = I \alpha \)
Since \( \alpha = \frac{a}{R} \), we can substitute:
\( F_{\text{friction}} \cdot R = mR^2 \cdot \frac{a}{R} \)
Thus:
\( F_{\text{friction}} = \frac{ma}{R} \)
Step 6: Substitute Back into the Equation of Motion
Now substituting \( F_{\text{friction}} \) into the equation of motion:
\( mg \sin \theta - \frac{ma}{R} = ma \)
This simplifies to:
\( mg \sin \theta = ma + \frac{ma}{R} \)
Factoring out \( m \):
\( g \sin \theta = a \left(1 + \frac{1}{R}\right) \)
Step 7: Solve for Acceleration
Now, we can express the acceleration \( a \):
\( a = \frac{g \sin \theta}{1 + \frac{1}{R}} \)
Step 8: Calculate the Final Velocity
Using the kinematic equation \( v^2 = u^2 + 2as \) (where initial velocity \( u = 0 \)):
\( v^2 = 0 + 2as \)
Substituting for \( a \):
\( v^2 = 2s \cdot \frac{g \sin \theta}{1 + \frac{1}{R}} \)
Thus, the final velocity \( v \) at the bottom of the incline is:
\( v = \sqrt{\frac{2gs \sin \theta}{1 + \frac{1}{R}}} \)
Step 9: Compare with the Smooth Incline
From the smooth incline case, we had:
\( v_{\text{smooth}} = \sqrt{2gs \sin \theta} \)
Thus, the ratio of the velocities is:
\( \frac{v_{\text{ring}}}{v_{\text{smooth}}} = \frac{\sqrt{\frac{2gs \sin \theta}{1 + \frac{1}{R}}}}{\sqrt{2gs \sin \theta}} = \frac{1}{\sqrt{1 + \frac{1}{R}}} \)
Conclusion
The final velocity of the ring at the bottom of the rough incline is given by:
\( v_{\text{ring}} = \frac{v_{\text{smooth}}}{\sqrt{1 + \frac{1}{R}}} \)
When a body of mass m slides down a smooth incline, it reaches the bottom with a certain velocity. The velocity can be derived from energy conservation principles. The potential energy at the top is converted into kinetic energy at the bottom.
For a smooth incline, the potential energy (PE) at height h is given by:
\( PE = mgh \)
The kinetic energy (KE) at the bottom is:
\( KE = \frac{1}{2} mv^2 \)
By conservation of energy:
\( mgh = \frac{1}{2} mv^2 \)
This simplifies to:
\( v^2 = 2gh \)
Thus, the velocity \( v \) at the bottom of the incline is:
\( v = \sqrt{2gh} \)
When the incline is made rough and the mass is in the form of a ring, the ring rolls down the incline. The forces acting on the ring include gravitational force, normal force, and friction.
The gravitational force acting down the incline is:
\( F_{\text{gravity}} = mg \sin \theta \)
The frictional force provides the torque necessary for rolling. The equation of motion for the ring can be expressed as:
\( mg \sin \theta - F_{\text{friction}} = ma \)
Where \( a \) is the linear acceleration of the center of mass of the ring.
For a ring, the moment of inertia \( I \) is given by:
\( I = mR^2 \)
The frictional force \( F_{\text{friction}} \) also contributes to the angular acceleration \( \alpha \):
\( F_{\text{friction}} \cdot R = I \alpha \)
Since \( \alpha = \frac{a}{R} \), we can substitute:
\( F_{\text{friction}} \cdot R = mR^2 \cdot \frac{a}{R} \)
Thus:
\( F_{\text{friction}} = \frac{ma}{R} \)
Now substituting \( F_{\text{friction}} \) into the equation of motion:
\( mg \sin \theta - \frac{ma}{R} = ma \)
This simplifies to:
\( mg \sin \theta = ma + \frac{ma}{R} \)
Factoring out \( m \):
\( g \sin \theta = a \left(1 + \frac{1}{R}\right) \)
Now, we can express the acceleration \( a \):
\( a = \frac{g \sin \theta}{1 + \frac{1}{R}} \)
Using the kinematic equation \( v^2 = u^2 + 2as \) (where initial velocity \( u = 0 \)):
\( v^2 = 0 + 2as \)
Substituting for \( a \):
\( v^2 = 2s \cdot \frac{g \sin \theta}{1 + \frac{1}{R}} \)
Thus, the final velocity \( v \) at the bottom of the incline is:
\( v = \sqrt{\frac{2gs \sin \theta}{1 + \frac{1}{R}}} \)
From the smooth incline case, we had:
\( v_{\text{smooth}} = \sqrt{2gs \sin \theta} \)
Thus, the ratio of the velocities is:
\( \frac{v_{\text{ring}}}{v_{\text{smooth}}} = \frac{\sqrt{\frac{2gs \sin \theta}{1 + \frac{1}{R}}}}{\sqrt{2gs \sin \theta}} = \frac{1}{\sqrt{1 + \frac{1}{R}}} \)
The final velocity of the ring at the bottom of the rough incline is given by:
\( v_{\text{ring}} = \frac{v_{\text{smooth}}}{\sqrt{1 + \frac{1}{R}}} \)
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 2Exercise:Multiple Correct
Explore 26 Videos
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 2Exercise:Linked Comprehension
Explore 71 Videos
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 2Exercise:Subjective
Explore 19 Videos
- RIGID BODY DYNAMICS 1Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 1Exercise:Integer
Explore 11 Videos
- SOUND WAVES AND DOPPLER EFFECTBook:CENGAGE PHYSICS ENGLISHChapter:SOUND WAVES AND DOPPLER EFFECTExercise:Integer
Explore 16 Videos
Similar Questions
A body of mass m slides down an incline and reaches the bottom with a velocity v. If the same mass were in the form of a ring which rolls down this incline, the velocity of the ring at the bottom would have been
View SolutionA block of mass m slides down a rough inclined plane with an acceleration g2

View Solution
Knowledge Check
A man goes at the top of a smooth inclined plane. He releases a bag to fall freely and himself slides down on inclined plane to reach the bottom. If u1andu2 are the respective velocities of the man and bag at the bottom of inclined plane, then
Au1>u2Bu1<u2Cu1=u2Du1andu2 cannot be compared
When a point mass slips down a smooth incline from top, it reaches the bottom with linear speed v. If same mass in the form of disc rolls down without slipping a rough incline of identical geometry through same distance, what will be its linear velocity at the bottom ?
View SolutionA body is released from the top of a smooth inclined plane of inclination θ. It reaches the bottom with velocity v. If the angle of inclina-tion is doubled for the same length of the plane, what will be the velocity of the body on reach ing the ground .
View SolutionIf a ring, a disc, a solid sphere and a cyclinder of same radius roll down an inclined plane, the first one to reach the bottom will be:
View SolutionA block of mass m slides down along the surface of the bowl from the rim to the bottom as shown in fig. The velocity of the block at the bottom will be-
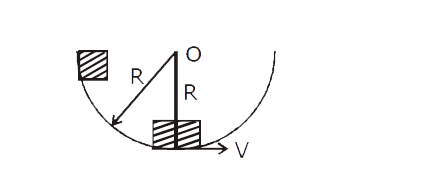
View SolutionA solid sphere and a solid cylinder having the same mass and radius, rolls down the same incline. The ratio of their acceleration will be
View SolutionA solid cylinder of mass M and radius R rolls down an inclined plane of height h. The angular velocity of the cylinder when it reaches the bottom of the plane will be :
View SolutionA block of mass 4 kg is pulled along a smooth inclined plane of inclination 30∘ with constant velocity 3 m/s as shown, power delivered by the force is
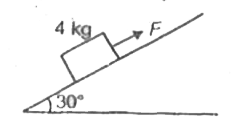
View Solution