The potential energy of a spring is the minimum when it is
compressed
extended
at its natural length
at its natural length but is kept at a height above the ground
The correct Answer is:C
To determine when the potential energy of a spring is at its minimum, we can follow these steps:
Step 1: Understand the formula for potential energy of a spring
The potential energy (PE) stored in a spring is given by the formula:
PE=12kx2
where:
- k is the spring constant (a measure of the spring's stiffness),
- x is the amount of extension or compression from the spring's natural length.
Step 2: Analyze the conditions for minimum potential energy
From the formula, we can see that the potential energy depends on the square of x. The value of x can be either positive (extension) or negative (compression).
Step 3: Determine when x is at its minimum
The potential energy will be minimized when x is at its minimum value. Since x represents the amount of extension or compression, the minimum value of x is:
x=0
This means that the spring is neither compressed nor extended.
Step 4: Relate x=0 to the spring's natural length
When x=0, the spring is at its natural length. This is the position where the spring is in its equilibrium state, and no external forces are acting on it to compress or extend it.
Step 5: Conclusion
Thus, the potential energy of a spring is at its minimum when the spring is at its natural length.
Final Answer
The potential energy of a spring is the minimum when it is at its natural length (Option 3).
---
The potential energy (PE) stored in a spring is given by the formula:
PE=12kx2
where:
- k is the spring constant (a measure of the spring's stiffness),
- x is the amount of extension or compression from the spring's natural length.
From the formula, we can see that the potential energy depends on the square of x. The value of x can be either positive (extension) or negative (compression).
The potential energy will be minimized when x is at its minimum value. Since x represents the amount of extension or compression, the minimum value of x is:
x=0
This means that the spring is neither compressed nor extended.
When x=0, the spring is at its natural length. This is the position where the spring is in its equilibrium state, and no external forces are acting on it to compress or extend it.
Thus, the potential energy of a spring is at its minimum when the spring is at its natural length.
The potential energy of a spring is the minimum when it is at its natural length (Option 3).
Topper's Solved these Questions
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:OBJECTIVE QUESTIONS FROM PREVIOUS IAS EXAMINATIONS
Explore 50 Videos
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:WAVES
Explore 98 Videos
- CIRCULAR MOTION Book:ICSEChapter:CIRCULAR MOTION Exercise:MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
Explore 24 Videos
- DIMENSIONSBook:ICSEChapter:DIMENSIONSExercise:SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)
Explore 9 Videos
Similar Questions
If the potential energy of a spring is V on stretching it by 2cm, then its potential energy when it is stretched by 10cm will be
View SolutionAssuming that potential energy of spring is zero when it is stretched by x02, its potential energy when it is compressed by x0 is
View SolutionIn figure, k=100N/m,M=1kg and F=10N
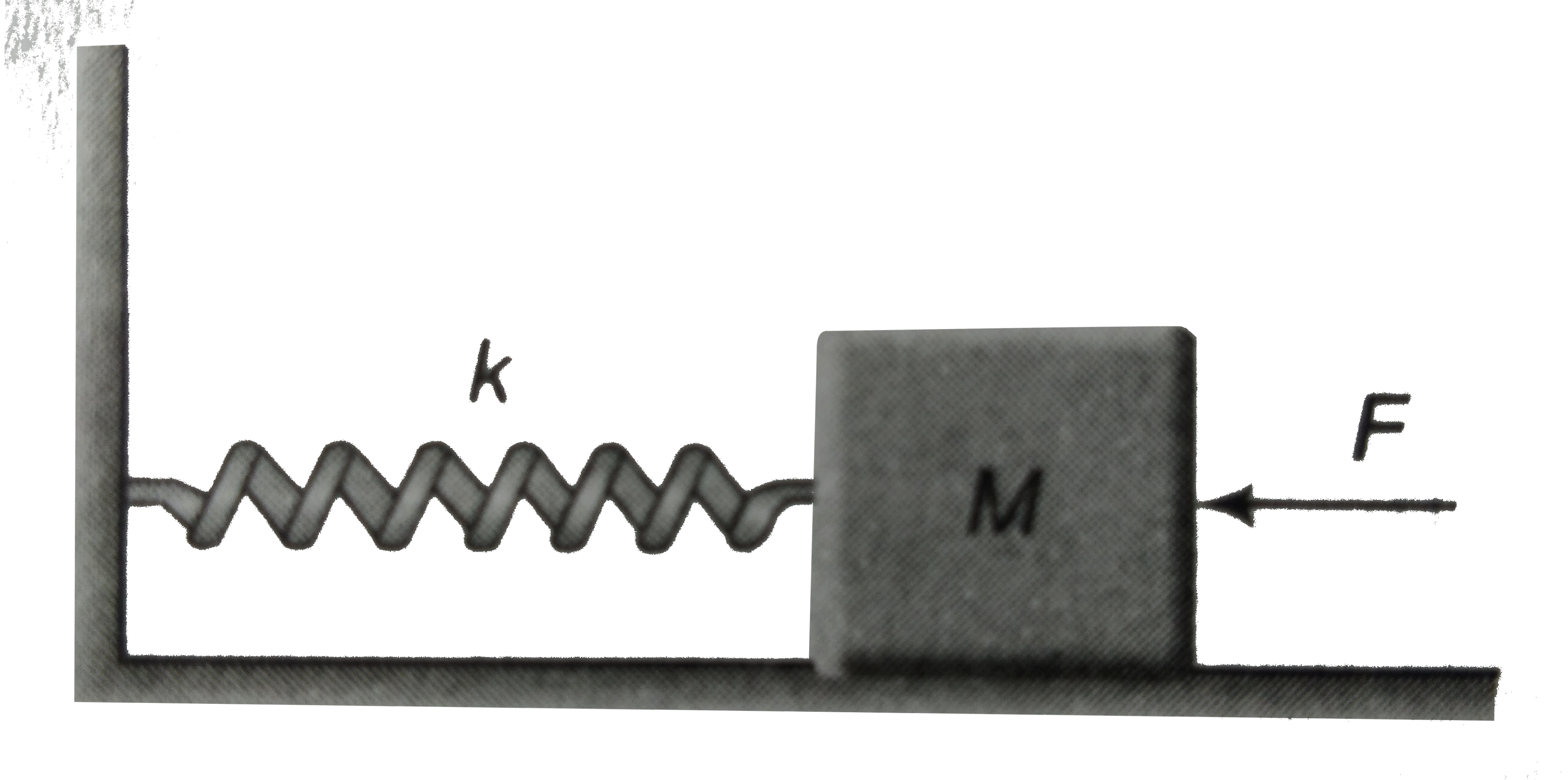
(a) Find the compression of the spring in the equilibrium position
(b) A sharp blow by some external agent imparts a speed of 2m/s to the block towards left. Find the sum of the potential energy of the spring and the kinetic energy of the block at this instant.
(c) Find the time period of the resulting simple harmonic motion.
(d) Find the amplitude.
(e) Write the potential energy of the spring when the block is at the left estreme.
(f) Write the potential energy of the spring when the block is at the right extreme.
The answers of (b), (e) and (f) are different. Explain why this does not violate the principle of conservation of energy ?
View SolutionWhy is the potential energy of the system minimum at the stage of formation of a covalent bond?
View SolutionA : The potential energy of a system increases when work is done by conservative force.
R : Kinetic energy can change into potential energy and vice-versa.
View SolutionSTATEMENT-1: If potential energy of a dipole in stable equilibrium position is zero, its potential energy is unstable equilibrium position will be 2pE, where p and E represent the dipole moment and electric field respectively
and
STATEMENT-2 : The potential energy of a dipole is minimum in stable equilibruim position and maximum in unstable equilibrium position.
View SolutionThe potential energy of a long spring when stretched by 2 cm is U. If the spring is stretched by 8 cm the potential energy stored in it is:-
View SolutionThe potential energy of a harmonic oscillation when is half way to its and end point is (where E it’s the total energy)
View SolutionA block of mass m is initially moving to the right on a horizontal frictionless surface at a speed v. It then compresses a spring of spring constant k. At the instant when the kinetic energy of the block is equal to the potential energy of the spring, the spring is compressed a distance of :
View SolutionIn case of an ideal spring, we take the reference point (where potential energy is assumed to be zero) at extension of x0 instead of taking at natural length. Then potential energy when spring is extended by x is :
View Solution