A ball weighing 4 kg and moving with velocity of 8 m/sec. Collides with a stationary ball of mass 12 kg. After the collision, the two balls move together. The final velocity is
0
2 m/sec
8 m/sec
16 m/sec
The correct Answer is:B
To solve the problem step by step, we will use the principle of conservation of momentum.
Step 1: Identify the given data
- Mass of the first ball (m1) = 4 kg
- Initial velocity of the first ball (u1) = 8 m/s
- Mass of the second ball (m2) = 12 kg
- Initial velocity of the second ball (u2) = 0 m/s (since it is stationary)
Step 2: Write the formula for conservation of momentum
According to the law of conservation of momentum:
Initial Momentum=Final Momentum
Step 3: Calculate the initial momentum
The initial momentum (p_initial) can be calculated as:
pinitial=m1⋅u1+m2⋅u2
Substituting the values:
pinitial=(4kg⋅8m/s)+(12kg⋅0m/s)
pinitial=32kg m/s+0
pinitial=32kg m/s
Step 4: Calculate the final momentum
After the collision, both balls stick together and move with a common velocity (v). The total mass after the collision is:
mtotal=m1+m2=4kg+12kg=16kg
The final momentum (p_final) can be expressed as:
pfinal=mtotal⋅v
So,
pfinal=16kg⋅v
Step 5: Set initial momentum equal to final momentum
Using the conservation of momentum:
pinitial=pfinal
32kg m/s=16kg⋅v
Step 6: Solve for the final velocity (v)
To find v, we rearrange the equation:
v=32kg m/s16kg
v=2m/s
Conclusion
The final velocity of the two balls moving together after the collision is 2 m/s.
---
- Mass of the first ball (m1) = 4 kg
- Initial velocity of the first ball (u1) = 8 m/s
- Mass of the second ball (m2) = 12 kg
- Initial velocity of the second ball (u2) = 0 m/s (since it is stationary)
According to the law of conservation of momentum:
Initial Momentum=Final Momentum
The initial momentum (p_initial) can be calculated as:
pinitial=m1⋅u1+m2⋅u2
Substituting the values:
pinitial=(4kg⋅8m/s)+(12kg⋅0m/s)
pinitial=32kg m/s+0
pinitial=32kg m/s
After the collision, both balls stick together and move with a common velocity (v). The total mass after the collision is:
mtotal=m1+m2=4kg+12kg=16kg
The final momentum (p_final) can be expressed as:
pfinal=mtotal⋅v
So,
pfinal=16kg⋅v
Using the conservation of momentum:
pinitial=pfinal
32kg m/s=16kg⋅v
To find v, we rearrange the equation:
v=32kg m/s16kg
v=2m/s
The final velocity of the two balls moving together after the collision is 2 m/s.
Topper's Solved these Questions
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - OBJECTIVE QUESTIONS FROM PREVIOUS IAS EXAMINATIONS
50 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - WAVES
98 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
24 Videos
- DIMENSIONSBOOK - ICSECHAPTER - DIMENSIONSEXERCISE - SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)
9 Videos
Similar Questions
A metal ball of mass 2 kg moving with a velocity of 36km/h has a head on collision with a stationery ball of mass 3 kg. If after the collision, the two balls move together, the loss in kinetic energy dur to collision is
View SolutionA ball of mass 1 kg moving with a velocity of 0.4 ms−1 collides with another stationary ball. After the collision, the first ball moves with a velocity of 0.3ms1 in a direction making an angle of 90∘ with its initial direction. The momentum of the second ball after the collision will be (in kg ms−1)
View SolutionA ball of mass 0.50 kg moving at a speed of 5.0 m/s collides with another ball of mas 1.0 kg. After the collision the balls stick together ane remain motionless. What was the velocity of the 1.0 kg block before the collision?
View SolutionA body of mass 10 kg and velocity 10 m/s collides with a stationary body of mass 5 kg . After collision both bodies stick to each other , velocity of the bodies after collision will be
View SolutionA smooth ball 'A' moving with velocity 'V' collides with another smooth identical ball at rest. After collision both the balls move with same speed with angle between their velocities 60∘. No external force acts on the system of balls.

The speed of each ball after the collision is
View SolutionA smooth ball 'A' moving with velocity 'V' collides with another smooth identical ball at rest. After collision both the balls move with same speed with angle between their velocities 60∘. No external force acts on the system of balls.

The value of coefficient of resititution is
View SolutionA smooth ball 'A' moving with velocity 'V' collides with another smooth identical ball at rest. After collision both the balls move with same speed with angle between their velocities 60∘. No external force acts on the system of balls.
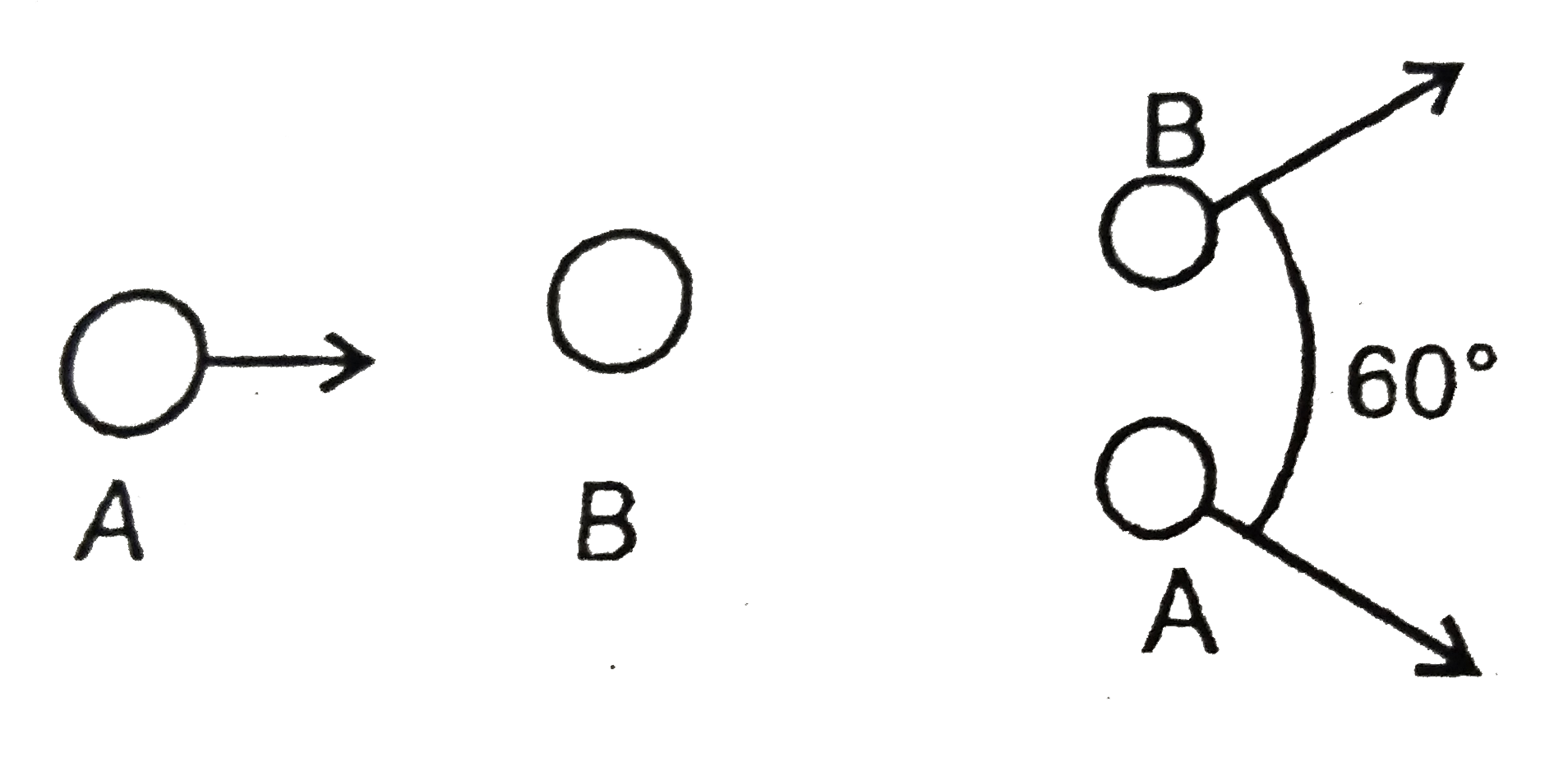
If the kinetic energy lost is fully converted to heat then heat produced is
View SolutionA ball of mass 5 kg moving with speed 8 m/s collides head on with another stationary ball of mass 15 kg. If collision is perfectly inelastic, then loss in kinetic energ is
View SolutionA ball of mass '2m' moving with velocity uˆi collides with another ball of mass 'm' moving with velocity −2uˆi. After the collision, mass 2m moves with velocity u2(ˆi−ˆj). Then the change in kinetic energy of the system ( both balls ) is
View SolutionA ball moving with velocity 2ms−1 collides head on with another stationary ball of double the mass. If the coefficient of restitution is 0.5, then their velocities (in ms−1) after collision will be
View Solution
