Just before striking the ground a 5.0 kg body has a kinetic energy of 980 J. IF friction is ignored, from what height was it dropped ?
9.8 m
50 m
20.0 m
24 m
The correct Answer is:A
To solve the problem, we will use the principle of conservation of energy, which states that the potential energy (PE) at the height from which the body is dropped is converted into kinetic energy (KE) just before it strikes the ground.
1. Identify the Given Values:
- Mass of the body (m) = 5.0 kg
- Kinetic energy just before striking the ground (KE) = 980 J
- Acceleration due to gravity (g) = 9.8 m/s² (standard value)
2. Write the Formula for Kinetic Energy:
The kinetic energy (KE) of an object is given by the formula:
KE=12mv2
However, in this case, we will relate kinetic energy to potential energy.
3. Write the Formula for Potential Energy:
The potential energy (PE) at height (h) is given by:
PE=mgh
4. Apply Conservation of Energy:
Since we are ignoring friction, the kinetic energy just before hitting the ground is equal to the potential energy at the height from which it was dropped:
KE=PE
Therefore:
980J=mgh
5. Substitute the Known Values:
Substitute the values of mass (m = 5 kg) and acceleration due to gravity (g = 9.8 m/s²) into the equation:
980=5⋅9.8⋅h
6. Solve for Height (h):
Rearranging the equation to solve for h:
h=9805⋅9.8
Calculate the denominator:
5⋅9.8=49
Now substitute back:
h=98049
Performing the division:
h=20 meters
Final Answer:
The height from which the body was dropped is 20 meters.
- Mass of the body (m) = 5.0 kg
- Kinetic energy just before striking the ground (KE) = 980 J
- Acceleration due to gravity (g) = 9.8 m/s² (standard value)
The kinetic energy (KE) of an object is given by the formula:
KE=12mv2
However, in this case, we will relate kinetic energy to potential energy.
The potential energy (PE) at height (h) is given by:
PE=mgh
Since we are ignoring friction, the kinetic energy just before hitting the ground is equal to the potential energy at the height from which it was dropped:
KE=PE
Therefore:
980J=mgh
Substitute the values of mass (m = 5 kg) and acceleration due to gravity (g = 9.8 m/s²) into the equation:
980=5⋅9.8⋅h
Rearranging the equation to solve for h:
h=9805⋅9.8
Calculate the denominator:
5⋅9.8=49
Now substitute back:
h=98049
Performing the division:
h=20 meters
The height from which the body was dropped is 20 meters.
Topper's Solved these Questions
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - OBJECTIVE QUESTIONS FROM PREVIOUS IAS EXAMINATIONS
50 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - WAVES
98 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
24 Videos
- DIMENSIONSBOOK - ICSECHAPTER - DIMENSIONSEXERCISE - SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)
9 Videos
Similar Questions
A ball of mass 20 g falls from a height of 10 m and after striking the ground.It rebounds from the ground to a height of 8 m .
(a) Calculate:
(i)The kinetic energy of the ball just before striking the ground ,and
(ii)The loss in kinetic energy of the ball on striking the ground,and
(ii)the loss in kinetic energy of the ball on striking the ground.
(b)What happens to the loss in kinetic energy in part(ii) ?
Take g=10 m s−2 Neglect air friction .
View SolutionA ball of mass 10 g falls from a height of y 5 m. It rebounds from the ground to a height of 4m. Find :
(a) the initial potential energy of the ball, (b) the kinetic energy of the ball just before the ground. striking the ground,
(c) the kinetic energy of the ball after striking the ground ,and
(d) the loss in kinetic energy on striking the ground .
Take g=9.8 m s−2.
View Solution
Knowledge Check
A body dropped from a height h with initial velocity zero, strikes the ground with a velocity 3 m/s. Another body of same mass dropped from the same height h with an initial velocity of 4m/s. Find the final velocity of second mass with which it strikes the ground.
A3ms−1B4ms−1C5ms−1D12ms−1
The only force acting on a 2.0−kg body as it moves along the x-axis varies as shown in figure. The velocity of the body at x=0 is 4.0ms−1.
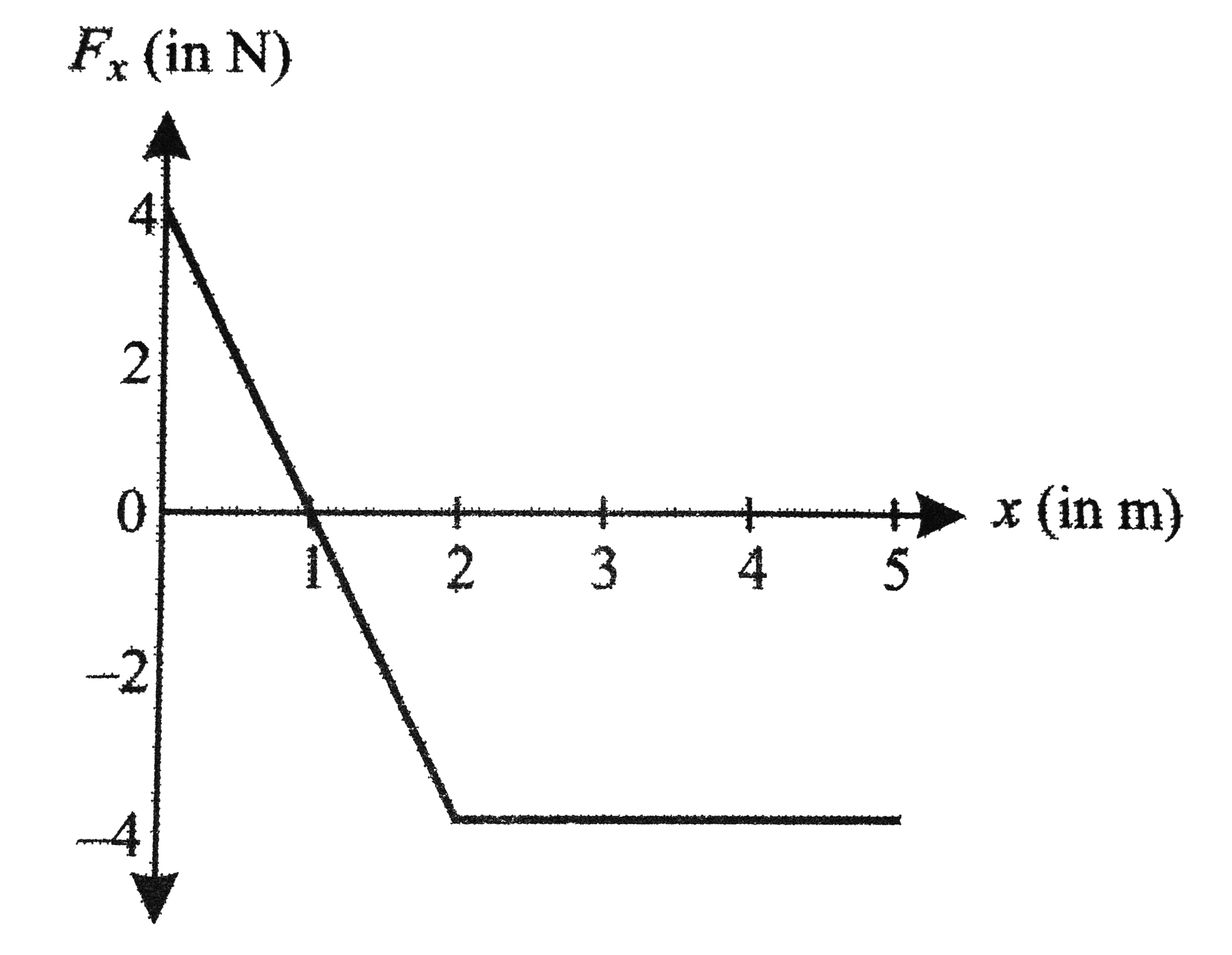
a. What is the kinetic energy of the body at x=3.0m?
b. At what value of x will the body have a kinetic energy of 8.0J?
c. What is the maximum kinetic energy attained by the body between x=0 and x=5.0m?
View SolutionA rock of mass m is dropped to the ground from a height h. A second rock, with mass 2m, is dropped from the same height. When the second rock strikes the ground, what is its kinetic energy? (a) Twice that of the first rock, (b) four times that of the first rock, (c) same as that of the first rock, (d) half as much as that of the first rock, (e) impossible to determine.
View SolutionA particle is released from height H. At certain height from the ground its kinetic energy is twice its gravitational potential energy. Find the height and speed of particle at that height.
View SolutionTwo balls are dropped to the ground from different heights. One ball is dropped 2s after the other, but both strike the ground at the same time 5s after the Ist is dropped.
(a) What is the difference in the heights from which they were dropped ?
(b) From what height was the first ball dropped?
View SolutionA body of mass 5 kg is thrown vertically up with a kinetic energy of 490 J. What will be height at which the kinetic energy of the body becomes half of the original value ? (Acceleration due to gravity=9.8ms−2)
View SolutionA body of mass 2kg is thrown up vertically with kinetic energy of 490J. If g=9.8m/s2, the height at which the kinetic energy of the body becomes half of the original value, is
View SolutionA body of mass 2kg is projected upward from the surface of the ground at t=0 with a velocity of 20m/s. One second later a body B, also of mass 2kg, is dropped from a height of 20m. If they collide elastically, then velocities just after collision are :-
View Solution
