A particle is moving in a circle with uniform speed. IT has constant
velocity
Acceleration
kinetic energy
displacement
The correct Answer is:c
To solve the question, we need to analyze the motion of a particle moving in a circle with uniform speed and determine which of the given options is constant.
1. Understanding the Motion:
- A particle is moving in a circular path with uniform speed. This means that while the speed (magnitude of velocity) remains constant, the direction of the particle's velocity is continuously changing.
2. Analyzing Velocity:
- Velocity is a vector quantity, which means it has both magnitude and direction. Since the direction of the particle's motion changes at every point along the circular path, the velocity is not constant.
- Conclusion: Velocity is not constant.
3. Analyzing Acceleration:
- Acceleration is defined as the rate of change of velocity. Since the velocity is changing (due to the change in direction), the acceleration is also changing.
- In circular motion, there is centripetal acceleration directed towards the center of the circle, and if there is any tangential acceleration (due to changes in speed), it would also contribute to the overall acceleration.
- Conclusion: Acceleration is not constant.
4. Analyzing Kinetic Energy:
- Kinetic energy (KE) is given by the formula KE=12mv2, where m is mass and v is speed.
- Since the speed of the particle is constant and the mass is also constant, the kinetic energy remains constant throughout the motion.
- Conclusion: Kinetic energy is constant.
5. Analyzing Displacement:
- Displacement is the shortest distance from the initial to the final position of the particle. As the particle moves around the circle, its displacement changes continuously.
- For example, when the particle returns to its starting point, the displacement is zero, but at other points, it has a non-zero value.
- Conclusion: Displacement is not constant.
Final Answer:
The only quantity that remains constant as the particle moves in a circle with uniform speed is kinetic energy.
- A particle is moving in a circular path with uniform speed. This means that while the speed (magnitude of velocity) remains constant, the direction of the particle's velocity is continuously changing.
- Velocity is a vector quantity, which means it has both magnitude and direction. Since the direction of the particle's motion changes at every point along the circular path, the velocity is not constant.
- Conclusion: Velocity is not constant.
- Acceleration is defined as the rate of change of velocity. Since the velocity is changing (due to the change in direction), the acceleration is also changing.
- In circular motion, there is centripetal acceleration directed towards the center of the circle, and if there is any tangential acceleration (due to changes in speed), it would also contribute to the overall acceleration.
- Conclusion: Acceleration is not constant.
- Kinetic energy (KE) is given by the formula KE=12mv2, where m is mass and v is speed.
- Since the speed of the particle is constant and the mass is also constant, the kinetic energy remains constant throughout the motion.
- Conclusion: Kinetic energy is constant.
- Displacement is the shortest distance from the initial to the final position of the particle. As the particle moves around the circle, its displacement changes continuously.
- For example, when the particle returns to its starting point, the displacement is zero, but at other points, it has a non-zero value.
- Conclusion: Displacement is not constant.
The only quantity that remains constant as the particle moves in a circle with uniform speed is kinetic energy.
Topper's Solved these Questions
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - UNIFORM CIRCULAR MOTION (ROTATIONAL MOTION AND MOMENT OF INERTIA )
25 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - GRAVITATION
25 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - MOTION IN FLUIDS
25 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
24 Videos
- DIMENSIONSBOOK - ICSECHAPTER - DIMENSIONSEXERCISE - SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)
9 Videos
Similar Questions
A particle is moving in a circle with uniform speed its motion is
View SolutionA particle is moving in a circle with uniform speed v . In moving from a point to another diametrically opposite point
View SolutionWhen a particle moves in a circle with a uniform speed
View SolutionA particle is rotating in circle with uniform speed as shown. The angular momentum of the particle w.r.t. origin is :-

View SolutionA particle moves in a circular path with a uniform speed. Its motion is
View Solution(A): When a particle moves in a circle with a uniform speed, its velocity and accele ration both changes.
(R): The centripetal acceleration in circular motion is dependent on angular velocity of the body.
View SolutionConsider a particle , moving in a circle with constant speed . P is a point outside the circle , in the same plane . Then , the angular momentum of the particle about the point P is
View SolutionA particle is moving along a circle of radius R with a uniform speed v. At t=0 , the particle is moving along the east. Find the average acceleration (magnitude and direction) in 1/4th revolution.
View SolutionA particle P is moving in a circle of radius 'a' with a uniform speed v. C is the centre of the circle and AB is a diameter. When passing through B the angular velocity of P about A and C are in the ratio
View SolutionA particle is moving in a circle of radius r with speed v as shown in the figure. The magnitude of change in velocity in moving from P to Q is
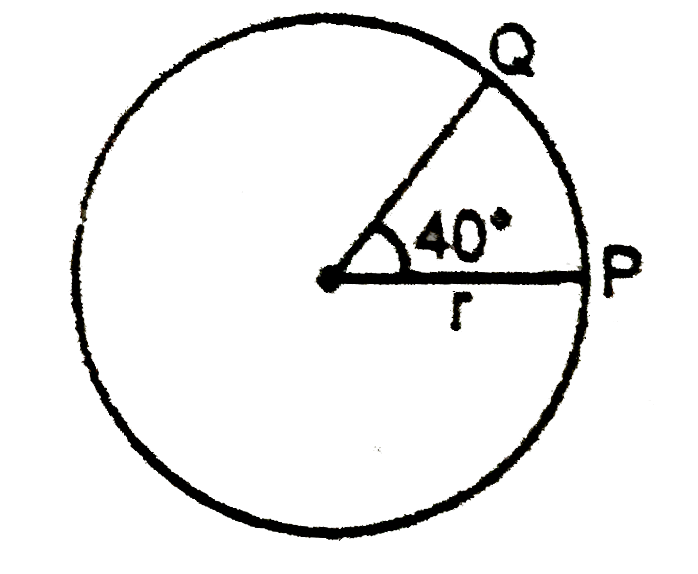
View Solution
