Two cells of same emf E, but different internal resistances r1andr2 are connected to an external
resistance R as shown in the figure given above. The voltmeter V reads zero. Obtain an expression for R in terms of r1andr2. Calculate the voltage across the cell of internal resistance r2. (Assume that the voltmeter V is of infinite resistance).
To solve the problem, we need to analyze the circuit with two cells of the same emf E but different internal resistances r1 and r2 connected to an external resistance R. The voltmeter V reads zero, which indicates that there is no potential difference across it.
1. Understanding the Circuit:
- We have two cells in series, each with an emf E and internal resistances r1 and r2.
- The total emf in the circuit is 2E (since both cells contribute).
- The total internal resistance is r1+r2.
2. Applying Kirchhoff's Loop Rule:
- According to Kirchhoff's loop rule, the sum of the potential differences around a closed loop is zero.
- The equation can be written as:
2E−I(R+r1+r2)=0
- Here, I is the current flowing through the circuit.
3. Finding the Current I:
- Rearranging the equation gives:
I=2ER+r1+r2
4. Voltage Across the Internal Resistance r2:
- The voltage drop across r2 can be calculated using Ohm's law:
Vr2=I⋅r2
- Substituting the expression for I:
Vr2=(2ER+r1+r2)⋅r2
5. Condition for Voltmeter Reading Zero:
- The voltmeter reads zero when the potential difference across it is zero, which means:
E−Ir1=0
- Thus, we can write:
E=Ir1
- Substituting I from the previous step:
E=(2ER+r1+r2)r1
6. Solving for R:
- Rearranging gives:
E(R+r1+r2)=2Er1
- Dividing both sides by E (assuming E≠0):
R+r1+r2=2r1
- Therefore:
R=2r1−r1−r2=r1−r2
Final Expressions:
- The expression for the external resistance R in terms of r1 and r2 is:
R=r1−r2
- The voltage across the cell with internal resistance r2 is:
Vr2=2Er2R+r1+r2
- We have two cells in series, each with an emf E and internal resistances r1 and r2.
- The total emf in the circuit is 2E (since both cells contribute).
- The total internal resistance is r1+r2.
- According to Kirchhoff's loop rule, the sum of the potential differences around a closed loop is zero.
- The equation can be written as:
2E−I(R+r1+r2)=0
- Here, I is the current flowing through the circuit.
- Rearranging the equation gives:
I=2ER+r1+r2
- The voltage drop across r2 can be calculated using Ohm's law:
Vr2=I⋅r2
- Substituting the expression for I:
Vr2=(2ER+r1+r2)⋅r2
- The voltmeter reads zero when the potential difference across it is zero, which means:
E−Ir1=0
- Thus, we can write:
E=Ir1
- Substituting I from the previous step:
E=(2ER+r1+r2)r1
- Rearranging gives:
E(R+r1+r2)=2Er1
- Dividing both sides by E (assuming E≠0):
R+r1+r2=2r1
- Therefore:
R=2r1−r1−r2=r1−r2
- The expression for the external resistance R in terms of r1 and r2 is:
R=r1−r2
- The voltage across the cell with internal resistance r2 is:
Vr2=2Er2R+r1+r2
Similar Questions
Two cells of the same e.mf. e but different internal resistances r1andr2 are connected in series with an external resistance 'R'. The potential drop across the first cell is found to be zero. The external resistance Ris
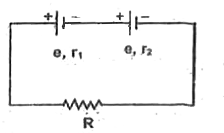
View SolutionA cell of emf E and internal resistance r is connected across an external resistance R. Plot a graph showing the variation o P.D. Across R, verses 'R.
View Solution
Knowledge Check
In a circuit a cell with internal resistance r is connected to an external resistance R. The condition for the maximum current that drawn from the cell is
AR=rBR<rCR>rDR=0
Two cells of equal e.m.f. E b ut of difference internal resistance r1 and r2 are connected in parallel and the combination is connected with an external resistance R to obtain maximum power in R. find the value of R

View Solution
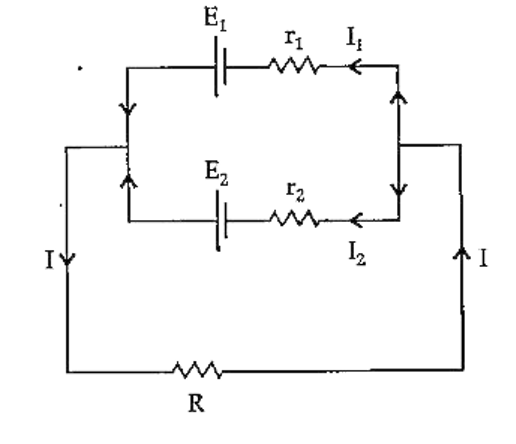
Two cells of same emf E but internal resistance r1andr2 are connected in series to an external resistor R(figure). What should be the value of R so that the potential difference across the terminals of the first cell becomes zero?
View SolutionTwo cells of emf E each and internal resistance r1 and r2 are connected in series across a load resistance R. If potential difference across the first cell is zero, then find the relation between R,r1 and r2
View SolutionTwo cells of emf E each and internal resistance r1 and r2 are connected in series across a load resistance R. If potential difference across the first cell is zero, then find the relation between R,r1 and r2
View SolutionTwo cells of emf E1 and E2 have internal resistance r1 and r2. Deduce an expression for equivalent emf of their parallel combination.
View SolutionA cell of emf E and internal resistance r is connected in series with an external resistance nr. Than what will be the ratio of the terminal potential difference to emf, if n=9.
View SolutionA cell of emf E having an internal resistance R varies with R as shown in figure by the curve

View Solution