A cell of emf E and internal resistance r is connected across an external resistance R. Plot a graph showing the variation o P.D. Across R, verses 'R.
To solve the problem of plotting the variation of potential difference (P.D.) across an external resistance R versus R when connected to a cell of EMF E and internal resistance r, we can follow these steps:
Step 1: Understand the Circuit
We have a cell with EMF E and internal resistance r connected to an external resistance R. The total resistance in the circuit is R+r.
Step 2: Write the Expression for Potential Difference
The potential difference V across the external resistance R can be expressed using Ohm's law and the formula for the total current I in the circuit:
I=ER+r
The potential difference across R is given by:
V=I⋅R=ER+r⋅R
This simplifies to:
V=E⋅RR+r
Step 3: Analyze the Equation
From the equation V=E⋅RR+r, we can see that:
- E is a constant (the EMF of the cell).
- r is also a constant (the internal resistance).
- R is the variable we will change.
Step 4: Determine the Behavior of V as R Changes
1. When R=0:
V=E⋅00+r=0
2. As R increases, V increases.
3. When R approaches infinity:
V = \frac{E \cdot R}{R + r} \approx E \quad \text{(as R becomes very large)}
Step 5: Plot the Graph
- On the x-axis, we will plot the external resistance R.
- On the y-axis, we will plot the potential difference V.
- The graph starts at the origin (0,0) and rises as R increases, approaching the value E asymptotically.
Step 6: Conclusion
The graph will show that V increases with R and levels off at E, indicating that the potential difference across the external resistance reaches a maximum equal to the EMF of the cell.
Graph Summary
- The graph starts at (0,0).
- It increases steadily and approaches a horizontal asymptote at V=E.
We have a cell with EMF E and internal resistance r connected to an external resistance R. The total resistance in the circuit is R+r.
The potential difference V across the external resistance R can be expressed using Ohm's law and the formula for the total current I in the circuit:
I=ER+r
The potential difference across R is given by:
V=I⋅R=ER+r⋅R
This simplifies to:
V=E⋅RR+r
From the equation V=E⋅RR+r, we can see that:
- E is a constant (the EMF of the cell).
- r is also a constant (the internal resistance).
- R is the variable we will change.
1. When R=0:
V=E⋅00+r=0
2. As R increases, V increases.
3. When R approaches infinity:
V = \frac{E \cdot R}{R + r} \approx E \quad \text{(as R becomes very large)}
- On the x-axis, we will plot the external resistance R.
- On the y-axis, we will plot the potential difference V.
- The graph starts at the origin (0,0) and rises as R increases, approaching the value E asymptotically.
The graph will show that V increases with R and levels off at E, indicating that the potential difference across the external resistance reaches a maximum equal to the EMF of the cell.
- The graph starts at (0,0).
- It increases steadily and approaches a horizontal asymptote at V=E.
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:NCERT EXEMPLAR ENGLISHChapter:CURRENT ELECTRICITYExercise:MCQs (More than one options)
Explore 5 Videos
- COMMUNICATION SYSTEMBook:NCERT EXEMPLAR ENGLISHChapter:COMMUNICATION SYSTEMExercise:LONG ANSWER TYPE QUESTIONS
Explore 5 Videos
- DUAL NATURE OF RADIATION AND MATTERBook:NCERT EXEMPLAR ENGLISHChapter:DUAL NATURE OF RADIATION AND MATTERExercise:LONG ANSWER TYPE QUESTION
Explore 5 Videos
Similar Questions
A cell of e.m.f. 'E' and internal resistance 'r' is connected across a variable resistor 'R'. Plot a graph showing the variation of terminal potential 'V' with resistance R.
Predict from the graph the condition under which 'V' becomes equal of 'E'

View SolutionA cell of emf ε and internal resistance r is connected across a variable resistor R. Plot a graph showing variation of terminal voltage V of the cell versus the current I. Using the plot, show how the emf of the cell and its internal resistance can be determined.
View Solution
Knowledge Check
A cell having an emf E and internal resistance r is connected across a variable external resistance R. As the resistance R is increased, the plot of potential difference V across R is given by
A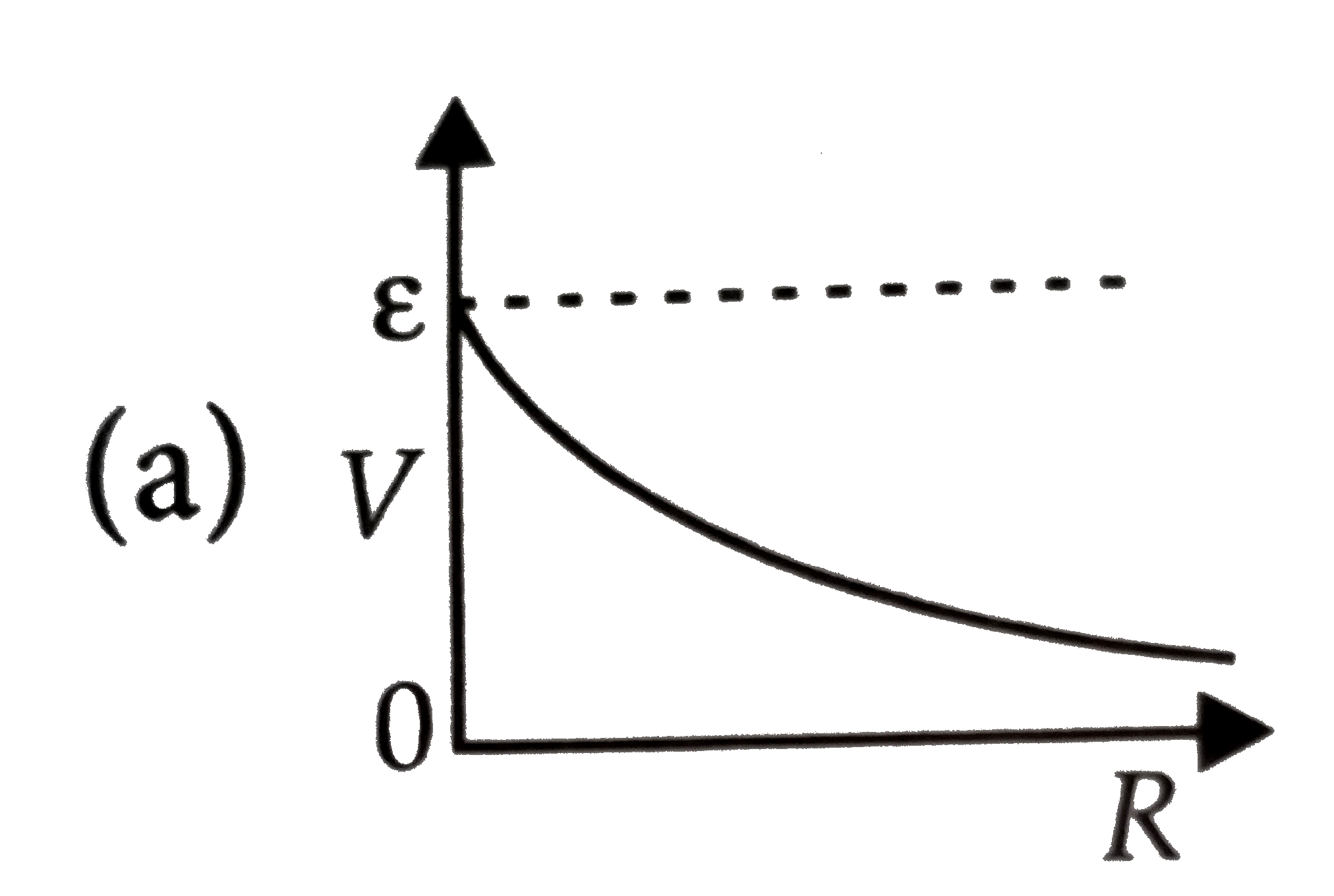 B
B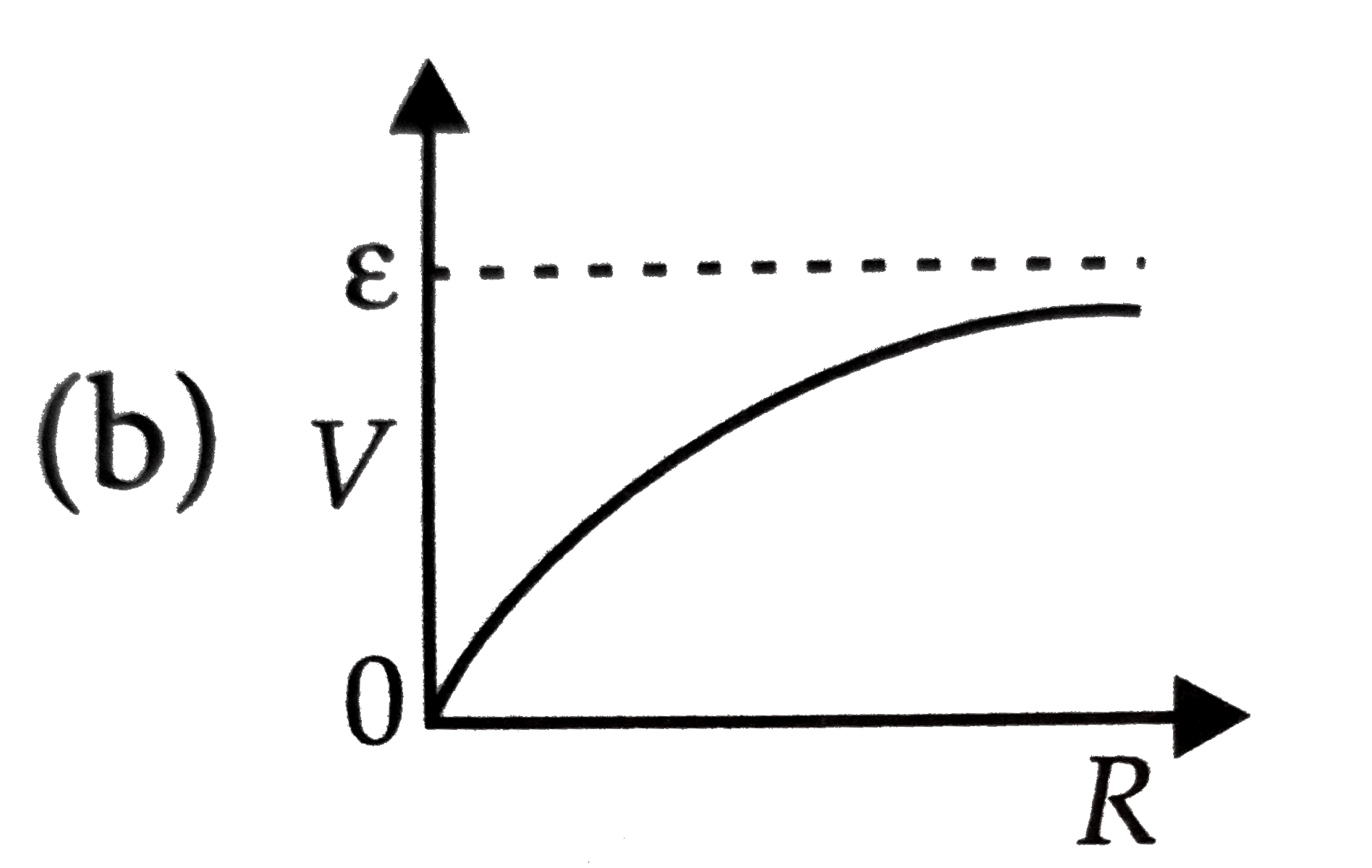 C
C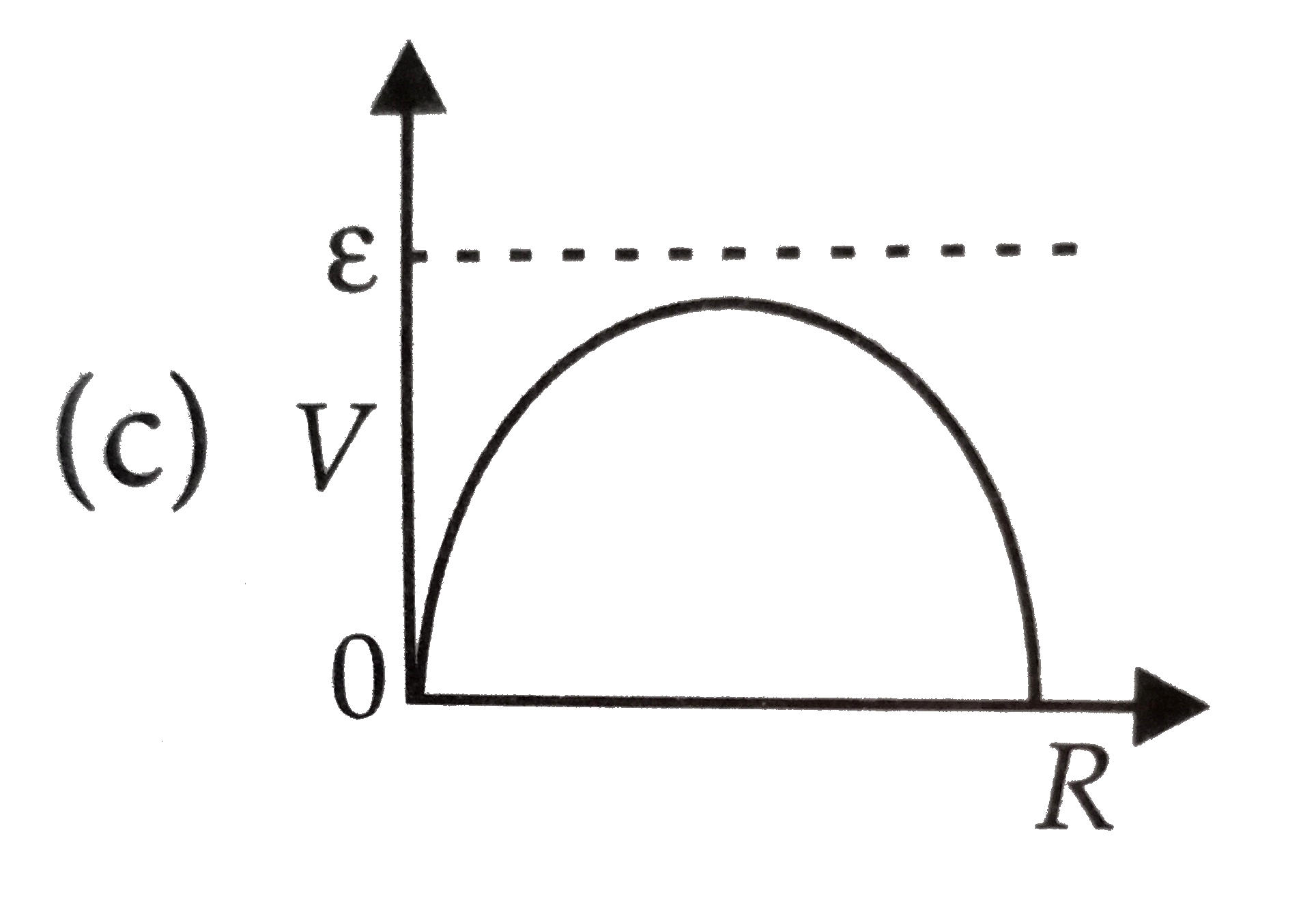 D
D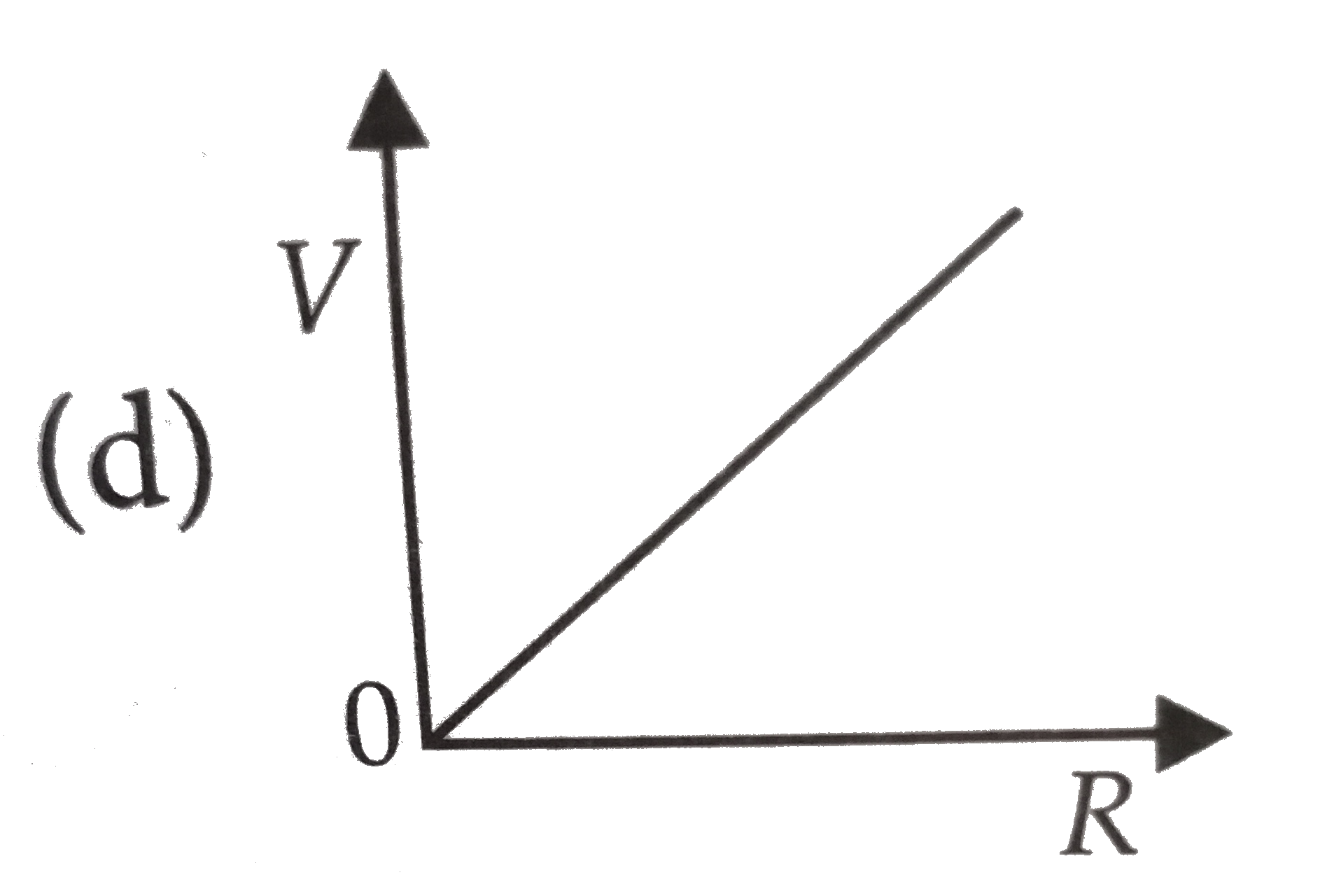
A cell of e.m.f E and internal resitance r ,is connected to an external resistance R. the variation of potential difference V across the resistance R as function of R as shown in the figure, is the best represented by curve
_PHY_E01_011_Q01.png)
View SolutionA cell of emf E and internal resistance r is connected in series with an external resistance nr. Than what will be the ratio of the terminal potential difference to emf, if n=9.
View SolutionA cell of emf ε and internal resistance r is connected across a load resistance R
(i) Find the maximum power delivered at the load ?
.
View SolutionA cell of e.m. E and internal resistance r is connected across a resistancer. The potential difference between the terminals of the cell must be
View SolutionFour cells, each of emf E and internal resistance r, are connected in series across an external resistance R. By mistake one of the cells is connected in reverse. Then, the current in the external circuit is
View SolutionA cell of e.mf. E and internal resistance r is connected in series with an external resistance nr. Then, the ratio of the terminal potential difference to E.M.F.is
View SolutionAn element with emfε and interval resistance r is connected across an external resistance R. The maximum power in external circuit is 9W. The current flowing through the circuit in these conditions is 3A. Then which of the following is // are correct ?
View Solution

