If the radius of a wheel is 14 cm, find the distance covered in 150 revolutions.
15,200cm
13,200cm
14,200cm
16,200cm
The correct Answer is:B
To find the distance covered by a wheel in 150 revolutions when the radius is given as 14 cm, we can follow these steps:
Step 1: Calculate the Circumference of the Wheel
The formula for the circumference C of a circle (or wheel) is given by:
C=2πr
where r is the radius of the wheel.
Given:
- Radius r=14 cm
Substituting the value of r:
C=2π×14
Step 2: Substitute the Value of π
We can use π≈227 for our calculations:
C=2×227×14
Step 3: Simplify the Calculation
Now, let's simplify the expression:
C=2×22×147
Calculating 22×14:
22×14=308
Now substituting back:
C=2×3087
C=6167 cm
Step 4: Calculate the Distance for 150 Revolutions
The distance covered in 150 revolutions is given by:
Distance=150×C
Substituting the value of C:
Distance=150×6167
Step 5: Perform the Multiplication
Calculating the distance:
Distance=150×6167
Calculating 150×616:
150×616=92400
So,
Distance=924007
Step 6: Divide to Find the Final Distance
Now, dividing:
Distance=13200 cm
Step 7: Convert to Meters
Since 100 cm = 1 m, we can convert the distance into meters:
Distance in meters=13200100=132 m
Final Answer
The distance covered in 150 revolutions is 13200 cm or 132 m.
---
The formula for the circumference C of a circle (or wheel) is given by:
C=2πr
where r is the radius of the wheel.
- Radius r=14 cm
C=2π×14
We can use π≈227 for our calculations:
C=2×227×14
Now, let's simplify the expression:
C=2×22×147
Calculating 22×14:
22×14=308
Now substituting back:
C=2×3087
C=6167 cm
The distance covered in 150 revolutions is given by:
Distance=150×C
Substituting the value of C:
Distance=150×6167
Calculating the distance:
Distance=150×6167
Calculating 150×616:
150×616=92400
So,
Distance=924007
Now, dividing:
Distance=13200 cm
Since 100 cm = 1 m, we can convert the distance into meters:
Distance in meters=13200100=132 m
The distance covered in 150 revolutions is 13200 cm or 132 m.
Topper's Solved these Questions
- PERIMETER AND AREA BOOK - ICSECHAPTER - PERIMETER AND AREA EXERCISE - WARMUP EXERCISE
10 Videos
- PERIMETER AND AREA BOOK - ICSECHAPTER - PERIMETER AND AREA EXERCISE - EXERCISE 17.1
15 Videos
- PERCENTAGE, PROFIT AND LOSSBOOK - ICSECHAPTER - PERCENTAGE, PROFIT AND LOSSEXERCISE - REVISION EXERCISE
26 Videos
- PROBABILITYBOOK - ICSECHAPTER - PROBABILITYEXERCISE - TRY THIS
14 Videos
Similar Questions
The diameter of the wheel of a car is 0.70 m. Find the distance covered by it in 500 revolutions.
If the wheel takes 5 minutes to make 500 revolutions, find its speed in :
(i) m/s (ii) km/hr
View SolutionThe radius of a circular wheel is 42 cm. Find the distance travelled by it in:
(i) 1 revolution
(ii) 50 revolutions
(iii) 200 revolutions
View SolutionThe radius of a wheel is 77 cm, find the number of revolutions it will make in travelling a distance of 2.904 km
View SolutionThe diameter of a wheel of a car is 1.12 m . Find the distance covered by the car in making 5000 revolutions by its wheels . (Take π=227)
View SolutionThe diameter of a circle is 14 cm, find its radius.
View SolutionThe diameter of a wheel of a car is 63 cm. Find the distance travelled by the car during the period, the wheel makes 1000 revolutions.
View SolutionA round table cover has six equal designs as shown in the given figure. If the radius of the cover is 35 cm then find the total area of the design. (Use √3=1.732andπ=3.14)
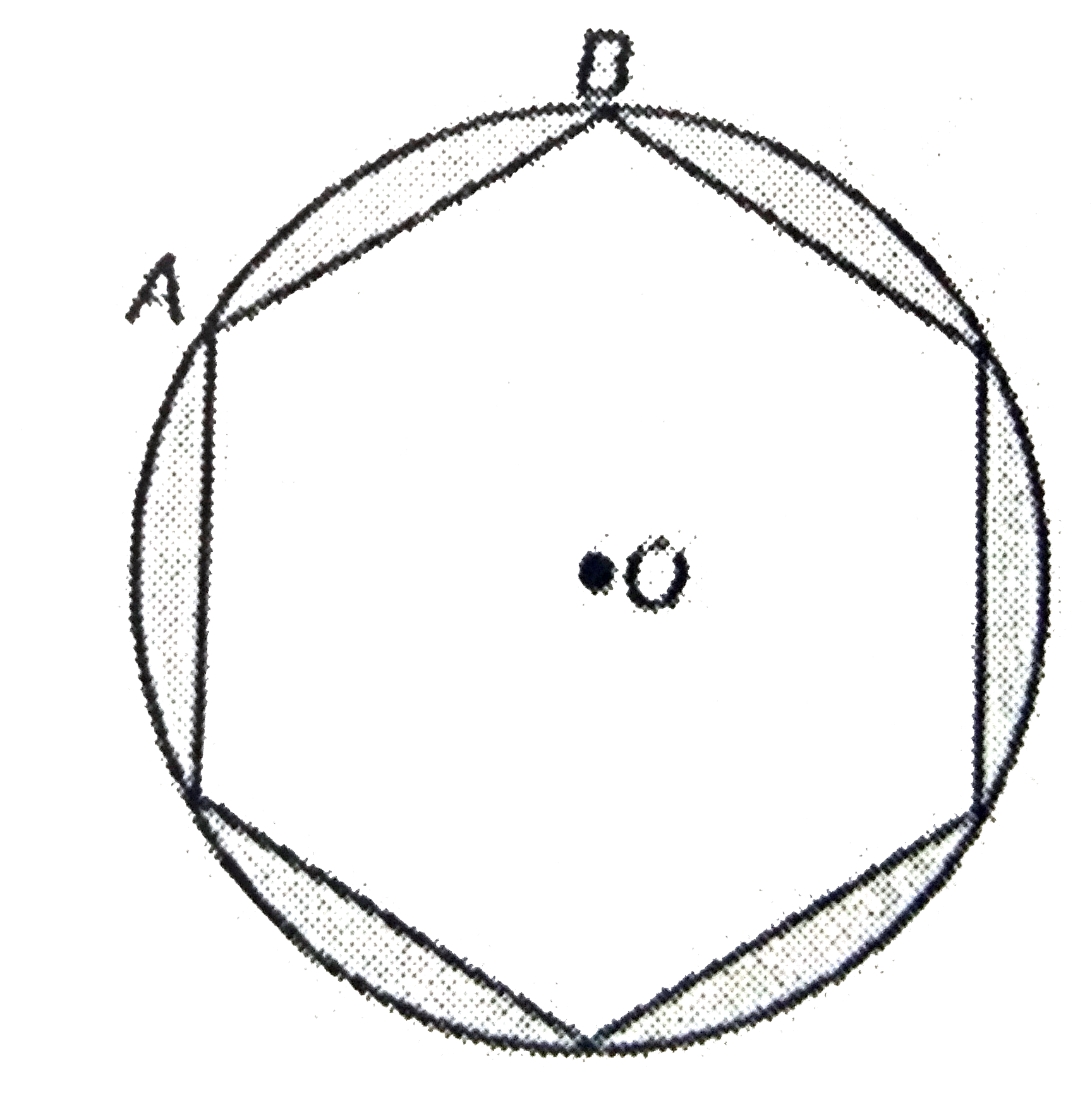
View SolutionThe radius of a circle is 14 cm. Find the radius of the circle whose area is double of the area of the circle.
View SolutionThe diameter of a garden roller is 1.4 m and it is 2m long . Find the maximum area covered by it in 50 revolutions?
View SolutionAn express train is running between two stations with a uniform speed. If the diameter of each wheel of the train is 42 cm and each wheel makes 1200 revolutions per minutes, find the speed of the train.
View Solution
