A rectangle measures 27 cm by 16 cm. One diagonal divides the rectangle into two triangles. Find the area of each triangle.
The correct Answer is:216 sq. cm
To find the area of each triangle formed by the diagonal of the rectangle, we can follow these steps:
Step 1: Identify the dimensions of the rectangle
The rectangle has a length of 27 cm and a breadth of 16 cm.
Step 2: Understand how the diagonal divides the rectangle
The diagonal of the rectangle divides it into two right-angled triangles. Let's label the rectangle as ABCD, where AB = 27 cm (length) and AD = 16 cm (breadth). The diagonal AC divides the rectangle into triangles ABC and ADC.
Step 3: Use the formula for the area of a triangle
The area A of a triangle can be calculated using the formula:
A=12×base×height
In our case, for triangle ABC:
- The base can be taken as AB (27 cm)
- The height can be taken as AD (16 cm)
Step 4: Substitute the values into the formula
Substituting the values into the area formula:
A=12×27×16
Step 5: Calculate the area
First, calculate 27×16:
27×16=432
Now, substitute this back into the area formula:
A=12×432=216 cm2
Step 6: Conclusion
Since both triangles (ABC and ADC) are identical, the area of triangle ADC is also 216 cm². Thus, the area of each triangle is:
Area of each triangle=216 cm2
---
The rectangle has a length of 27 cm and a breadth of 16 cm.
The diagonal of the rectangle divides it into two right-angled triangles. Let's label the rectangle as ABCD, where AB = 27 cm (length) and AD = 16 cm (breadth). The diagonal AC divides the rectangle into triangles ABC and ADC.
The area A of a triangle can be calculated using the formula:
A=12×base×height
In our case, for triangle ABC:
- The base can be taken as AB (27 cm)
- The height can be taken as AD (16 cm)
Substituting the values into the area formula:
A=12×27×16
First, calculate 27×16:
27×16=432
Now, substitute this back into the area formula:
A=12×432=216 cm2
Since both triangles (ABC and ADC) are identical, the area of triangle ADC is also 216 cm². Thus, the area of each triangle is:
Area of each triangle=216 cm2
Topper's Solved these Questions
Similar Questions
A rectangle measure 30 cm by 25 cm. A diagonal divides the rectangle into two triangles. Find the area of each triangle.
View SolutionEach side of an equilateral triangle measure 10 cm. Find the area of the triangle .
View SolutionThe length of a rectangle exceeds its width by 8 cm and the area of the rectangle is 240 sq. cm. Find the dimensions of the rectangle.
View SolutionPLOT is a rectangel with length 22 cm and breadth 16 cm. PO is a diagonal of this rectangel. Find the ara of the ΔPLO.
View SolutionThe perimeter of a rectangle is 130 cm. If the breadth of the rectangle is30 cm, find its length. Also find the area of the rectangle.
View SolutionTwo sides of a triangle are 6 cm and 8 cm. If height of the triangle corresponding to 6 cm side is 4 cm, find :
(i) area of the triangle
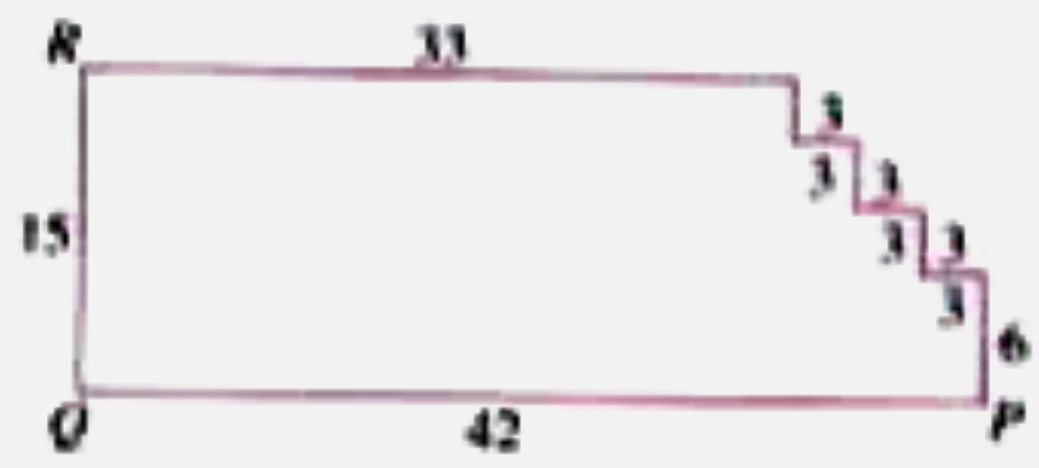
(ii) height of the triangle corresponding to 8 cm side.View SolutionPQRS is a rectangle measuring 42 cm by 15 cm. Find its perimeter and area. A portion from one end of the rectangle is cut off as shown in the perimeter and area of the new gigure thus formed.
 View Solution
View SolutionA square has the same area as that of a rectangle. The length and the breadth of the rectangle are 9cm and 4cm, respectively.
Find the area of the rectangle.View SolutionOne of the equal sides of an isosceles triangle is 13 cm and its perimeter is 50 cm. Find the area of the triangle.
View SolutionA rectangle has length 40 cm and breadth 30 cm, it has an ecternal isosceles triangle with equal sides of 17 cm each along one of its smaller sides as the base of te triangle. Find the pentagon thus formed.
View Solution
