In an isosceles triangle ABC, right angle at B. I is incentre of triangle then, find the ratio area of ΔAIB,ΔBICandΔAIC.
The correct Answer is:1:1:√2
To find the ratio of the areas of triangles AIB, BIC, and AIC in the isosceles triangle ABC with a right angle at B, we can follow these steps:
1. Identify the Triangle Dimensions:
- Let the lengths of the equal sides AB and BC be denoted as a.
- Since triangle ABC is a right triangle at B, the length of the hypotenuse AC can be calculated using the Pythagorean theorem:
AC=√AB2+BC2=√a2+a2=√2a2=a√2.
2. Determine the Inradius (r):
- The area A of triangle ABC can be calculated as:
A=12×AB×BC=12×a×a=a22.
- The semi-perimeter s of triangle ABC is:
s=AB+BC+AC2=a+a+a√22=2a+a√22=a(1+√22).
- The inradius r can be calculated using the formula r=As:
r=a22a(1+√22)=a2(1+√22).
3. Calculate Areas of Triangles AIB, BIC, and AIC:
- Area of triangle AIB:
AreaAIB=12×AB×r=12×a×r=12×a×a2(1+√22)=a24(1+√22).
- Area of triangle BIC:
AreaBIC=12×BC×r=12×a×r=a24(1+√22).
- Area of triangle AIC:
AreaAIC=12×AC×r=12×a√2×r=12×a√2×a2(1+√22)=a2√24(1+√22).
4. Find the Ratio of the Areas:
- Now we can express the ratio of the areas:
AreaAIB:AreaBIC:AreaAIC=a24(1+√22):a24(1+√22):a2√24(1+√22).
- Simplifying this gives:
1:1:√2.
Final Answer:
The ratio of the areas of triangles AIB, BIC, and AIC is 1:1:√2.
- Let the lengths of the equal sides AB and BC be denoted as a.
- Since triangle ABC is a right triangle at B, the length of the hypotenuse AC can be calculated using the Pythagorean theorem:
AC=√AB2+BC2=√a2+a2=√2a2=a√2.
- The area A of triangle ABC can be calculated as:
A=12×AB×BC=12×a×a=a22.
- The semi-perimeter s of triangle ABC is:
s=AB+BC+AC2=a+a+a√22=2a+a√22=a(1+√22).
- The inradius r can be calculated using the formula r=As:
r=a22a(1+√22)=a2(1+√22).
- Area of triangle AIB:
AreaAIB=12×AB×r=12×a×r=12×a×a2(1+√22)=a24(1+√22).
- Area of triangle BIC:
AreaBIC=12×BC×r=12×a×r=a24(1+√22).
- Area of triangle AIC:
AreaAIC=12×AC×r=12×a√2×r=12×a√2×a2(1+√22)=a2√24(1+√22).
- Now we can express the ratio of the areas:
AreaAIB:AreaBIC:AreaAIC=a24(1+√22):a24(1+√22):a2√24(1+√22).
- Simplifying this gives:
1:1:√2.
The ratio of the areas of triangles AIB, BIC, and AIC is 1:1:√2.
Topper's Solved these Questions
Similar Questions
Knowledge Check
In an isosceles triangle ABC, AB=AC and ∠A=3∠B. Find ∠C.
A36∘B32∘C28∘D40∘In an isosceles triangle DEF,∠D=110∘. If I is the in centre of the triangle, then what is the value (in degrees) of ∠EIF?
A110B130C145D155
Find the ratio of area of ΔADE to Δ ABC

View SolutionIn figure ABC is an isosceles triangle , right angled at C . Therefore

View SolutionIn △ABC, ∠A=58∘ . If I is the incentre of the triangle, then the measure of ∠BIC is:
View SolutionABC is an isosceles right Angle triangle . I is incentre. Find ratio of area of triangle ΔAICandΔABC
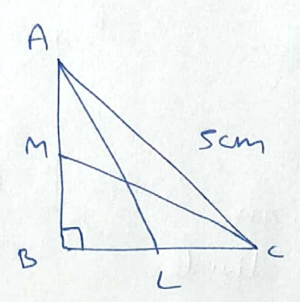
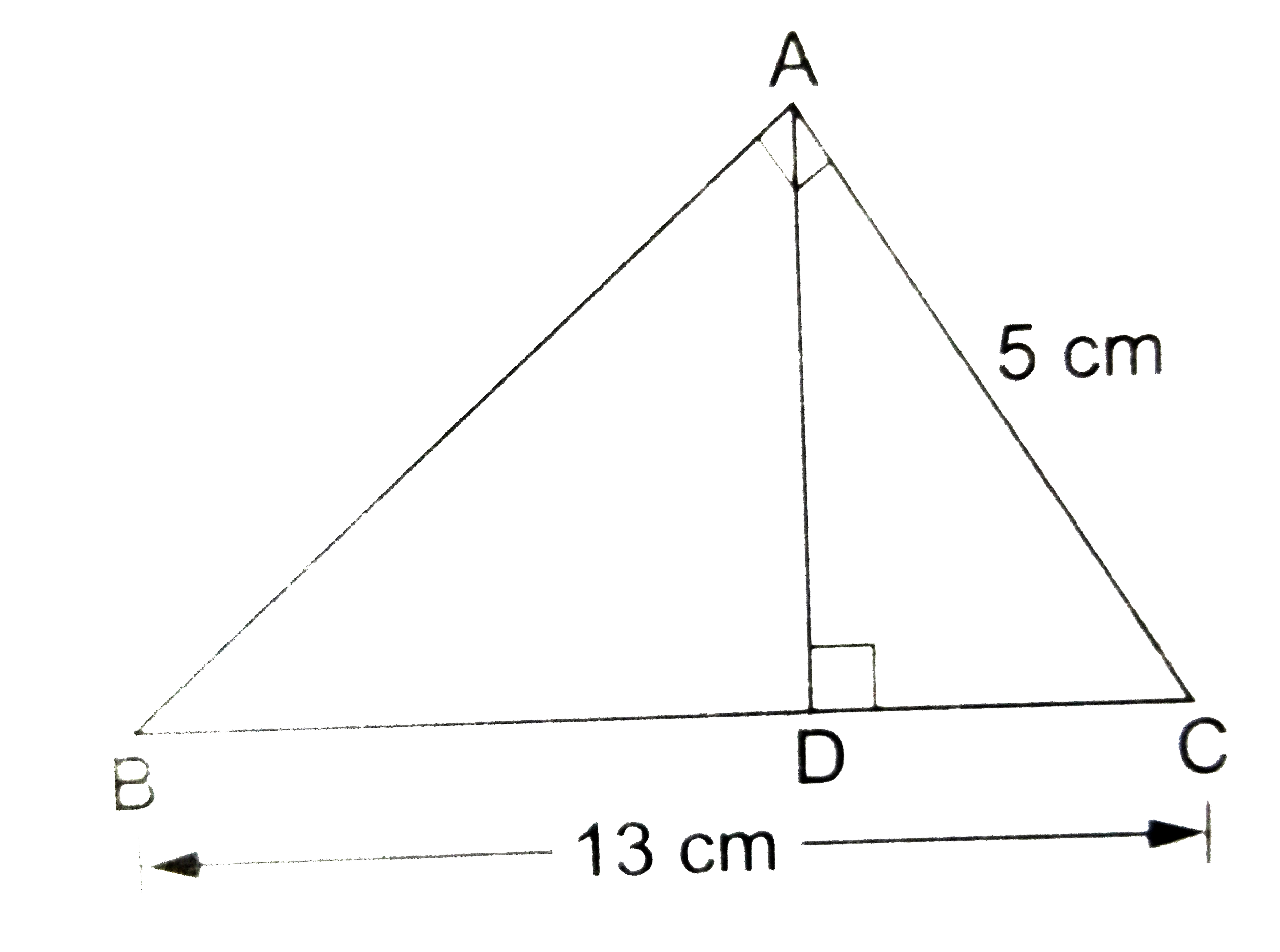
View SolutionΔABC is right-angled at A and AD⊥BC. If BC=13cmandAC=5cm, find the ratio of areas of ΔABCandΔADC.
View SolutionIn an isosceles right triangle PQR, if ∠Q=90∘, then find ∠PRQ.
View Solution
