Question

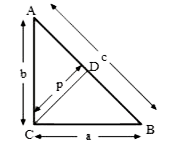
In a $\Delta ABC$ right angle at C .P is the length of $\perp$ from C to AB. If a, b, c, are the length of BC , CA, AB. Which relation is correct .

Options

A. `(1)/(P^(2))=(1)/(a^(2))-(1)/(b^(2))`
B. `(1)/(P^(2))=(1)/(b^(2))-(1)/(a^(2))`
C. `(1)/(P^(2))=(1)/(a^(2))+(1)/(b^(2))`
D. `(1)/(P^(2))+(1)/(a^(2))=(1)/(b^(2))=0`

Answer

(iii)

Explanation

To solve the problem step by step, we will analyze the right triangle ABC, where angle C is the right angle. We will derive the relationship between the sides of the triangle and the height from point C to side AB.

Step 1: Understand the Triangle
We have a right triangle ABC with:
- Angle C = 90 degrees
- Sides:
- BC = a
- CA = b
- AB = c

Step 2: Area of Triangle
The area of triangle ABC can be calculated using the formula:
\( \text{Area} = \frac{1}{2} \times \text{base} \times \text{height} \)
We can consider different bases and corresponding heights.

Step 3: Using Base AB
If we take AB as the base, the height from C to AB is P. Thus, the area can be expressed as:
\( \text{Area} = \frac{1}{2} \times c \times P \)

Step 4: Using Base BC
Now, if we take BC as the base, the height from A to BC is the same height P. Thus, the area can also be expressed as:
\( \text{Area} = \frac{1}{2} \times a \times P \)

Step 5: Equating the Areas
Since both expressions represent the area of the same triangle, we can equate them:
\( \frac{1}{2} \times c \times P = \frac{1}{2} \times a \times P \)
This simplifies to:
\( c \times P = a \times P \)
Assuming P is not zero, we can divide both sides by P:
\( c = a \)
This is not correct since we know that c is the hypotenuse.

Step 6: Using Pythagorean Theorem
Using the Pythagorean theorem, we know:
\( a^2 + b^2 = c^2 \)

Step 7: Expressing Area in Terms of Sides
We can also express the area in terms of the sides:
\( \text{Area} = \frac{1}{2} \times a \times b \)

Step 8: Relating Area with Height
We can also express the area using the height:
\( \text{Area} = \frac{1}{2} \times c \times P \)
Equating the two area expressions gives:
\( \frac{1}{2} \times a \times b = \frac{1}{2} \times c \times P \)
Cancelling \(\frac{1}{2}\) from both sides, we get:
\( a \times b = c \times P \)

Step 9: Deriving the Final Relation
Rearranging gives us:
\( P = \frac{a \times b}{c} \)
Now, we can use this to derive the relationship:
Using the area again, we can express:
\( \frac{1}{P} = \frac{1}{a} + \frac{1}{b} \)
This leads us to:
\( \frac{1}{c^2} = \frac{1}{a^2} + \frac{1}{b^2} \)

Final Relation
Thus, the correct relationship is:
\( \frac{1}{c^2} = \frac{1}{a^2} + \frac{1}{b^2} \)