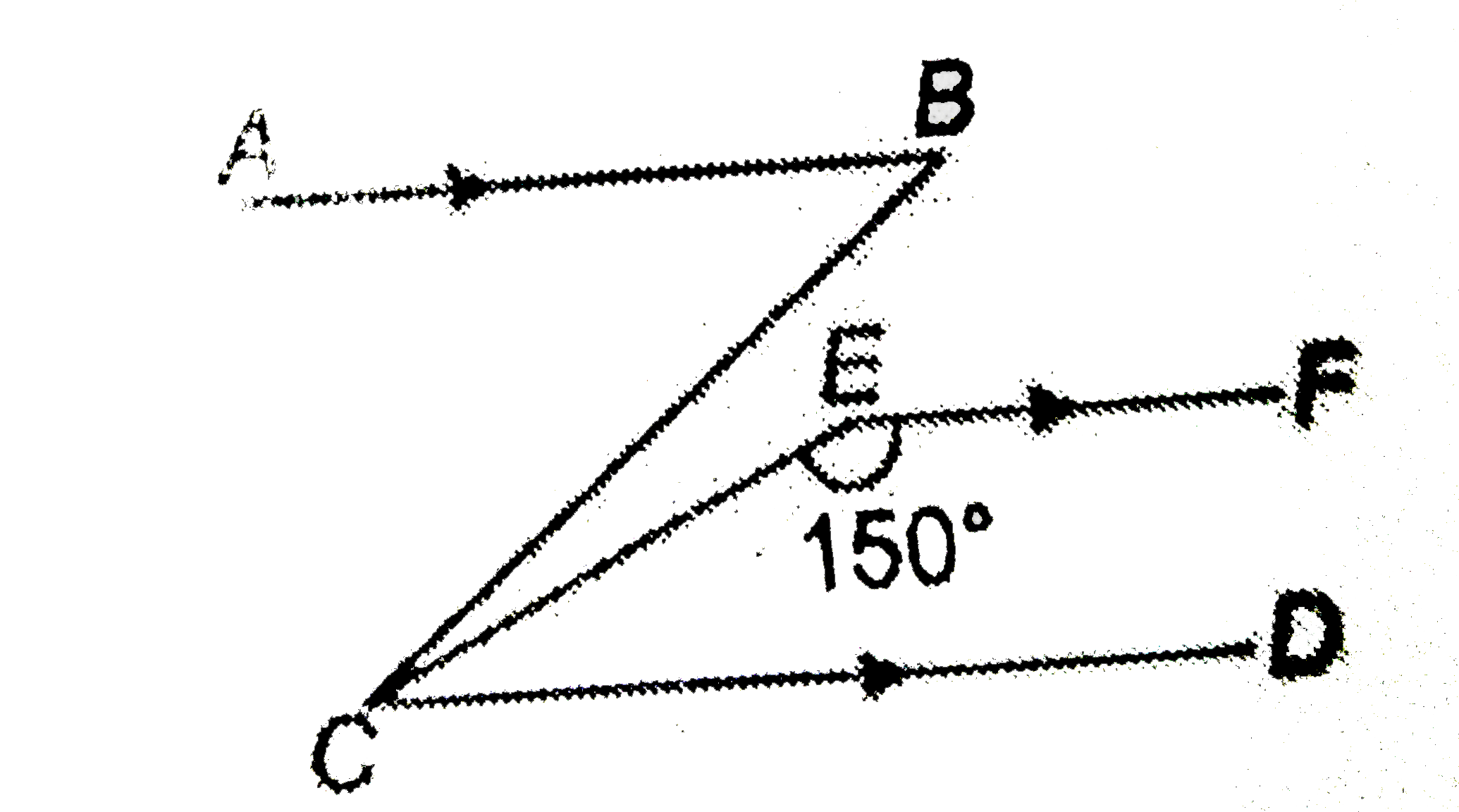
AB and CD are two parallel lines. PQ cuts AB and CD at E and F respectively. EL is the bisector of ∠BEF. If ∠LEB=35∘ ,then ∠CFQ will be :
70∘
55∘
110∘
125∘
The correct Answer is:C
To solve the problem, we need to analyze the given information step by step.
Step 1: Understand the Geometry
We have two parallel lines AB and CD, and a transversal line PQ that intersects AB at point E and CD at point F. The angle LEB is given as 35 degrees, and EL is the angle bisector of angle BEF.
Step 2: Identify Angles
Since EL is the bisector of angle BEF, we can denote:
- Angle LEB = 35 degrees (given)
- Angle LEF = 35 degrees (because EL bisects angle BEF)
Thus, angle BEF can be calculated as:
Angle BEF=Angle LEB+Angle LEF=35∘+35∘=70∘
Step 3: Use the Properties of Parallel Lines
Since AB and CD are parallel lines, the angles formed by the transversal PQ have certain relationships. Specifically, the angles on the same side of the transversal (interior angles) sum up to 180 degrees.
Let’s denote:
- Angle EFD = x (the angle we need to find)
According to the property of parallel lines:
Angle BEF+Angle EFD=180∘
Substituting the value of angle BEF:
70∘+x=180∘
Now, solve for x:
x=180∘−70∘=110∘
Step 4: Find Angle CFQ
By the alternate interior angles theorem, angle CFQ is equal to angle EFD because they are corresponding angles formed by the transversal PQ with the parallel lines AB and CD.
Thus:
Angle CFQ=Angle EFD=110∘
Final Answer
Therefore, the measure of angle CFQ is:
Angle CFQ=110∘
---
We have two parallel lines AB and CD, and a transversal line PQ that intersects AB at point E and CD at point F. The angle LEB is given as 35 degrees, and EL is the angle bisector of angle BEF.
Since EL is the bisector of angle BEF, we can denote:
- Angle LEB = 35 degrees (given)
- Angle LEF = 35 degrees (because EL bisects angle BEF)
Angle BEF=Angle LEB+Angle LEF=35∘+35∘=70∘
Since AB and CD are parallel lines, the angles formed by the transversal PQ have certain relationships. Specifically, the angles on the same side of the transversal (interior angles) sum up to 180 degrees.
- Angle EFD = x (the angle we need to find)
Angle BEF+Angle EFD=180∘
Substituting the value of angle BEF:
70∘+x=180∘
Now, solve for x:
x=180∘−70∘=110∘
By the alternate interior angles theorem, angle CFQ is equal to angle EFD because they are corresponding angles formed by the transversal PQ with the parallel lines AB and CD.
Angle CFQ=Angle EFD=110∘
Therefore, the measure of angle CFQ is:
Angle CFQ=110∘
Topper's Solved these Questions
Similar Questions
Knowledge Check
AB and CD are two parallel lines, PQ cuts AB and CD at E and F respectively. EL is the bisector of ∠BEF. If ∠LEB=35∘ then ∠CFQ will be :
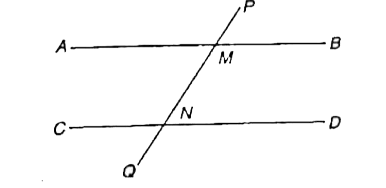
A70∘B55∘C110∘D125∘AB and CD are two parallel lines and a transversal PO intersects AB and CD at M and N respectively. The bisector of the interior angles form a quadrilateral :
 ArectangleBsquareCparallelogramDnone of these
ArectangleBsquareCparallelogramDnone of theseTwo parallel lines AB and CD are intersected by a transversal EF at M and N respectively.
The lines MP and NP are the bisectors of interior angles ∠BMN and ∠DNM on the same side of the transversal. Then ∠MPN is equal to :A60∘B90∘C45∘D120∘
In the given figure, AB∣∣CD and a transversal t cuts them at E and F respectively. If EP and FQ are the bisectors of ∠AEF and ∠EFD respectively, prove that EP∣∣FQ.

View SolutionIn the given figure, AB∣∣CD and a transversal t cuts them at E and F respectively. If EG and FG are this bisectors of ∠BEF and ∠EFD respectivelym prove that ∠EGF=90∘.

View SolutionAB and CD are parallel straight lines. EG intersects the lines AB and CD at F and G respectively. If ∠AFE=60∘ and the straight line HI bisects ∠EGD, then ∠DGI is
View SolutionAB and CD are two parallel lines. The points B and C are joined such that <ABC=65∘. A line CE is drawn making angle of 35∘ with the line CB, EF is drawn parallel to AB, as shown in figure, then <CEF is
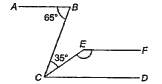
View SolutionTwo parallel lines AB and CD are intersected by a transversal EF at M and N respectively.
The lines MP and NP are the bisectors of interior angles ∠BMN and ∠DNM on the same side of the transversal. Then ∠MPN is equal to :
View Solution