A concave mirror is placed at the bottom of an empty tank with face upwards and axis vertical. When sunlight falls normally on the mirror, it is focussed at distance of 32cm from the mirror. If the tank filled with water (μ=4/3) up to a height of 20cm, then the sunlight will now get focussed at
The correct Answer is:B
To solve the problem step by step, let's break it down:
Step 1: Understand the Initial Setup
We have a concave mirror placed at the bottom of an empty tank. When sunlight falls normally on the mirror, it focuses at a distance of 32 cm from the mirror.
Step 2: Determine the Focal Length of the Mirror
Since the sunlight focuses at a distance of 32 cm when the tank is empty, this distance is equal to the focal length (f) of the concave mirror. Therefore:
- Focal length, f=32cm
Step 3: Fill the Tank with Water
The tank is filled with water up to a height of 20 cm. We need to find the new position where the sunlight will focus after the water is added.
Step 4: Calculate the Actual Depth Below Water Surface
The distance from the water surface to the mirror is:
- Actual depth, h=20cm
Step 5: Calculate the Distance from the Water Surface to the Focus Point
The distance from the mirror to the point where the sunlight was originally focused (O) is 32 cm. Therefore, the distance from the water surface to the focus point (O) is:
- Distance from water surface to mirror, BO=32cm−20cm=12cm
Step 6: Apply the Concept of Apparent Depth
When light passes from a denser medium (water) to a rarer medium (air), it bends away from the normal. The relationship between the actual depth and the apparent depth can be described using the refractive index (μ):
μwaterμair=Actual DepthApparent Depth
Given that:
- μwater=43
- μair=1
Step 7: Set Up the Equation
Let BI be the apparent depth (the new focus point). The actual depth BO is 12 cm. Thus, we can write:
431=12cmBI
Step 8: Solve for Apparent Depth
Rearranging the equation gives:
BI=12cm×143=12cm×34=9cm
Conclusion
When the tank is filled with water, the sunlight will now focus at a distance of 9 cm from the mirror.
---
We have a concave mirror placed at the bottom of an empty tank. When sunlight falls normally on the mirror, it focuses at a distance of 32 cm from the mirror.
Since the sunlight focuses at a distance of 32 cm when the tank is empty, this distance is equal to the focal length (f) of the concave mirror. Therefore:
- Focal length, f=32cm
The tank is filled with water up to a height of 20 cm. We need to find the new position where the sunlight will focus after the water is added.
The distance from the water surface to the mirror is:
- Actual depth, h=20cm
The distance from the mirror to the point where the sunlight was originally focused (O) is 32 cm. Therefore, the distance from the water surface to the focus point (O) is:
- Distance from water surface to mirror, BO=32cm−20cm=12cm
When light passes from a denser medium (water) to a rarer medium (air), it bends away from the normal. The relationship between the actual depth and the apparent depth can be described using the refractive index (μ):
μwaterμair=Actual DepthApparent Depth
Given that:
- μwater=43
- μair=1
Let BI be the apparent depth (the new focus point). The actual depth BO is 12 cm. Thus, we can write:
431=12cmBI
Rearranging the equation gives:
BI=12cm×143=12cm×34=9cm
When the tank is filled with water, the sunlight will now focus at a distance of 9 cm from the mirror.
Topper's Solved these Questions
- RAY OPTICSBook:DC PANDEY ENGLISHChapter:RAY OPTICSExercise:medical entrance special format question
Explore 23 Videos
- RAY OPTICSBook:DC PANDEY ENGLISHChapter:RAY OPTICSExercise:Medical entrance gallary
Explore 76 Videos
- RAY OPTICSBook:DC PANDEY ENGLISHChapter:RAY OPTICSExercise:Checkpoint 9.7
Explore 10 Videos
- NUCLEIBook:DC PANDEY ENGLISHChapter:NUCLEIExercise:C MADICAL ENTRANCES GALLERY
Explore 46 Videos
- REFLECTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFLECTION OF LIGHTExercise:Subjective
Explore 9 Videos
Similar Questions
A concave mirror of radius of curvature 60 cm is placed at the bottom of tank containing water upto a height of 20 cm. The mirror faces upwards with its axis vertical. Solar light falls normally on the surface of water and the image of the sun is formed. If aμw=43 then with the observer in air, the distance of the image from the surface of water is:
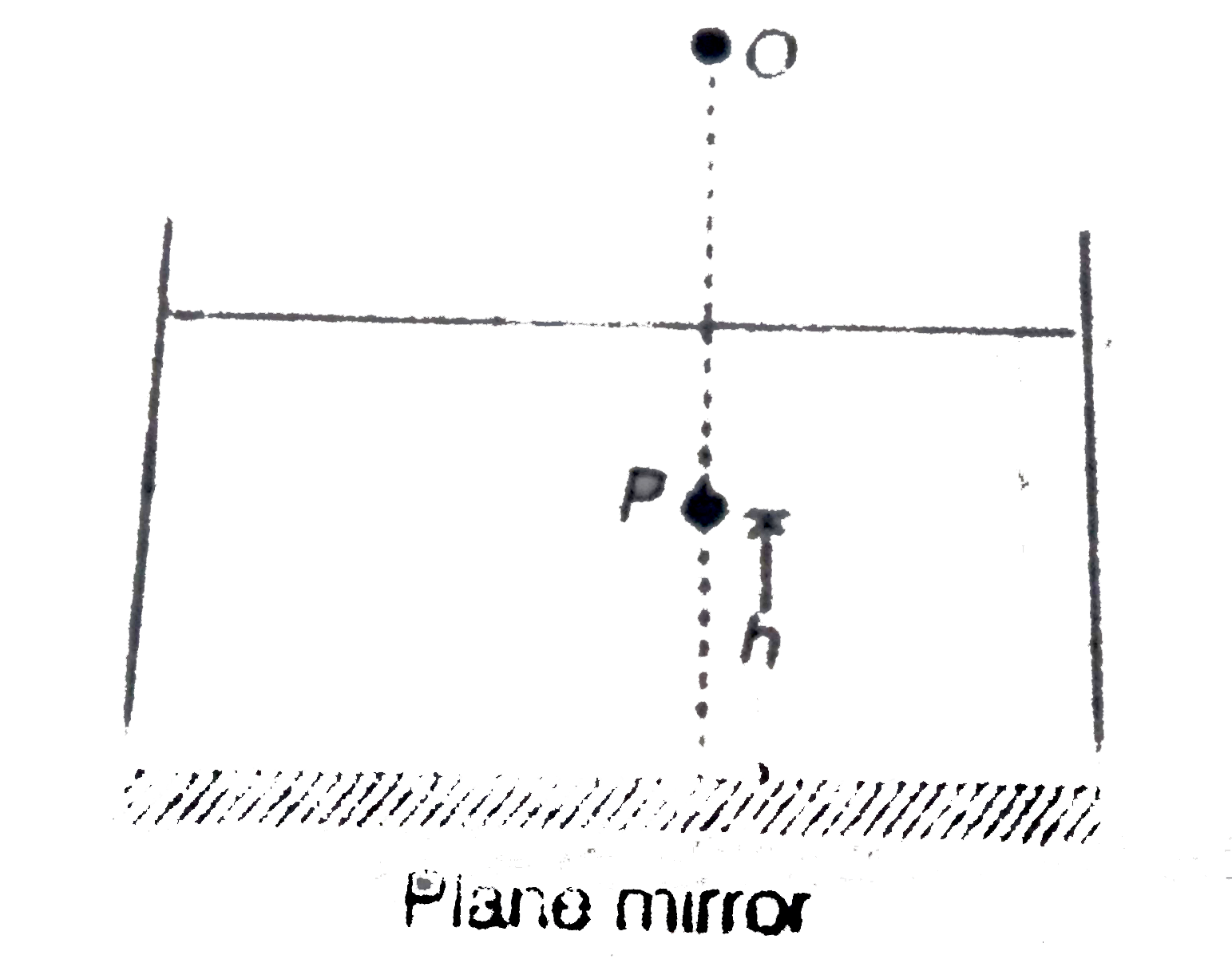
View SolutionA plane mirror is placed at the bottom of a tank containing a liquid of refractive index μ. P is a small object at a height h above the mirror. An observer observes P and its image in the mirror. The apparent distance between two will be
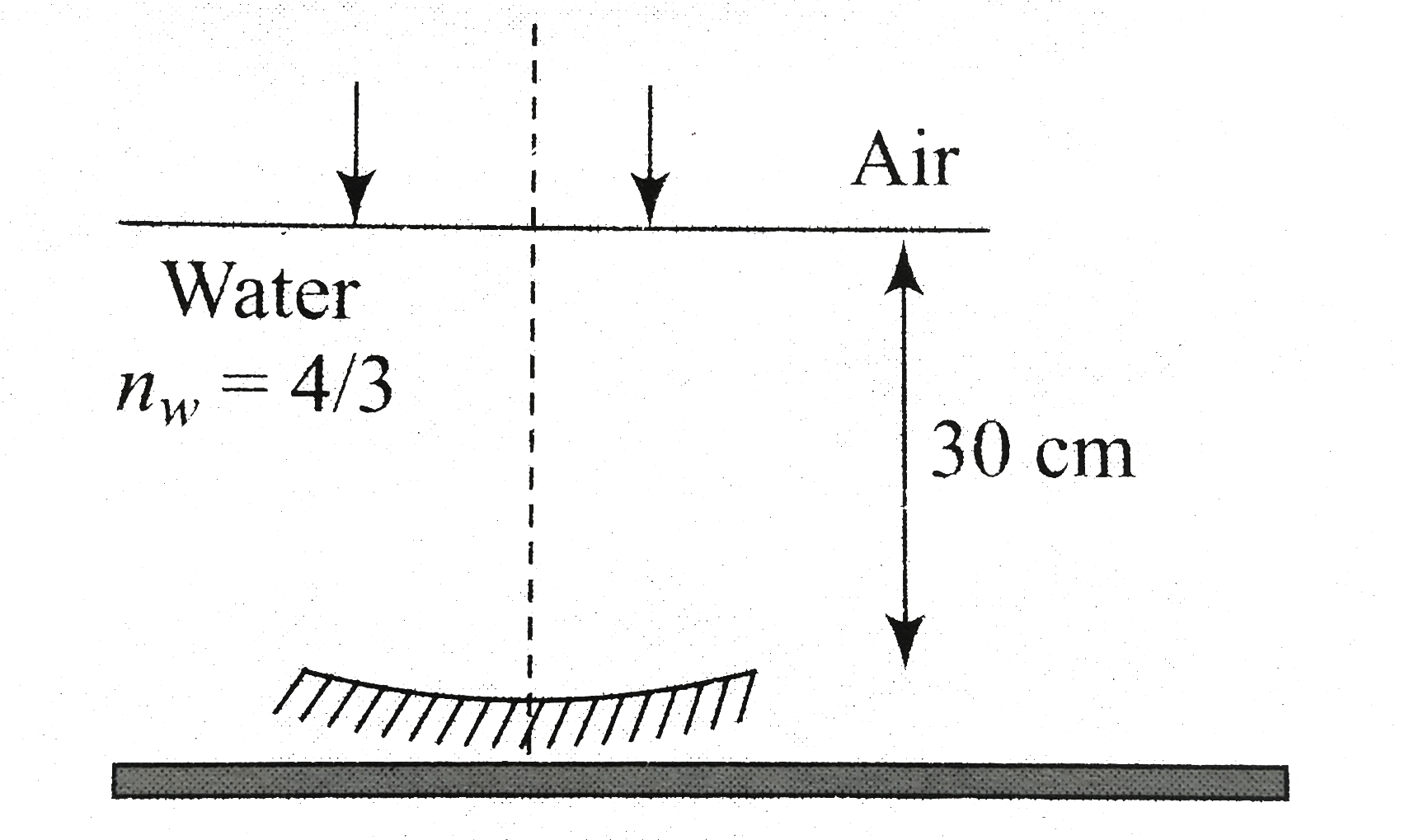
View SolutionA concave mirror of focal length 20cm is placed inside water with its shining surface upwards and principla axis vertical as shown in Fig. Rays are incident parallel to the principa, axis of concave mirror. Find the position of final image.
View SolutionAn object 4cm in size is placed at a distance of 25.0cm from a concave mirror of focal length 15.0 cm. Find the position, nature and height of the image.
View SolutionA concave mirror of focal length 15 cm is placed in water of refrective index μ=4/3. Water is filled upto 30 cm. An object of height 1 cm is paced at a distance of 20 cm in front of the mirror. If the size of image is n times size of object then n=
View SolutionA man uses a concave mirror for shaving. He keeps his face at a distance of 20cm form the mirror and gets an image which is 1.5 times entarged. Find the focal length of the mirror.
View SolutionAn object is placed at a distance of 20 cm from a concave mirror of focal lenght 10 cm. What is the image distance?
View SolutionA man uses a concave mirror for shaving. He keeps his face at a distance of 25 cm from the mirror and gets an image which is 1.4 times enlarged. Find the focal length of the mirror
View SolutionFor an object placed at a distance 20 cm from a concave mirror, the image is formed at the same position. What is the focal length of the mirror?
View SolutionA point object is moving on the principal axis of a concave mirror of focal length 24cm towards the mirror. When it is at a distance of 60cm from the mirror, its velocity is . 9sec/cm What is the velocity of the image at that instant
View Solution